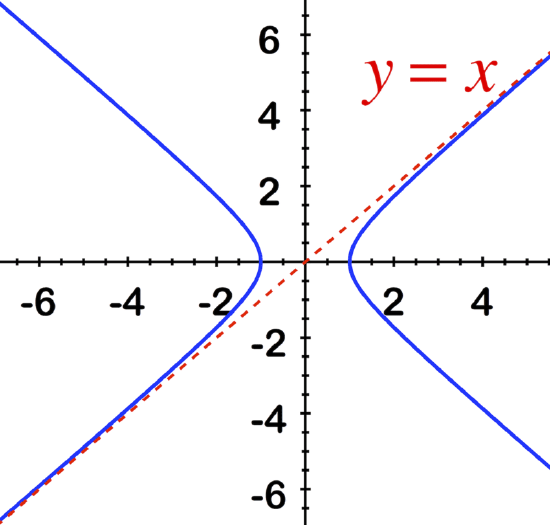
6.3.4: Hyperbola Equations and the Focal Property

Hyperbola Equations and the Focal Property

Adrian and Evan were discussing the math class they just completed. The class focused on hyperbolas (pun intended!), and reviewed the properties of hyperbolas.

Adrian thinks that hyperbolas are very similar to the parabolas that they studied last week, and believes that the shapes are really the same. Evan thinks that it does not matter what the parts of the hyperbola look like, since it is the complete shape that they are studying.

Who is correct?

---

Hyperbola Equations and the Focal Property

Compared to parabolas and ellipses, hyperbolas may seem... messy! Not only is it an infinite shape, but there are two pieces that aren’t even connected! Hyperbolas are conic sections though: when the plane slices through two parts of the cone, the two infinite “U”-shaped parts are together called a hyperbola.

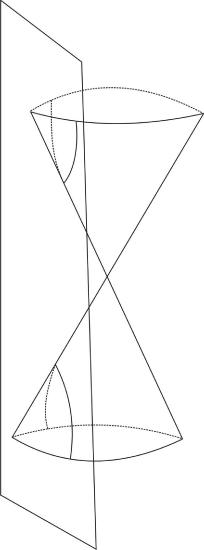
In this section we will see that this sprawling shape actually has some beautiful properties that make it as noble as its cousins.

The Focal Property

Even though this shape seems much harder to conceive of than an ellipse, the hyperbola has a defining focal property that is as simple as the ellipse’s. Remember, an ellipse has two foci and the shape can be defined as the set of points in a plane whose distances to these two foci have a fixed sum.

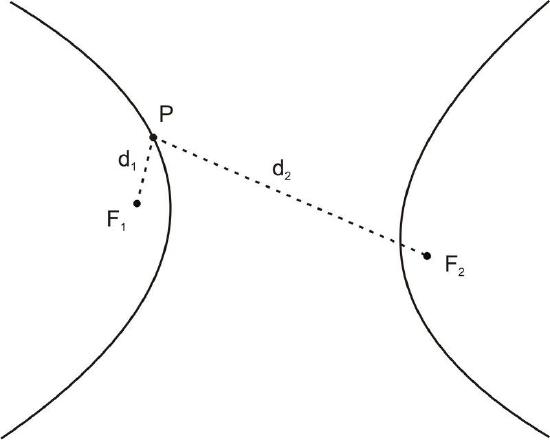
Hyperbolas also have two foci, and they can be defined as the set of points in a plane whose distances to these two points have the same difference. So in the picture below, for every point \(\ P\) on the hyperbola, \(\ \left|d_{2}-d_{1}\right|=C\) for some constant \(\ C\).

The general form for a hyperbola that opens upwards and downwards and whose foci lie on the y−axis is:

\(\ \frac{y^{2}}{a^{2}}-\frac{x^{2}}{b^{2}}=1\)

Switching x and y we have hyperbolas that open rightwards and leftwards and whose foci lie on the x−axis.

\(\ \frac{x^{2}}{a^{2}}-\frac{y^{2}}{b^{2}}=1\)

For a hyperbola that is centered around the point (h,k) we have the shifted equations:

\(\ \frac{(y-k)^{2}}{a^{2}}-\frac{(x-h)^{2}}{b^{2}}=1\)

for a hyperbola opening up and down, and

\(\ \frac{(x-h)^{2}}{a^{2}}-\frac{(y-k)^{2}}{b^{2}}=1\)

for a hyperbola opening left and right.

---

Examples

Example 1

Earlier, you were asked whether Evan or Adrian is correct.

Solution

Evan and Adrian are both correct in their own ways. Adrian is correct that a hyperbola is just two parabolas in opposite directions, which becomes clear when you consider that a parabola is created by slicing a single cone, and a hyperbola by slicing two identical cones at the same time. Evan is correct that although a hyperbola is constructed of two parabolas, it is the complete shape that they are studying, and many or most of the formulas and definitions that they will consider only apply to the complete shape.

Example 2

The hyperbola is infinite in size. In mathematics this is called unbounded, which means no circle, no matter how large, can enclose the shape. Explain why a focal property involving a difference results in an unbounded shape, while a focal property involving a sum results in a bounded shape.

Solution

In the case of an ellipse, we had two distances summing to a constant. Since the distances are both positive then there is a limit to the size of the numbers. In the case of hyperbolas, two very large positive numbers can have a much smaller difference, infinitely small in fact.

Example 3

Show that the following equation is a hyperbola. Graph it, and show its foci.

\(\ 144 x^{2}-576 x-25 y^{2}-150 y-3249=0\)

Solution

The positive leading coefficient for the \(\ x^{2}\) term and the negative leading coefficient for the \(\ y^{2}\) term indicate that this is a hyperbola that is horizontally oriented. Grouping and completing the square, we have:

\(\ \begin{aligned}
144\left(x^{2}-4 x\right)-25\left(y^{2}+6 y\right) &=3249 \\
144\left(x^{2}-4 x+4\right)-25\left(y^{2}+6 y+9\right) &=3249+576-225 \\
144(x-2)^{2}-25(y+3)^{2} &=3600 \\
\frac{(x-2)^{2}}{5^{2}}-\frac{(y+3)^{2}}{12^{2}} &=1
\end{aligned}\)

So our hyperbola is centered at \(\ (2,-3)\). Its vertices are 5 units to the right and left of \(\ (2,-3)\), or at the points \(\ (7,-3)\) and \(\ (-3,-3)\). It opens to the right and left from these vertices. It’s foci are \(\ c\) units to the left and right of \(\ (2,-3)\), where \(\ c=\sqrt{a^{2}+b^{2}}=\sqrt{5^{2}+12^{2}}=13\). So it’s foci are at \(\ (15,-3)\) and \(\ (-11,-3)\). Plotting a few points near \(\ (7,-3)\) and \(\ (-3,-3)\), the graph looks like:

Example 4

Graph the following hyperbola and mark its foci: \(\ 16 x^{2}+64 x-9 y^{2}+90 y-305=0\).

Solution

The positive leading coefficient for the term and the negative leading coefficient for the term indicate that this is a hyperbola that is horizontally oriented. Grouping and completing the square, we have:

\(\ 16\left(x^{2}+4 x+4\right)-9\left(y^{2}-10 y+25\right)-305=64-225\)

Factoring and combining like terms:

\(\ 16(x+2)^{2}-9(y-5)^{2}=144\)

Divide both sides by 144 and rewrite 9 and 16 as 3² and 4²:

\(\ \frac{(x+2)^{2}}{3^{2}}-\frac{(y-5)^{2}}{4^{2}}=1\)

Example 5

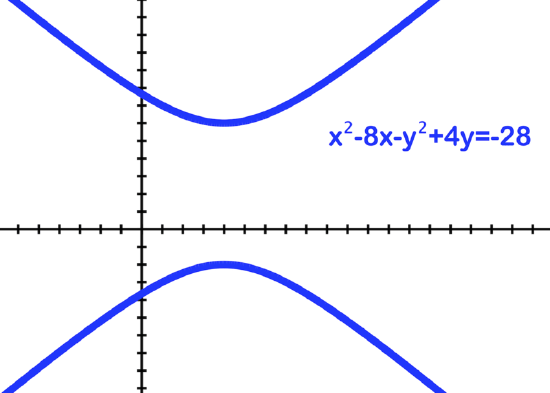
Graph the following hyperbola and mark its foci: \(\ x^{2}-8 x-y^{2}-4 y=-28\).

Solution

To graph \(\ x^{2}-8 x-y^{2}+4 y=-28\):

\(\ (x-4)^{2}-(y-2)^{2}=-16\)..... complete the square to factor

\(\ \frac{(y-2)^{2}}{16}-\frac{(x-4)^{2}}{16}=1\)..... rewrite in standard form

\(\ x-4=0 \rightarrow x=4\) and \(\ y-2=0 \rightarrow y=2\)..... therefore the center is \(\ (4, 2)\)

Mark 4 units left and right of \(\ (4, 2)\) and Mark 2 units above and below \(\ (4, 2)\), use those four points to define the sides of a box.

Connect the corners of the box to illustrate the asymptotes.

Since the "y" term is the positive one, the hyperbola opens up and down.

Graph the hyperbola, it should look like this:

Example 6

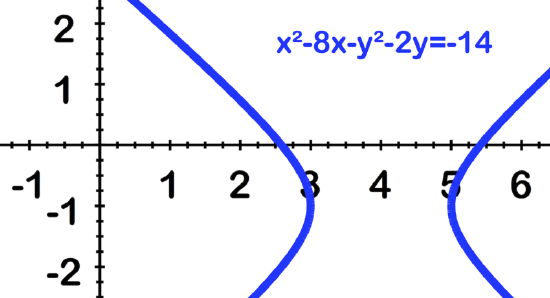
Graph the following hyperbola: \(\ x^{2}-8 x-y^{2}-2 y=-14\).

Solution

To graph \(\ x^{2}-8 x-y^{2}-2 y=-14\):

\(\ (x-4)^{2}-(y+1)^{2}=1\)..... complete the square to factor \(\ \frac{(x-4)^{2}}{1}-\frac{(y+1)^{2}}{1}=1\)..... rewrite in standard form

\(\ x-4=0 \rightarrow x=4\) and \(\ y+1=0 \rightarrow y=-1\)..... therefore the center is \(\ (4, -1)\)

Mark 4 units left and right of \(\ (4, -1)\) and Mark 1 unit above and below \(\ (4, -1)\), use those four points to define the sides of a box.

Connect the corners of the box to illustrate the asymptotes.

Since the "x" term is the positive one, the hyperbola opens left and right.

Graph the hyperbola, it should look like this:

Example 7

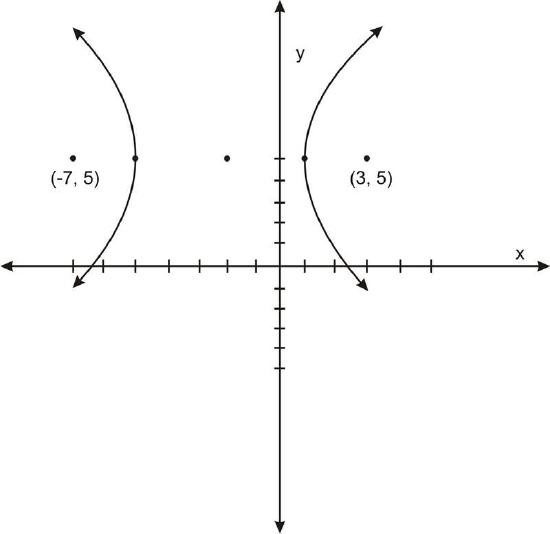
Find the equation for the following hyperbola:

Solution

\(\ \frac{(x-4)^{2}}{4}-\frac{(y+2)^{2}}{45}=1\)

---

Review

Sketch the hyperbolas.

1. \(\ \frac{(x+4)^{2}}{4}-\frac{(y-1)^{2}}{9}=1\)
2. \(\ \frac{(y+3)^{2}}{4}-\frac{(x-4)^{2}}{9}=1\)
3. \(\ \frac{(y+4)^{2}}{16}-\frac{(x-1)^{2}}{4}=1\)
4. \(\ (x-2)^{2}-4 y^{2}=16\)
5. \(\ \frac{y^{2}}{4}-\frac{(x-1)^{2}}{4}=1\)
6. \(\ \frac{(x-2)^{2}}{16}-\frac{(y+4)^{2}}{1}=1\)
7. \(\ \frac{(x+2)^{2}}{9}-\frac{(y+2)^{2}}{16}=1\)
8. \(\ \frac{(x+4)^{2}}{9}-\frac{(y-2)^{2}}{4}=1\)
9. Graph the hyperbola and mark its foci: \(\ 9 y^{2}+18 y-x^{2}+4 x-4=0\)
10. Graph the hyperbola and mark its foci: \(\ 25 x^{2}+150 x-4 y^{2}+24 y+89=0\)

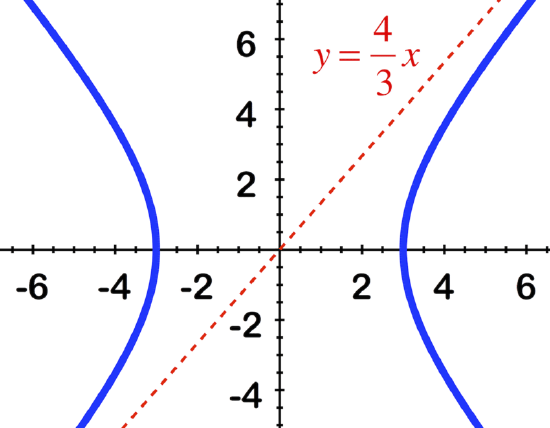
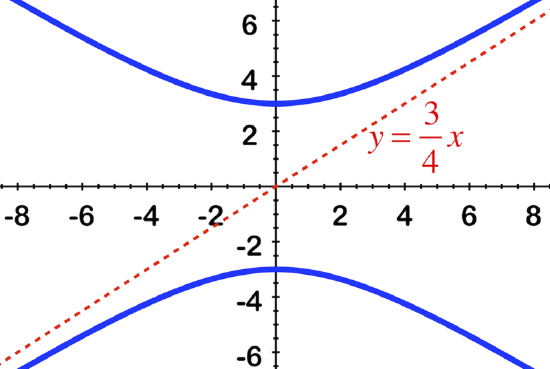
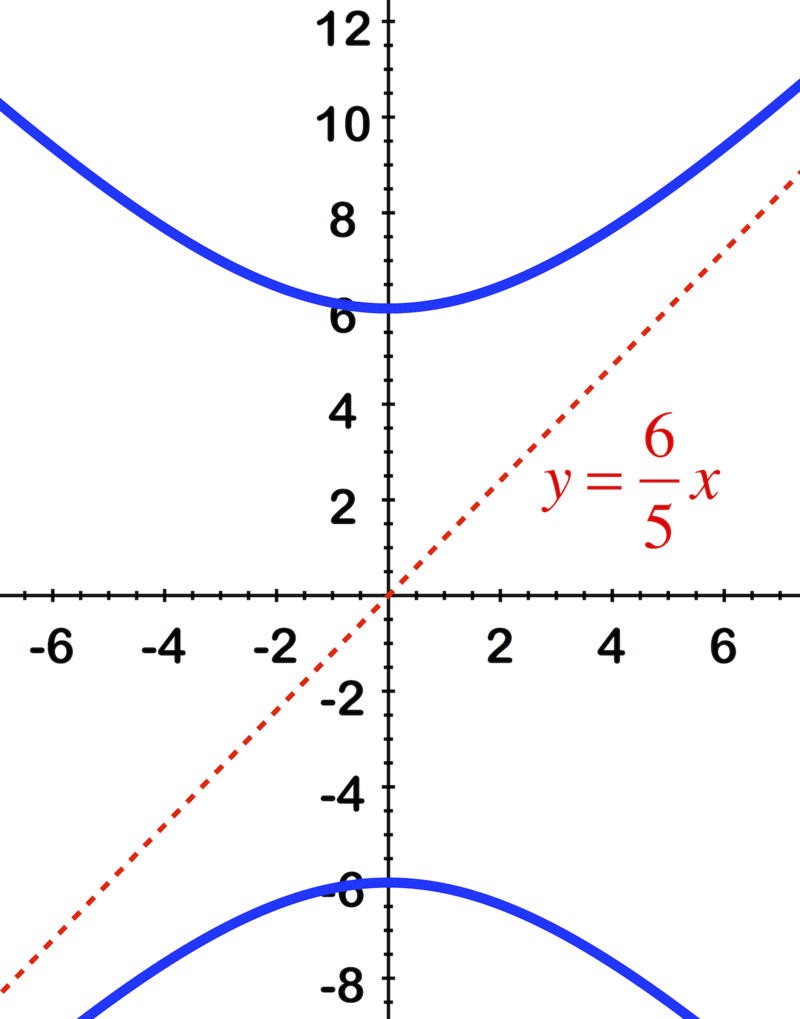
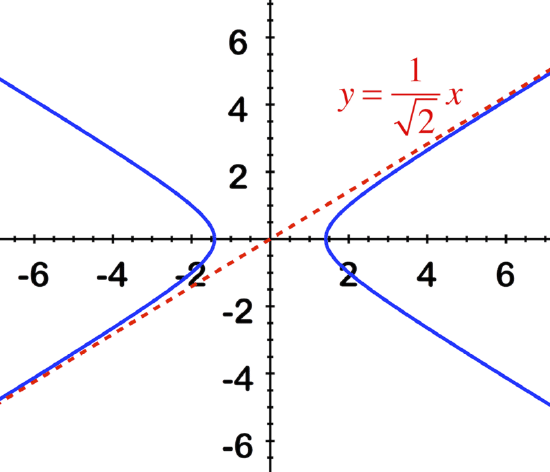
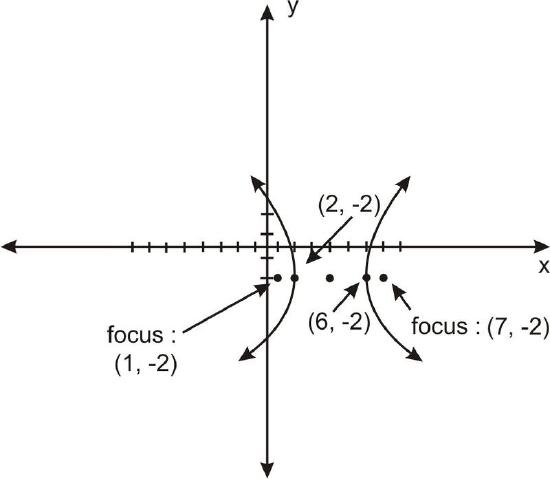
Identify the equation of the hyperbola using the image.

11. 
12. 
13. 
14. 
15. 

---

Review (Answers)

To see the Review answers, open this PDF file and look for section 6.6.

---

Vocabulary

Term	Definition
Asymptotes	An asymptote is a line on the graph of a function representing a value toward which the function may approach, but does not reach (with certain exceptions).
Conic	Conic sections are those curves that can be created by the intersection of a double cone and a plane. They include circles, ellipses, parabolas, and hyperbolas.
Ellipse	Ellipses are conic sections that look like elongated circles. An ellipse represents all locations in two dimensions that are the same distance from two specified points called foci.
Ellipses	Ellipses are conic sections that look like elongated circles. An ellipse represents all locations in two dimensions that are the same distance from two specified points called foci.
hyperbola	A hyperbola is a conic section formed when the cutting plane intersects both sides of the cone, resulting in two infinite “U”-shaped curves.
hyperbolas	A hyperbola is a conic section formed when the cutting plane intersects both sides of the cone, resulting in two infinite “U”-shaped curves.
Parabola	A parabola is the set of points that are equidistant from a fixed point on the interior of the curve, called the '''focus''', and a line on the exterior, called the '''directrix'''. The directrix is vertical or horizontal, depending on the orientation of the parabola.
perpendicular hyperbola	A perpendicular hyperbola has asymptotes that intersect at a 90∘ angle.
unbounded	To be unbounded means to be so large that no circle, no matter how large, can enclose the shape.