4.1.4: Angle-Side-Angle Triangles
- Page ID
- 4157
Law of sines given two angles and the included side.
You're eating lunch in the cafeteria one afternoon while working on your math homework. Lately you seem to notice the triangular shapes in everything. At home, at school, with your friends. It seems like triangles are everywhere. And you find yourself trying to apply what you are learning in math class to all of the triangles around you. And today is no exception. As you start to take a bite of your chip, you suddenly recognize that familiar shape - the triangle.
You estimate the length of one of the sides of the chip to be 3 cm. You also can tell that the angle adjacent to the 3 cm side is \(50^{\circ} \) and the angle adjacent on the other side of the 3 cm edge is \(60^{\circ} \). Can you find the lengths of the other two sides using techniques from your math class?
ASA Triangles
The Law of Sines states: \(\dfrac{\sin A}{a}=\dfrac{\sin B}{b}\). This is a ratio between the sine of an angle in a triangle and the length of the side opposite that angle to the sine of a different angle in that triangle and the length of the side opposing that second angle.
One case where we use the Law of Sines is when we know two angles in a triangle and the included side (ASA). For instance, in \(\Delta TRI\):

\(\angle T\), \(\angle R\), and \(i\) are known
\(\angle T\), \(\angle I\), and \(r\) are known
\(\angle R\), \(\angle I\), and \(t\) are known
In this case, the Law of Sines allows us to find either of the non-included sides.
Using the Law of Sines
1. In the triangle above, \(\Delta TRI\), \(\angle T=83^{\circ} \), \(\angle R=24^{\circ} \), and \(i=18.5\). Find the measure of \(t\).
Since we know two angles and the included side, we can find either of the non-included sides using the Law of Sines. Since we already know two of the angles in the triangle, we can find the third angle using the fact that the sum of all of the angles in a triangle must equal \(180^{\circ} \).
\(\begin{aligned} \angle I&=180−(83+24) \\ \angle I&=180−107 \\ \angle I&=73^{\circ} \end{aligned}\)
Now that we know \(\angle I=73^{\circ} \), we can use the Law of Sines to find \(t\).
\(\begin{aligned} \dfrac{\sin 73}{18.5}&=\dfrac{\sin 83}{t} \\ t(\sin 73)&=18.5(\sin 83) \\ t&=\dfrac{18.5(\sin 83)}{\sin 73} \\ t&\approx 19.2 \end{aligned}\)
Notice how we wait until the last step to input the values into the calculator. This is so our answer is as accurate as possible.
2. In order to avoid a large and dangerous snowstorm on a flight from Chicago to Buffalo, pilot John starts out \(27^{\circ} \) off of the normal flight path. After flying 412 miles in this direction, he turns the plane toward Buffalo. The angle formed by the first flight course and the second flight course is \(88^{\circ} \). For the pilot, two issues are pressing:
- What is the total distance of the modified flight path?
- How much further did he travel than if he had stayed on course?
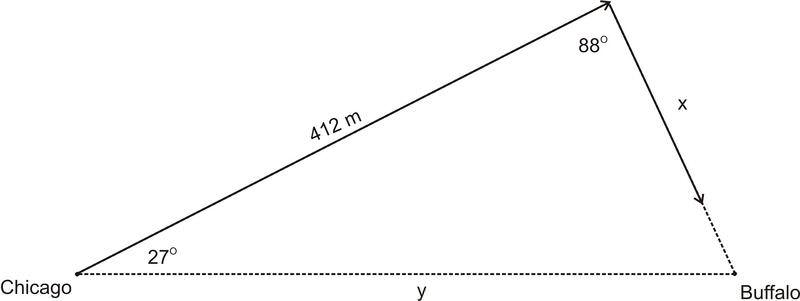
In order to find the total distance of the modified flight path, we need to know side \(x\). To find side \(x\), we will need to use the Law of Sines. Since we know two angles and the included side, this is an ASA case. Remember that in the ASA case, we need to first find the third angle in the triangle.
\(\begin{aligned} \text{Missing Angle}&=180−(27+88)=65^{\circ} && \text{The sum of angles in a triangle is 180} \\ \dfrac{\sin 65}{412}&=\dfrac{\sin 27}{x} && \text{Law of Sines} \\ x(\sin 65)&=412(\sin 27) && \text{Cross multiply}\\ x&=\dfrac{412(\sin 27)}{\sin 65} && \text{ Divide by} \sin 65 \\ x &\approx 206.4 \text{ miles} \end{aligned}\)
The total distance of the modified flight path is \(412+206.4=618.4 \text{ miles}\).
To find how much farther John had to travel, we need to know the distance of the original flight path, \(y\). We can use the Law of Sines again to find \(y\).
\(\begin{aligned}\dfrac{\sin 65}{412}&=\dfrac{\sin 88}{y} && \text{Law of Sines}\\ y(\sin 65)&=412(\sin 88) && \text{Cross multiply}\\ y&=\dfrac{412(\sin 88)}{\sin 65} && \text{Divide by }\sin 65\\ y &\approx 454.3 \text{ miles} \end{aligned}\)
John had to travel \(618.4−454.3=164.1 \text{ miles}\) farther.
2. In the triangle shown here:
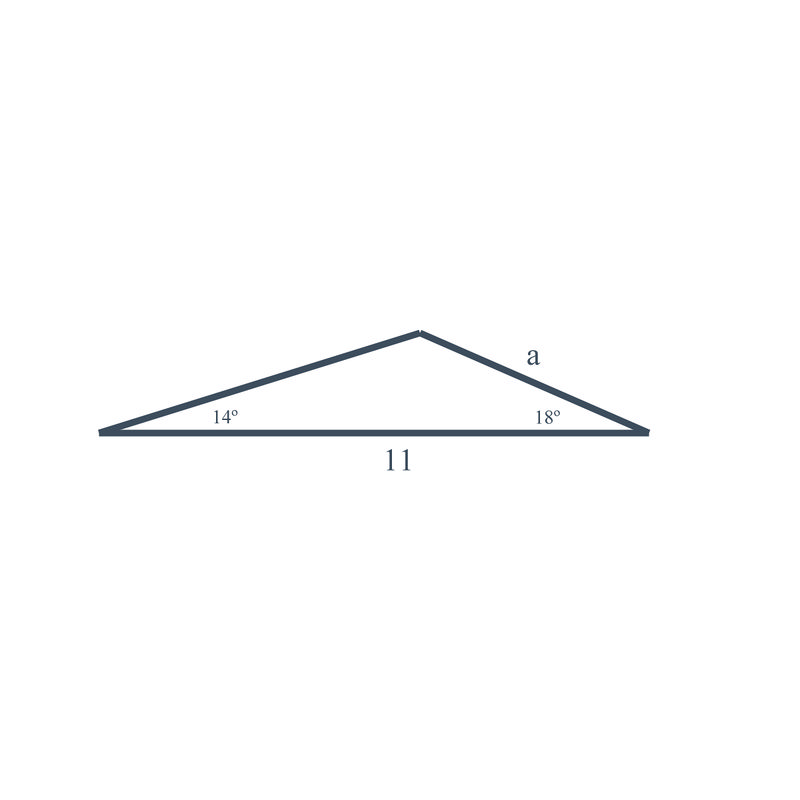
The sides given are \(\angle A=14^{\circ} \), \(\angle B=18^{\circ} \), and \(c = 11\). Find the length of side "a".
Since we know two angles and the included side, we can find either of the non-included sides using the Law of Sines. Since we already know two of the angles in the triangle, we can find the third angle using the fact that the sum of all of the angles in a triangle must equal \(180^{\circ} \).
\(\begin{aligned} \angle C &=180−(18+14)\\ \angle C &=180−32\\ \angle C&=148^{\circ} \end{aligned}\)
Use the Law of Sines to find the length of side "a":
\(\begin{aligned} \dfrac{\sin 14}{a}&=\dfrac{\sin 148}{11} \\ 11(\sin 14)&=a(\sin 148) \\ a&=\dfrac{11(\sin 14)}{\sin 148} \\ a &\approx 5.02 \end{aligned}\)
Earlier, you were asked to find the other two lengths of the triangle.
Solution
You can use the Law of Sines to find the length of either of the other 2 sides. However, first it is good to note that since the sum of the interior angles of a triangle must equal \(180^{\circ} \), the third angle in the triangle must measure \(180^{\circ} −50^{\circ} −60^{\circ} =70^{\circ} \).
Now to set up the ratios:
\(\begin{aligned} \dfrac{\sin 60}{a}&=\dfrac{\sin 70}{3} \\ 3(\sin 60)&=a(\sin 70) \\ a&=\dfrac{3(\sin 60)}{\sin 70} \\ a&\approx 2.7647 \end{aligned}\)
The length of one of the other 2 sides is approximately 2.7647 centimeters.
To find the length of the last side:
\(\begin{aligned} \dfrac{\sin 50}{b}&=\dfrac{\sin 70}{3} \\ 3(\sin 50)&=b(\sin 70) \\ b&=\dfrac{3(\sin 50)}{\sin 70} \\ b &\approx 2.46 \end{aligned}\)
The length of the other one of the 2 unknown sides is approximately 2.46 centimeters.
Find side "a" in the triangle below using the following information: \(b=16,\; A=11.7^{\circ} ,\; C=23.8^{\circ}\)
Solution
\(\dfrac{\sin 11.7^{\circ} }{a}=\dfrac{\sin 144.5^{\circ} }{16},\; a=5.6\)
Find side "a" in the triangle below using the following information: \(k=6.3,\; J=16.2^{\circ} ,\; L=40.3^{\circ}\)
Solution
\(\dfrac{\sin 40.3^{\circ} }{l}=\dfrac{\sin 123.5^{\circ} }{6.3} ,\; l=4.9\)
Even though ASA and AAS triangles represent two different cases of the Law of Sines, what do they both have in common?
Solution
Student answers will vary but they should notice that in both cases you know or can find an angle and the side across from it.
Review
In \(\Delta ABC\), \(m\angle A=40^{\circ} \), \(m\angle B=67^{\circ} \), and \(c = 6\).
- Find \(m\angle C\).
- Find the length of \(a\).
- Find the length of \(b\).
In \(\Delta DEF\), \(m\angle D=36^{\circ} \), \(m\angle E=101^{\circ} \), and \(f = 11\).
- Find \(m\angle F\).
- Find the length of \(d\).
- Find the length of \(e\).
In \(\Delta BIG\), \(m\angle B=56^{\circ} \), \(m\angle I=71^{\circ} \), and \(g = 23\).
- Find \(m\angle G\).
- Find the length of \(b\).
- Find the length of \(i\).
In \(\Delta APL\), \(m\angle A=79^{\circ} \), \(m\angle P=40^{\circ} \), and \(l = 15\).
- Find \(m\angle L\).
- Find the length of \(a\).
- Find the length of \(p\).
In \(\Delta SAU\), \(m\angle S=5^{\circ} \), \(m\angle A=99^{\circ} \), and \(u = 21\).
- Find \(m\angle U\).
- Find the length of \(s\).
- Find the length of \(a\).
Review (Answers)
To see the Review answers, open this PDF file and look for section 5.8.
Vocabulary
| Term | Definition |
|---|---|
| Angle Side Angle Triangle | The term 'angle-side-angle triangle' refers to a triangle with known measures of two angles and the length of the side between them. |
Additional Resources
Video: Law of Sines - Solving ASA Triangle
Practice: Angle-Side-Angle Triangles

