4.2.1: Area Formula for Non-Right Triangles
- Page ID
- 4165
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Area equals half the product of two sides and the sine of the included angle.
Alternate Formula for the Area of a Triangle
You are studying the Gulf of Mexico in your Geography class. Your Instructor brings up the idea of the Bermuda Triangle. This is a place where, according to some, many planes get lost. Here is an illustration of it:
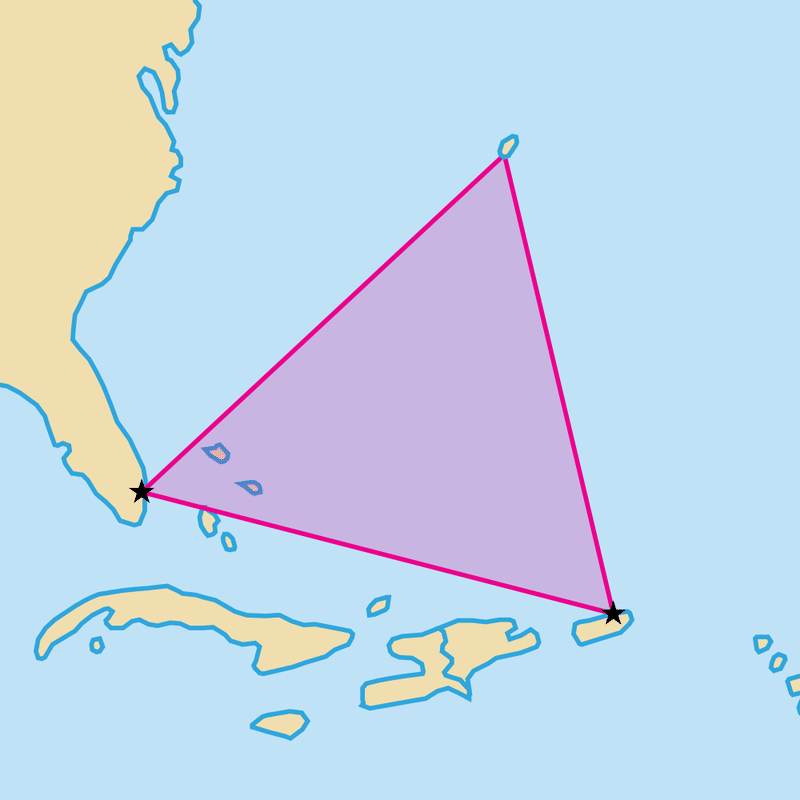
The first thing this makes you think of is your math class, since that class is your favorite. You would like to know just how big the Bermuda Triangle is. Unfortunately, the Bermuda Triangle isn't a right triangle. However, you do know that the lengths of one of the sides is 950 miles, the other side is 975 miles, and the angle between them is \(60^{\circ} \).
Is there any way to use this information to help you find out just how big the Bermuda Triangle is?
Area of a Triangle
In Geometry, you learned that the area of a triangle is \(A=\dfrac{1}{2} bh\), where \(b\) is the base and h is the height, or altitude.
Now that you know the trig ratios, this formula can be changed around, using sine.
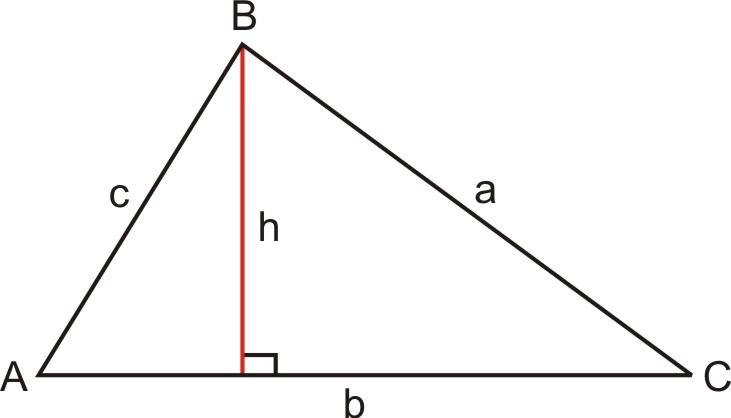
Looking at the triangle above, you can use sine to determine \(h\), \(\sin C=\dfrac{h}{a}\). So, solving this equation for \(h\), we have \(a \sin C=h\). Substituting this for \(h\), we now have a new formula for area.
\(A=\dfrac{1}{2} ab \sin C\)
What this means is you do not need the height to find the area anymore. All you now need is two sides and the angle between the two sides, called the included angle.
Finding Area
1.
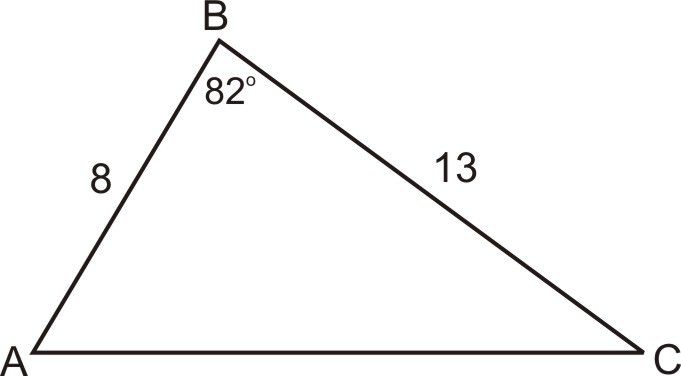
Using the formula, \(A=\dfrac{1}{2} ab \sin C\), we have
\(\begin{aligned} A&=\dfrac{1}{2} \cdot 8\cdot 13\cdot \sin 82^{\circ} \\ &=4\cdot 13\cdot 0.990\\ &=51.494 \end{aligned}\)
2.
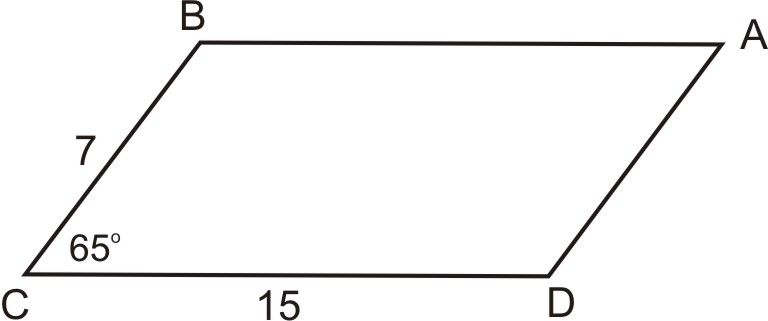
Recall that a parallelogram can be split into two triangles. So the formula for a parallelogram, using the new formula, would be: \(A=2\cdot \dfrac{1}{2} ab \sin C\) or \(A=ab \sin C\).
\(\begin{aligned} A&=7\cdot 15\cdot \sin 65^{\circ} \\&=95.162 \end{aligned} \)
3.
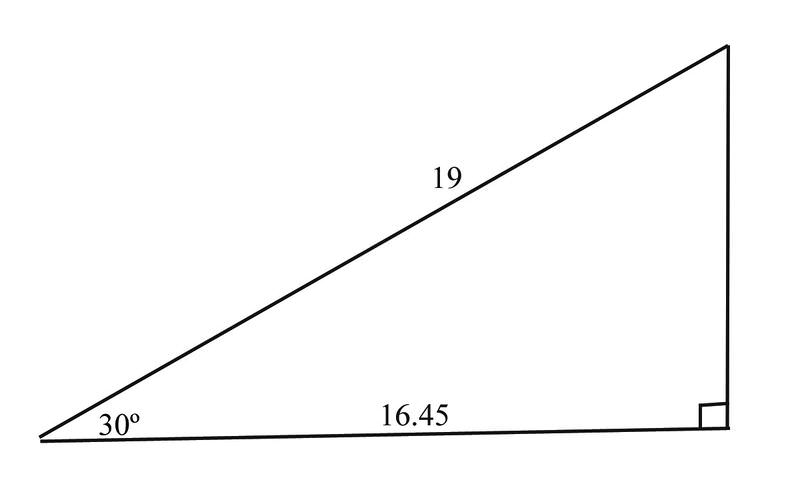
Using the formula, \(A=\dfrac{1}{2} ab \sin C\), we have
\(\begin{aligned} A&=\dfrac{1}{2} \cdot 16.45\cdot 19\cdot \sin 30^{\circ} \\ &=8.225\cdot 19\cdot 0.5 \\&=78.14\end{aligned}\)
Earlier, you were asked how big the Bermuda Triangle is.
Solution
Now that you know the equation for the area of a triangle in terms of two of the sides and the included angle, we can use that to solve for the area of the Bermuda Triangle:
\(\begin{aligned} A&=\dfrac{1}{2} ab \sin\theta \\ A&=\dfrac{1}{2} (950)(975) \sin 60^{\circ} \\ A&=\dfrac{1}{2} (950)(975)(.866) \\ A&=401066.25\end{aligned}\)
The area of the triangle is approximately 401,006 square miles.
Find the area of the triangle.
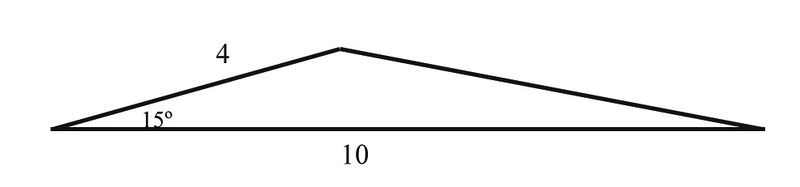
Solution
Using the formula, \(A=\dfrac{1}{2} ab \sin C\), we have
\(\begin{aligned} A&=\dfrac{1}{2} \cdot 4\cdot 10\cdot \sin 15^{\circ} \\ &=2\cdot 10\cdot 0.2589 \\ &=5.178 \end{aligned} \)
Find the area of the triangle.
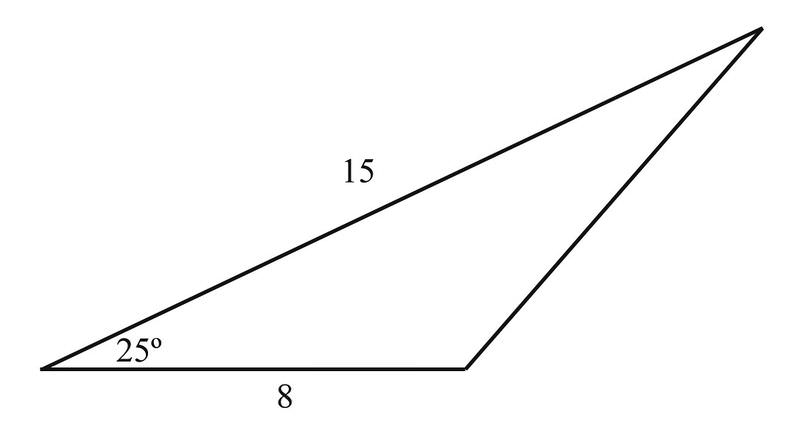
Solution
Using the formula, \(A=\dfrac{1}{2} ab \sin C\), we have
\(\begin{aligned} A&=\dfrac{1}{2}\cdot 8\cdot 15\cdot \sin 25^{\circ} \\ &=4\cdot 15\cdot 0.4226\\ &=25.356 \end{aligned}\)
Find the area of the triangle.
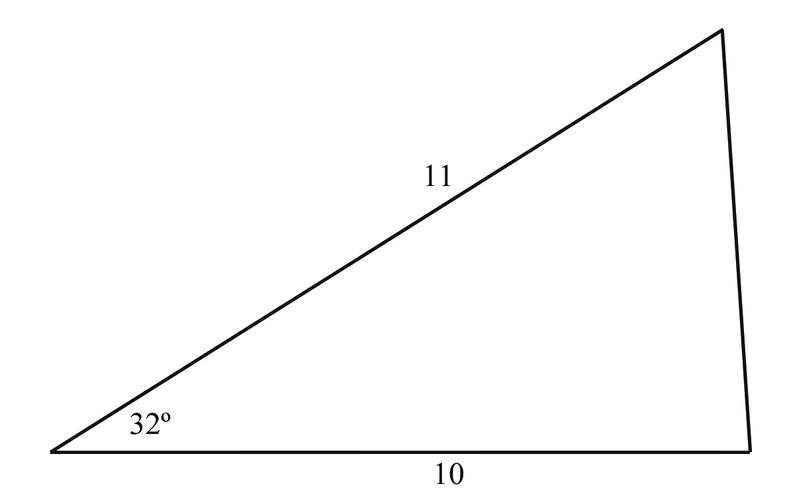
Solution
Using the formula, \(A=\dfrac{1}{2} ab \sin C\), we have
\(\begin{aligned} A&=\dfrac{1}{2} \cdot 10\cdot 11\cdot \sin 32^{\circ} \\&=5\cdot 11\cdot 0.53 \\ &=29.15 \end{aligned}\)
Review
Use the following picture for questions 1 and 2.
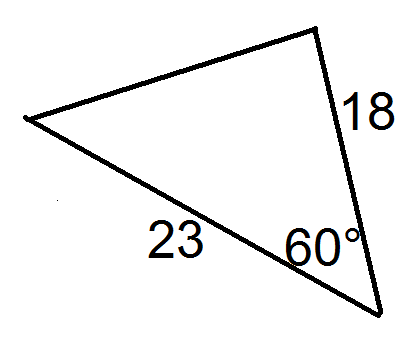
- Find the values of \(a\), \(b\), and \(C\) needed for the formula to find the area of the triangle.
- Now find the area of the triangle.
Use the following picture for questions 3 and 4.
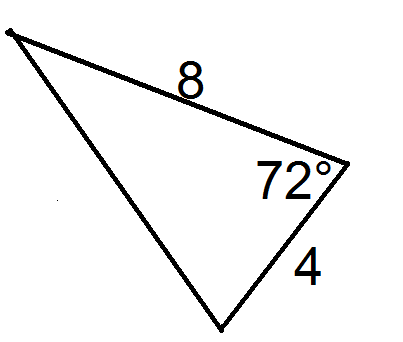
- Find the values of \(a\), \(b\), and \(C\) needed for the formula to find the area of the triangle.
- Now find the area of the triangle.
Find the area of each triangle below.
-
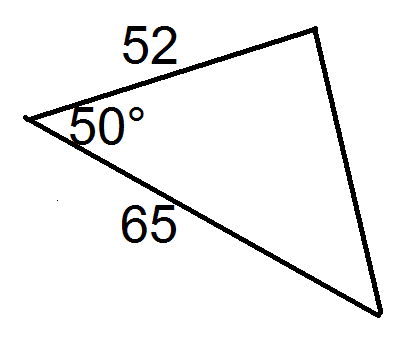
Figure \(\PageIndex{11}\) -
Figure \(\PageIndex{12}\) -
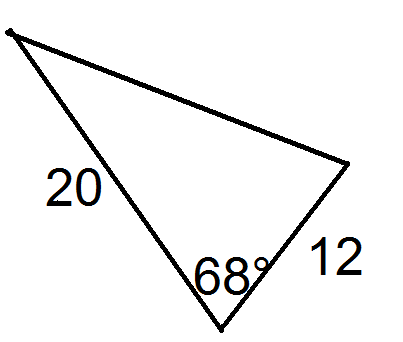
Figure \(\PageIndex{13}\) -
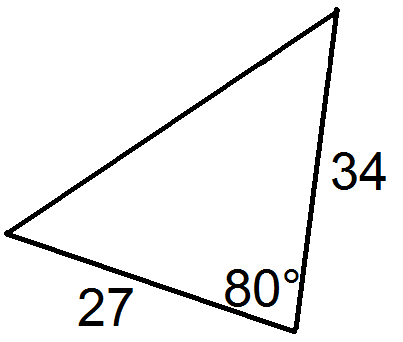
Figure \(\PageIndex{14}\) -
Figure \(\PageIndex{15}\) -
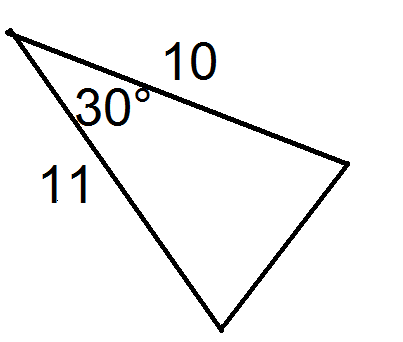
Figure \(\PageIndex{16}\) -
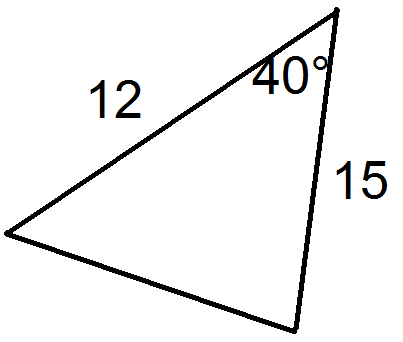
Figure \(\PageIndex{17}\)
Find the area of each parallelogram.
-
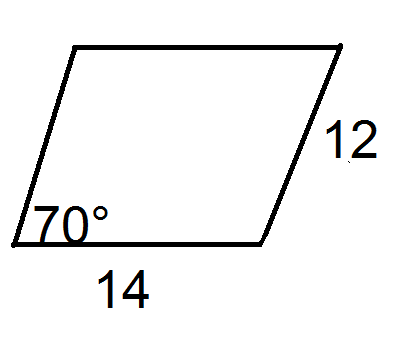
Figure \(\PageIndex{18}\) -
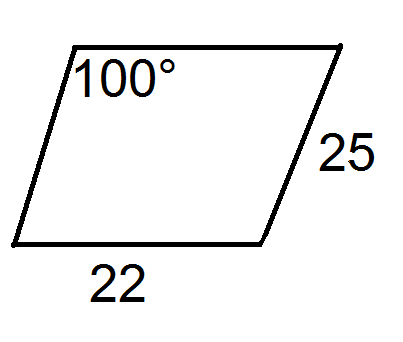
Figure \(\PageIndex{19}\) - Describe another way you could have found the area of the parallelogram in the previous problem.
- When you first learned about sine, you learned how it worked for right triangles. Explain why this method for calculating area uses sine, but works for non-right triangles.
Review (Answers)
To see the Review answers, open this PDF file and look for section 1.12.
Vocabulary
| Term | Definition |
|---|---|
| Included Angle | The included angle in a triangle is the angle between two known sides. |
| sine | The sine of an angle in a right triangle is a value found by dividing the length of the side opposite the given angle by the length of the hypotenuse. |
Additional Resources
Video: Example: Determine the Area of a Triangle Using the Sine Function
Practice: Area Formula for Non-Right Triangles
Real World: An Unusual Area?