5.2.2: Rectangular to Polar Conversions

Last updated
Save as PDF

Page ID: 4180

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Convert from cartesian to polar coordinates

Trig Riddle: I am the point \((1,−3)\). What are my polar coordinates?

Trigonometric Ratios of Points

Any point in the coordinate plane can be represented by its angle of rotation and radius, or distance from the origin. The point is said to lie on the terminal side of the angle. We can find the measure of the reference angle using right triangle trigonometry. When the point is identified in this manner we call the coordinates Polar coordinates. They are written as \((r,\theta )\), where \(r\) is the radius and \(\theta \) is the angle of rotation. The angle of rotation can be given in degrees or radians.

Let's find the angle of rotation (in degrees) and radius (distance from the origin) of the point \((−3,6)\).

First, make a sketch, plot the point and drop a perpendicular to the x-axis to make a right triangle.

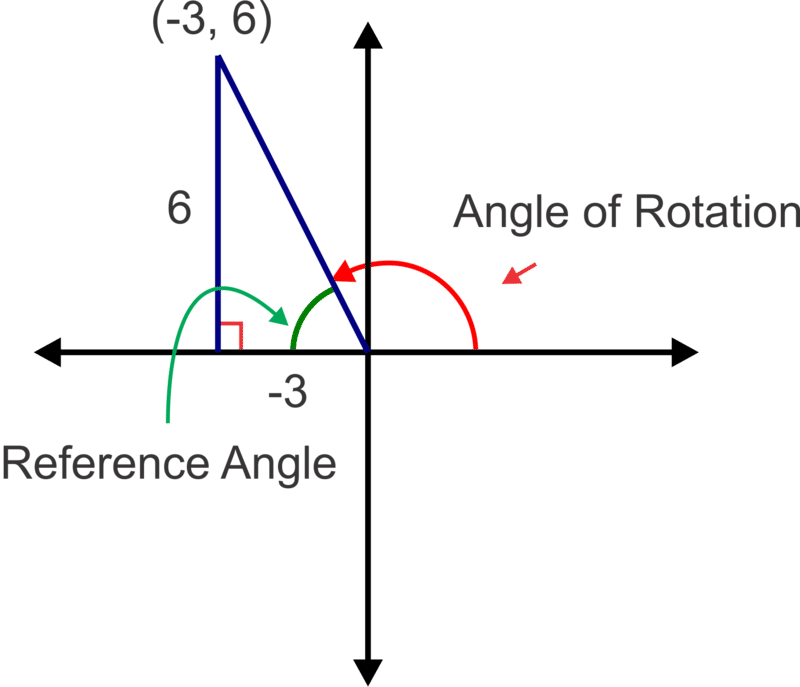
f-d_a3e37b4d8061317b75544bca369aee46808633312c10174c1e08fba2+IMAGE_TINY+IMAGE_TINY.png — Figure \(\PageIndex{1}\)

From the sketch, we can see that \(\tan^{-1}(−63)=63.4^{\circ}\) is the reference angle so the angle of rotation is \(180^{\circ}−63.4^{\circ}=116.6^{\circ}\).

The radius or distance from the origin is the hypotenuse of the right triangle.

\(\begin{aligned} r^2 &=(−3)2+(6)2\\ r^2 &=45\\ r&=\sqrt{45}=3 \sqrt{5}\end{aligned}\)

Using this information, we can write the point \((−3,6)\) in Polar coordinate form as \((3\sqrt{5}, 116.6^{\circ})\)

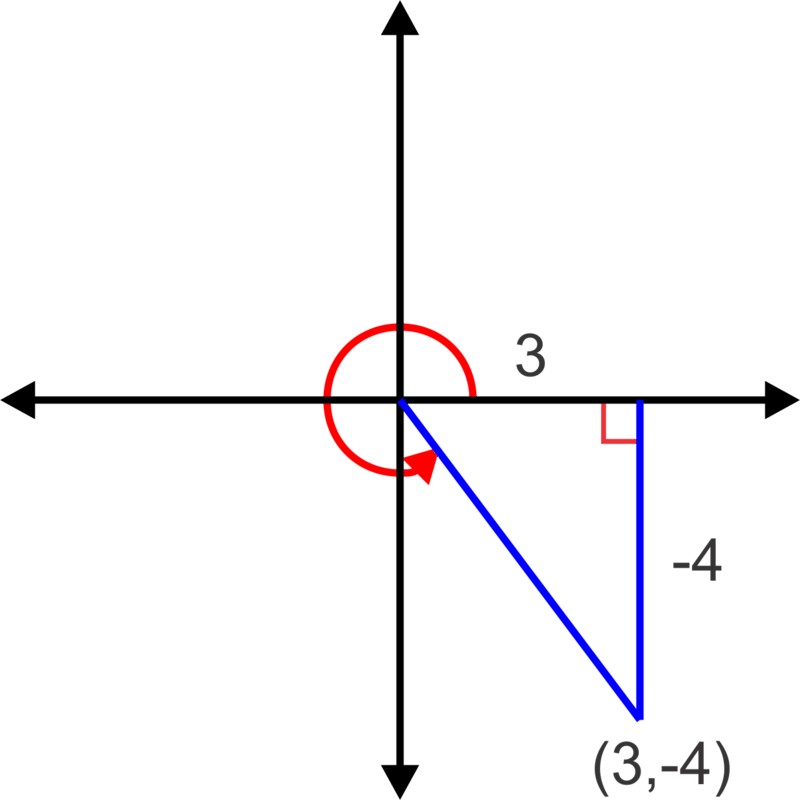
Now, let's write the Cartesian coordinates, \((3,−4)\), in Polar form and give the angle in degrees.

Again, start with a sketch.

We can find the reference angle again using tangent: \(\tan^{-1}\left(\dfrac{−4}{3}\right)=−53.1^{\circ}\). So the angle of rotation is \(360^{\circ}−53.1^{\circ}=306.9^{\circ}\)

Now find the radius:

\(\begin{aligned} r^2 &=3^2+(−4)^2\\ r^2 &=25 \\ r&=\sqrt{25}=5 \end{aligned}\)

The Polar coordinates are thus \((5, 306.9^{\circ})\)

f-d_cc7272cbe2d522cf016ca26e9826ea7f41da801d5bfd024e24073787+IMAGE_TINY+IMAGE_TINY.png — Figure \(\PageIndex{2}\)

Note: You may have noticed that there is a pattern that gives us a short cut for finding the Polar coordinates for any Cartesian coordinates, \((x,y)\):

The reference angle can be found using, \(\theta =\tan^{-1}\left(\dfrac{y}{x}\right)\) and then the angle of rotation can be found by placing the reference angle in the appropriate quadrant and giving a positive angle of rotation from the positive x – axis (\(0^{\circ}\leq \theta <360^{\circ}\) or \(0\leq \theta <2\pi\) ). The radius is always \(r=\sqrt{x^2+y^2}\) and should be given in reduced radical form.

Finally, given the point (−9,−5) on the terminal side of an angle, let's find the Polar coordinates (in radians) of the point and the six trigonometric ratios for the angle.

Make sure your calculator is in radian mode. Using the shortcut, we can find the Polar coordinates:

\(\tan^{-1}\left(\dfrac{5}{9}\right)=0.51\). Since x and y are both negative, the point lies in the third quadrant which makes the angle of rotation \(\pi +0.51=3.65\). The radius will be \(r=\sqrt{9^2+5^2}=\sqrt{106}\). The Polar coordinates are \((\sqrt{106}, 3.65)\). As for the six trigonometric ratios, a diagram will help us:

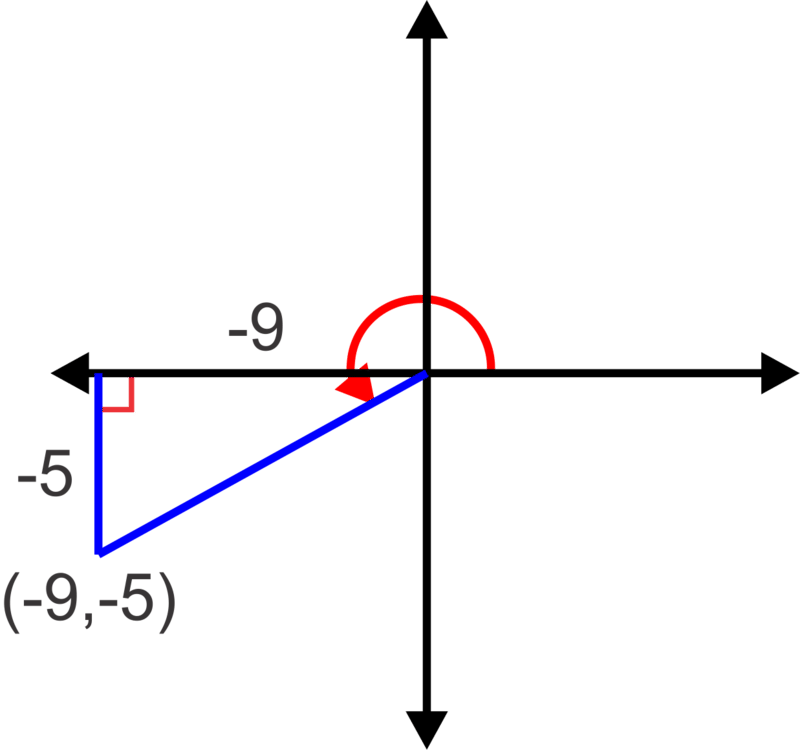
f-d_d8c3e19522e8c0b61f03c091797d20dd42cbff1b6ca032a9f59a8346+IMAGE_TINY+IMAGE_TINY.png — Figure \(\PageIndex{3}\)

We already know that \(\tan 3.65=\dfrac{5}{9}\), so \(\cot 3.65=\dfrac{9}{5}\).

Now we can use the hypotenuse, \(\sqrt{106}\) to find the other ratios:

\(\sin 3.65=\dfrac{−5}{\sqrt{106}}=−\dfrac{5 \sqrt{106}}{106}\) and \(\csc 3.65=\dfrac{\sqrt{−106}}{5}\).

\(\cos 3.65=\dfrac{−9}{\sqrt{106}}=−\dfrac{9\sqrt{106}}{106}\) and \(\sec 3.65=−\dfrac{\sqrt{106}}{9}\)

Example \(\PageIndex{1}\)

Earlier, you were asked to find the polar coordinates of the point \((1,−3)\).

Solution

First, make a sketch, plot the point and drop a perpendicular to the x-axis to make a right triangle.

From the sketch, we can see that \(\tan^{-1}(−31)=71.6^{\circ}\) is the reference angle. The point \((1,−3)\) is in the second quadrant, so the angle of rotation is \(180^{\circ}−71.6^{\circ}=108.4^{\circ}\).

The radius or distance from the origin is the hypotenuse of the right triangle.

\(\begin{aligned}
r^{2} &=(1)^{2}+(-3)^{2} \\
r^{2} &=10 \\
r &=\sqrt{10}
\end{aligned}\)

Therefore, my polar coordinates are \((\sqrt{10},108.4^{\circ})\).

Example \(\PageIndex{2}\)

Find the angle of rotation (in degrees) and radius (distance from the origin) of the point \((7,24)\).

Solution

\(r=\sqrt{7^2+24^2}=25\), \(\theta =\tan^{-1}\left(\dfrac{24}{7}\right) \approx 73.7^{\circ}\)

Example \(\PageIndex{3}\)

Write the Cartesian coordinates, (−8,−15), in Polar form(in radians) and find the six trigonometric ratios for the angle.

Solution

\(r=\sqrt{(−8)^2+(−15)^2}=17\) and \(\theta =\tan^{−1}\left(\dfrac{−15}{−8}\right)\approx 1.08\) so the polar coordinates are \((17,1.08)\).

The six trigonometric ratios are:

\(\begin{array}{ll}
\sin 1.08=-\dfrac{15}{17} & \csc 1.08=-\dfrac{17}{15} \\
\cos 1.08=-\dfrac{8}{17} & \sec 1.08=-\dfrac{8}{15} \\
\tan 1.08=\dfrac{15}{8} & \cot 1.08=-\dfrac{8}{15}
\end{array}\)

Example \(\PageIndex{4}\)

Given the point \((12,−4)\) on the terminal side of an angle, find the Polar coordinates (in degrees) of the point and the six trigonometric ratios for the angle.

Solution

\(r=\sqrt{12^2+(−4)^2} =4\sqrt{10}\) and \(\theta =\tan^{−1}\left(\dfrac{−4}{12}\right)\approx 341.6^{\circ}\) so the polar coordinates are \((4\sqrt{10}, 341.6^{\circ})\).

The six trigonometric ratios are:

\(\begin{aligned}
\sin 341.6^{\circ} &=-\dfrac{\sqrt{10}}{10} & \csc 341.6^{\circ} &=-\sqrt{10} \\
\cos 341.6^{\circ} &=\dfrac{3 \sqrt{10}}{10} & \csc 341.6^{\circ} &=\dfrac{\sqrt{10}}{3} \\
\tan 341.6^{\circ} &=-\dfrac{1}{3} & \tan 341.6^{\circ} &=-3
\end{aligned}\)

Review

Angle measures should be rounded to the dearest degree or hundredth of a radian or given exactly if possible. All values of r should be given in reduced radical form.

Write the following Cartesian coordinate pairs in Polar form. Use degrees for problems 1 and 2 and radians for problems 3-5.

\((16,−30)\)
\((5,5)\)
\((−5,−12)\)
\((−9,40)\)
\((−4,8)\)

Given the points on the terminal side of an angle, find the Polar coordinates (in degrees) of the point and the six trigonometric ratios for the angles.

\((−6,8)\)
\((0,−15)\)
\((10,−8)\)
\((4\sqrt{3},4)\)
\((−6,6)\)

Given the points on the terminal side of an angle, find the Polar coordinates (in radians) of the point and the six trigonometric ratios for the angles.