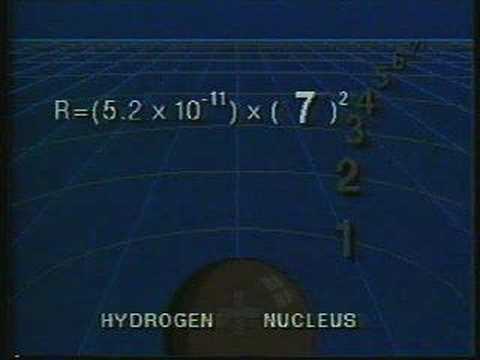20.3: Bohr's Atomic Model

Figure 20.3.1

The gold foil experiment was conducted under the supervision of Ernest Rutherford at the University of Manchester in 1909 by Hans Geiger and Ernest Marsden. The popular theory of atomic structure at the time of Rutherford’s experiment was the “plum pudding model.” This theory held that the negatively charged electrons in an atom were floating in a sea of positive charge (the electrons playing the role of plums in a bowl of plum pudding). Rutherford’s gold foil experiment demonstrated that almost all of the mass of an atom is in a tiny volume in the center of the atom which Rutherford called the nucleus. This positively charged mass was responsible for deflecting alpha particles propelled through the gold foil. Rutherford’s “nuclear model” of the atom replaced the plum pudding model in 1911.

The Bohr Model of the Atom

Early History of the Atom

Hypotheses concerning the existence of atoms as indivisible particles were known as early as the ancient Greek philosophers Democritus and Epicurus. The concepts of atoms that prevailed in the 17^th and 18^th centuries were poorly defined. Some scientists supposed that atoms could combine into more complex particles but specific chemical and physical properties were not attributed to atoms. In the period 1780-1803, Lavoisier made careful quantitative measurements which allowed the compositions of compounds to be determined accurately, Proust established the Law of Definite Proportions (each compound always contained the same proportions of elements), and John Dalton had developed the Law of Simple Proportions (compounds composed of the same elements contained simple multiples of component elements). These laws provided a solid foundation for a more thorough atomic theory.

Dalton’s Atomic Theory

John Dalton developed his atomic theory in 1803. Dalton’s theory was different from previous discussions of atoms because it had the weight of careful chemical measurements behind it. Dalton’s theory went beyond just a philosophical statement that there are atoms. Dalton’s theory proposed a number of basic ideas: 1) All matter is composed of indivisible, tiny particles called atoms, 2) atoms can neither be created nor destroyed, 3) all atoms of the same element are identical (have the same mass), 4) elements differ from one another because they have different types of atoms (different mass), 5) compounds are composed of combinations of different elements because the atoms of different elements are bonded to one another, and 6) chemical reactions occur when atoms in compounds are rearranged.

Dalton’s theory did not convince everyone immediately. It did convince a number of chemists right away but several decades were required for all opposition to cease. Dalton’s theory underwent a number of modifications in the next 150 years but many of its ideas are still part of the modern atomic theory. In 1869, Dmitri Mendeleev ascertained that there were groups of elements which had the same (or very similar) chemical properties, the same valence, and similar physical properties and showed that the chemical and physical properties of the elements were periodically repeated. His periodic table remains a major idea in chemistry today.

Discovery of Electrons and Radioactivity

During the investigation of the flow of electric current through gases, scientists discovered rays that were emitted from a cathode discharge tube and had the property of being deflected in electric and magnetic fields. It was determined that these rays consisted of rapidly moving, negatively charged particles called electrons. It was also determined that upon heating or illumination by light, metals emitted electrons. Logically, neutral atoms containing electrons must also contain positively charged particles. Thus, the indivisibility of the atom was disproved. This was further emphasized by Marie Curie’s discoveries that through alpha and beta decay, atoms of one element could transmute into other elements. Curie’s discoveries also determined that atoms of the same element may have different masses (isotopes) and thus the idea that all the atoms of the same element are identical was also lost.

Rutherford’s Planetary Model of the Atom

Now that it was known that the atom had component parts, a new model was needed. In the model proposed by J. J. Thomson in 1903, the atom was represented as a positively charged sphere with the negatively charged electrons distributed around the exterior. This was the so-called “plum-pudding” model with the positive charge playing the role of the pudding and the electrons playing the role of the plums. The next big step in the development of the model of the atom occurred in 1911 with Rutherford’s gold foil experiment. In the Thomson model of the atom, the density of the atom was necessarily small. The mass of the atom divided by the volume of the atom and assuming an even distribution of mass resulted in a low density for the atom.

In 1896, Henri Becquerel discovered that uranium compounds emitted penetrating rays, some of which were massive, high speed, positively charged particles which were later named alpha particles (α). These alpha particles would be detected by a zinc sulfide coated screen (scintillation counter) that emitted a small flash of light every time an alpha particle hit it. Rutherford used these alpha particles and the zinc sulfide coated screen in his gold foil experiment. The alpha particles were fired at a very thin sheet of gold foil. It was thought that all of the alpha particles would pass straight through the gold foil with no deflection. This was because the alpha particles were known to be very dense and, due to the “plum pudding” model of the atom where the mass of the atom was spread out evenly over the volume of the atom, the atoms of gold were thought to have very low density.

While the great majority of the alpha particles did pass straight through the foil with no deflection, to everyone’s surprise, some alpha particles were deflected. In fact, some alpha were bounced almost straight backward by the foil. Rutherford, using Coulomb’s law and Newton’s laws found that the results could be explained only if all the positive charge of the atom were concentrated in a tiny, central core, now called the nucleus. Rutherford’s model of the atom is therefore, called the nuclear modelof the atom. All of the positive charge and essentially all of the mass of the atom are in its nucleus. The atom is 10,000 times as large as the nucleus and is mostly empty space. It was known that electrons are outside the nucleus but how the electrons were arranged in an atom was still a mystery.

Problems with the Planetary Model

The planetary model had two major problems immediately. According to classical mechanics, a charged particle being accelerated (speeding up, slowing down, or turning a corner) would always emit electromagnetic radiation. That means that an orbiting electron would constantly emit energy and thus move closer to the nucleus until eventually it would collapse into the nucleus. Clearly this doesn’t happen and thus it opposes the idea that electrons move like planets. The second problem was that atoms gain energy (in the form of heat or light) and re-emit the energy in an exact set of light frequencies. The magnitude of the energy involved in this light emission is too small to be involved with nuclear changes and therefore, the electron configuration must be responsible for the light emission by atoms. The planetary model offered no explanation for the light spectrum of atoms.

Figure 20.3.2

A major clue to the electron arrangement in an atom came from studying the light emitted by atoms. When electricity is passed through gaseous atoms, the atoms emit a spectrum of light that is specific for that element. The hydrogen atom, for example, emits a pinkish light but when that light is passed through a prism, we see a very few frequencies of light that are quite specific for hydrogen. The energies of these light frequencies is much too small to be involved in the nucleus of atoms, therefore, any explanation of these wavelengths would have to involve the electron arrangement in atoms.

The Bohr Model of the Atom

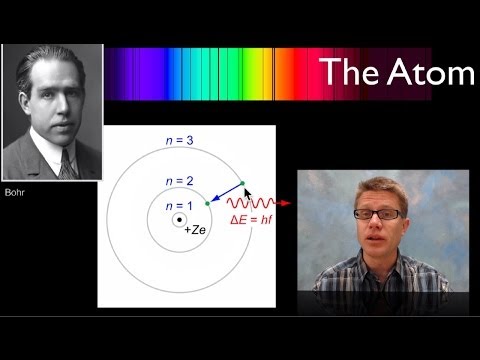
Niels Bohr attempted to join the nuclear model of the atom with Einstein’s quantum theory of light and with his own idea of electron energy levels to explain the electron arrangement within the atom. Bohr started with the planetary model of electron arrangement but postulated that electrons in stable orbits would not radiate energy even though the electrons were being accelerated by traveling in circular paths. Bohr hypothesized that the electrons were organized into stepwise energy levels within the electron cloud and only radiated energy when the electrons moved from one energy level to another. Bohr’s hypothesis suggested that the energy of atomic electrons came in packages and only whole packages could be absorbed or emitted. This quantization of energy allowed electrons to only absorb or emit exact amounts of energy to move from one energy level to another.

The quantization of energy is not apparent in everyday experience. If we could observe molecular sized automobiles traveling down miniature roads, we would see cars traveling at 7 miles per hour, or 14 miles per hour, or 21 miles per hour, but never at 9 or 17 miles per hour. The quantization of energy means that energy comes in packages and when energy is added to an object, whole packets of energy must be added. This is the explanation for why atomic electrons are only allowed to have certain amounts of energy and therefore, occupy certain energy levels. The lowest energy level for an electron is near the nucleus and each quanta of energy added moves the electron to the next distant energy level. Einstein’s theory says that each light photon has an energy of hf, where h is Planck’s constant, and f is the frequency. The emission of a photon of light from an atom indicates a change in energy level for an electron such that

hf=E_higher−E_lower

The energy of an orbit is related to the inverse of the square of the orbit number. The energy of an electron in a given energy level of hydrogen is calculated by

E_n=(−2.17×10⁻¹⁸ J)(1/n²)

The radius of an orbit is related to the square of the orbit number.

Bohr calculated allowed electron energy levels for the hydrogen atom and found the emission spectrum of hydrogen to match perfectly with particular electron transitions between his suggested energy levels. Other electron transitions predicted electromagnetic frequencies outside the visible range and when those were looked for, they were present and also matched precisely with theoretical calculations.

Examples

Example 20.3.1

For the hydrogen atom, determine

1. the energy of the innermost energy level (n=1).
2. the energy of the second energy level.
3. the difference between the first and second energy levels.

Solution

1. E_n=(−2.17×10⁻¹⁸ J)(1/n²) E₁=(−2.17×10⁻¹⁸ J)(1/1²)=−2.17×10⁻¹⁸ J
2. E_n=(−2.17×10⁻¹⁸ J)(1/n²) E₂=(−2.17×10⁻¹⁸ J)(1/2²)=−5.43×10⁻¹⁹ J
3. E₂₋₁=(−5.43×10⁻¹⁹ J)−(−2.17×10⁻¹⁸ J)=1.63×10⁻¹⁸ J

This is the amount of energy that would need to be added to a 1^st energy level electron to raise it to the second energy level.

Example 20.3.2

According to the Bohr model, how many times larger is the second level hydrogen orbit compared to the first level hydrogen orbit?

Solution

4 times

Launch the PLIX Interactive below to observe Bohr’s Atomic Model. You can excite the red electron and drag it to a higher orbital to model what would happen if the electron gained energy. Then, use the table to determine the wavelength of radiation emitted when the electron releases this energy and returns to its lowest orbital:

Interactive Element

Summary

• Rutherford, using Coulomb’s law and Newton’s laws, found that the results of his 'gold foil experiment' could be explained only if all the positive charge of the atom were concentrated in a tiny, central core, now called the nucleus.
• The atom is 10,000 times as large as the nucleus and is mostly empty space.
• The energies of light frequencies in the emission spectrum of atoms is much too small to be involved in the nucleus of atoms, therefore, any explanation of these wavelengths would have to involve the electron arrangement.
• Bohr hypothesized that the electrons were organized into stepwise energy levels within the electron cloud in a planetary model and only radiated energy when the electrons were changing from one energy level to another.
• Bohr suggested that the energy levels were quantized, that is, the energy held by the atomic electrons came in packages and only whole packages could be absorbed or emitted.
• Einstein’s theory says that each light photon has an energy of hf, where h is Planck’s constant, and f is the frequency. The emission of a photon of light from an atom indicates a change in energy level for an electron such that hf=E_higher−E_lower
• The energy of an electron in a given energy level of hydrogen is calculated by

E_n=(−2.17×10⁻¹⁸ J)(1/n²).

Review

1. Why did Rutherford suggest that the positive charge in an atomic nucleus is concentrated in a tiny region rather than spread evenly throughout the atom?
2. The absorption spectrum of an element has the same frequencies as the emission spectrum. How does Bohr’s theory explain this?
3. Why do scientists say the planetary model can’t be correct because the electrons would collapse into the nucleus?
4. If you were trying to explain the idea of quantization to younger students, do you think you should use water or money as an example? Why?

Explore More

Use this resource to answer the questions that follow.

1. Why does the Rutherford planetary model predict that the electron would collapse into the nucleus?
2. Bohr used Planck’s suggestion that energy emitted by matter was not emitted in continuous quantities but in _________ bundles.
3. Bohr’s calculations were based on the atoms of which element?

Additional Resources

Videos:

Real World Application: Scanning Tunneling Microscopy