6.2.5: Applications of Parabolas
- Page ID
- 14756
Applications of Parabolas
Kelly is nearly done with the chapter on parabolas in her math class. She has become pretty familiar with the general shape of a parabola, and has started noticing the use of parabolas all around her. One thing that she hasn't figured out yet is why satellite-TV dishes are 3D parabolas. She is sure it isn't a coincidence, but doesn't know what it is about a parabolic shape that is important.
Do you know?
Applications of Parabolas
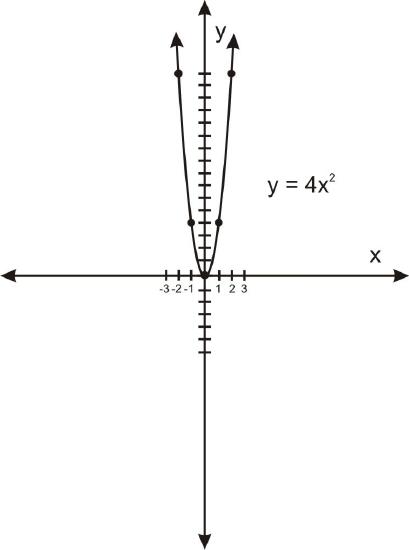
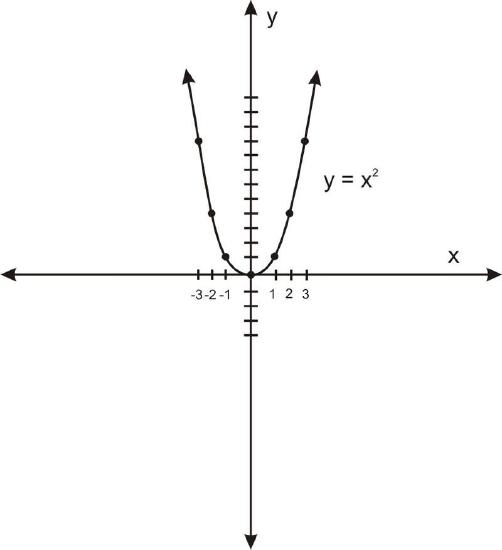
There is a very interesting property of parabolas. This is the fact that all parabolas have the same shape. Or, in the language of geometry, any two parabolas are similar to one another. This means that any parabola can be scaled in or out to produce another parabola of exactly the same shape. This may come across as surprising, because parabolas where \(\ x^{2}\) has a large coefficient certainly look much “steeper” than parabolas with a small coefficient when examined over the same domain, as shown in the graphs below.
|
a. Tall and skinny:
|
b. Short and wide:
|
|---|
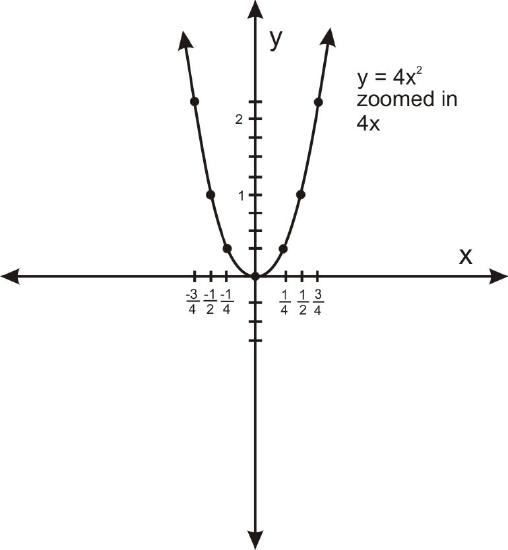
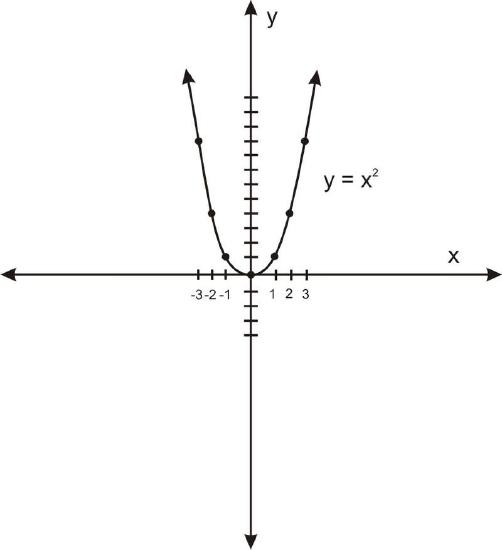
But when one of the parabolas is scaled appropriately, these parabolas are identical:
|
a. Tall and skinny - zoomed in by 4x:
|
b. Short and wide:
|
|---|
This fact about parabolas can be seen from an algebra standpoint given the fact that all parabolas are generated from a line and a point not on that line. This configuration of generating objects, a line and a point, is always the same shape. Any other line and point looks exactly the same—simply zoom in or out until the line and point are the same distance from one another. So the shapes that any two such configurations generate must also be the same shape.
From an analytic geometry standpoint, there were two factors that might affect the shape of the parabola. The first is the distance between the cutting plane and the apex of the cone. But cones have the same proportions at any scale, so no matter what this distance, the picture can be reduced or enlarged, affecting this distance but not the shape of the cone or plane. So this parameter does not actually change the shape of the conic section that results. The other factor is the shape of the actual cone. This is its steepness, defined by the angle at the apex, or equivalently by the ratio between the radius and the height at any point. This is a bit trickier. It’s not at all obvious that short, squat cones and tall, skinny cones would produce parabolas of the same shape.
|
a. Tall and skinny:
|
b. Short and wide:
|
|---|
According to what we found, any parabola produced by slicing any cone resulted in an equation of this form:
\(\ y=a x^{2}\)
We want to show that if we generate two such parabolas, that they actually have the same shape. So suppose we use two cone constructions and come up with these parabolas:
\(\ y=a_{1} x^{2}\) and \(\ y=a_{2} x^{2}\). We want to show there is some scale factor, call it \(\ f\), that shrinks or enlarges \(\ y=a_{1} x^{2}\) into \(\ y=a_{2} x^{2}\). To keep a shape the same, the scale factor needs to affect both the \(\ x-\) and \(\ y-\text { variables }\). So we need to find an \(\ f\) such that \(\ (f y)=a_{1}(f x)^{2}\) is equivalent to \(\ y=a_{2} x^{2}\). The first equation can be written \(\ y=\left(a_{1} f\right) x^{2}\), which is equivalent to the second equation when \(\ a_{1} f=a_{2}\), or when \(\ f=\frac{a_{2}}{a_{1}}\). Such an \(\ f\) always exists for non-negative numbers \(\ a_{1}\) and \(\ a_{2}\). So the parabolas are indeed the same shape. If \(\ a\) is less than zero, then the parabola can be reflected vertically to produce a parabola of the same shape and with positive coefficient \(\ a\).
Parabola Applications
Burning Mirrors
Diocles (∼240−180 BCE) was a mathematician from Ancient Greece about whom we know very little. However, we know enough from a few scant documents that he thought about an important application of parabolas. It comes from what is sometimes called the “optical property” of parabolas. Remember the optical property of ellipses: lines from one focus “bounce off” the side of the ellipse to hit the other focus.
For parabolas, since parabolas have only one focus, the directrix plays a role. For the parabola, the optical property is that lines perpendicular to the directrix “bounce off” the parabola and converge at the focus. Or, alternatively, lines from the focus “bounce off” off the parabola and continue perpendicular to the directrix. As with the ellipse, “bouncing off” means that the two lines meet the parabola at equal angles to the tangent.
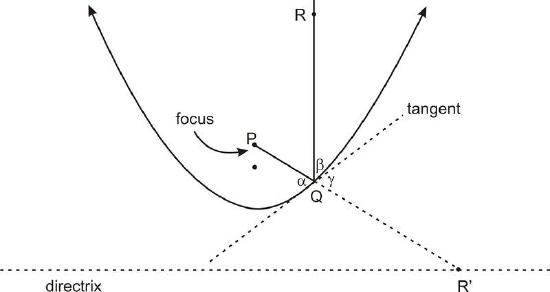
In the above diagram, the optical property states that ◻ α≅◻ β. The proof is similar to the proof of the optical property of ellipses. In the above diagram P is the focus and Q is a point on the parabola. Let R′ be the point on the directrix that is obtained by extending PQ. Then PR′, the straight line, is clearly the shortest distance between P and R′ that passes through the tangent line. Let R be on the line lying directly above Q such that QR=QR′. The R can be thought of as R′, reflected across the tangent line. Then ◻ α≅◻ γ (vertical angles) and ◻ γ≅◻ β (reflected angles), and so ◻ α≅◻ β (transitive property).
The optical property has some interesting applications. Diocles described one potential application in his document “On Burning Mirrors”. He envisioned a parabolic-shaped mirror (basically a parabola rotated about its line of symmetry) which would collect light from the sun and focus it on the focal point, creating enough of a concentration of light to start a fire at that point. Some claim that Archimedes attempted to make such a contraption with copper plates to fight the Romans in Syracuse.
|
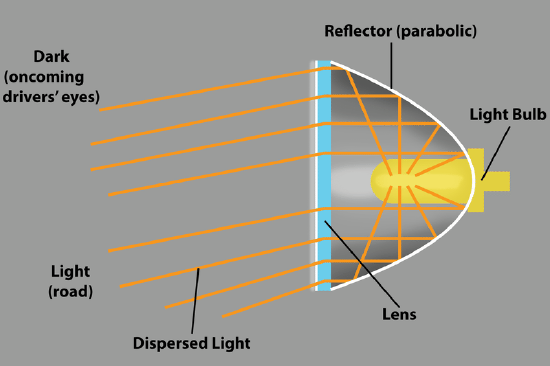
Headlights: The optical property is also responsible for parabola-shaped unidirectional lights, such as car headlights. If a bulb is placed at the focus of a parabolic mirror, the light rays reflect off the mirror parallel to each other, making a focused beam of light.
|
Cassegrain Telescopes: Satellite telescopes take advantage of the optical property of parabolas to collect as much light from a distant star as possible. The dish of the satellite below is parabolic in shape and reflects light to the point in the middle.
|
|---|
Examples
Earlier, you were asked a question about why satellite-TV dishes are 3D parabolas.
Solution
The satellite dish is a 3D parabola so that all of the signal it collects over a wide area will be concentrated at the focus of the parabola, significantly increasing the reception.
Explain why not all ellipses are similar the way parabolas are. While enlarging or shrinking doesn’t work to make two ellipses identical, how can you change the view of two ellipses that have different shapes so that they look the same?
Solution
The eccentricity of ellipses defines the shape, so when the eccentricity is different for two ellipses, the ellipses are not similar to one another. Viewing one of the ellipses at an angle, however, changes the perceived eccentricity of that ellipse, and the angle can be chosen to match the perceived eccentricity to the eccentricity of the other ellipse, producing an image that is similar to the other ellipse.
If nothing was used to deflect light before it entered a "burning mirror," where would the sun have to be in relationship to you and the place you want to start a fire? Why is this a constraint? Design a way to circumvent this constraint.
Solution
The fire-locale must lie on the segment between you and the sun. This is a problem because to start a ground fire, you would have to wait until evening when the sun is low in the sky so you could aim your lens at the ground, unfortunately the sun is not as bright in the evening so you would lose a significant amount of power. A lens or mirror that changes the angle of the suns rays could help you work around this constraint.
In the above diagram of a car headlight, the lens directs the beams of light downwards, to keep them out of the eyes of oncoming drivers, if that was the only purpose of the lens, alternatively the lens could be omitted and the headlight could just be angled down slightly. But there is another purpose to the lens. What is it?
Solution
The lens also expands the array of light which is why it is called “dispersed light.” Without the lens, the headlight would only illuminate a strip the width of the headlight itself, which would not be very useful for driving.
A satellite dish antenna is to be constructed in the shape of a paraboloid. The paraboloid is formed by rotating the parabola with focus at the point (25, 0) and directrix \(\ x=−25\) about the x-axis, where x and y are in inches. The diameter of the antenna is to be 80 inches.
- Find the equation of the parabola and the domain of x.
- Sketch the graph of the parabola, showing the location of the focus.
- A receiver is to be placed at the focus. The designer has warned that a user or installer should take care, the receiver would hit the ground and could be damaged if the antenna were placed "face-down". Determine algebraically whether this observation is correct.
Solution
- \(\ x=\frac{1}{100} y^{2}\)
The domain is the positive real number set.
- Sketch the graph of the parabola, showing the location of the focus.
- Yes, as discovered in part a and shown in the graph of part b, the focus is at x = 25, while the dish only extends to x = 16.
Review
- football with an initial vertical velocity of 96 feet per second. After how many seconds will the ball reach its maximum height above sea level? What is the maximum height?
- A shot-gun is discharged vertically upward at a height of 3 feet above the ground. If the bullet has an initial muzzle velocity of 200 feet per second, what maximum height will it reach before it starts to fall to the ground? a) 628 feet b) 1,878 feet c) 20.87 feet d) 199.33 feet
- An over-zealous golfer hits a flop shot with a sand wedge to get out of the corner of a sand trip with an initial vertical velocity of 45 feet per second. What is the maximum height that the golf ball will reach? a) 45 feet b) 13.19 feet c) 36.64 feet d) 95.26 feet
- You are standing on the top of a 1680 ft tall hill, and throw a small ball upwards. At every second, you measure the distance of the ball from the ground. Exactly t seconds after you throw the object, its height, (measured in feet) is \(\ h(t)=-16 t^{2}+256 t+1680\) a) Find \(\ h(3)\) the ball position 3 seconds after you throw it. b) How much does the object travel during the two seconds between 5 seconds and 7 seconds? c) How long does it take for the object to reach a height of 2640 ft? d) How long does it take for the object to hit the ground?
- A student participating in a game of kick ball kicked the ball with an initial vertical velocity of 32 feet per second. Its height above the earth in feet is given by \(\ s(t)=-16 t^{2}+32 t\) where t is time in seconds. What is the maximum height reached by the ball? HINT - Graph the parabola (you only need to worry about the section where 0≤t≤2 or rewrite the equation to find the vertex.
- An arch over the entrance to an enchanted trail has a parabolic shape, the arch has a height of 25 feet and it is 30 feet between the support pillars. Find an equation that models the arch, using the x-axis to represent the ground of the park. State the focus and directrix.
- A satellite dish has a parabolic shape with a diameter of 80 meters. The collected tv signals are focused on a single point, called the "focal" point, which is the focus of the paraboloid (the cross-section of the parabola). If the focal length is 45 meters, find the depth of the dish, rounded to one decimal place.
- When new highways go in, they are often designed with parabolic surfaces which allow water to drain off. A new highway is being laid, it is 32 feet wide and is .4 feet higher in the center of the highway then on the sides. a) Find an equation if the parabola that models the highway surface (assume that the origin is at the center of the highway) b) How far from the center of the highway is the surface of the road .1 feet lower than in the middle?
- The internal distance of the sketch of an arch is 8cm and the height of the arch is 9cm. Assuming the scale 1cm = 2m, work out a formula which calculates the actual height of the inside edge of this structure (y), in metres at any horizontal distance x measured from the origin point which is the floor at the center of the arch.
A new bridge has been built for foot traffic cross a river. The two towers on either end of the bridge are 50 feet high and 300 feet away from each other. The supporting cables (2) are connected at the top of the towers and hang in a curve that forms the shape of a parabola. There are vertical cables that connect the walkway to the supporting cables. These cables connect every 15 feet from the walkway up to the supporting cables. At the center of the bridge, the parabola is 5 feet above the walkway.
Specialty Cable Company back east sells cable for $52.75 per 10 feet with a shipping charge of $300.00 for the entire order. Cables R US, on the West Coast sells cable for $432.90 per 100 feet with a shipping charge of $350.00 for the entire order. Cable had to be purchased in either 10-foot lengths from SCC or in 100-foot lengths from CRUS. Once purchased, the cables can be cut or welded together.
- Write an equation for the parabola that represents each of the support cables.
- Determine the number of vertical cables needed.
- Determine the length of each of the vertical cables.
- How much does it cost to purchase the needed materials from each company?
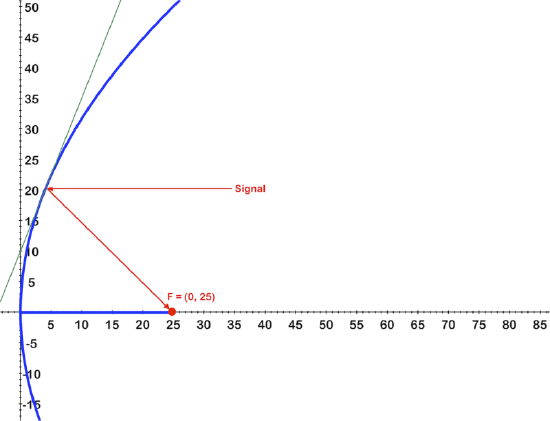
Look at the image. It shows the parabolic cross section of a satellite dish antenna. The equation for the parabola is \(\ x=0.01 y^{2}\)

- What is the focus of the parabola as shown in the image?
- A radio signal comes in at y = 20 as marked in red. Identify where the ray strikes the parabola, give coordinates.
- Identify the angle measure between the incoming ray and a line between the strike point and the focus.
- An incoming ray and its reflected ray make angles of equal measure with a line tangent to the curved surface. Use the angle you just measured between the signal and the reflected line to calculate the measures of the angles between the incoming signal and the tangent to the point of impact and from the tangent to the reflected ray. Are they the same? Measure with a protractor to confirm your calculations.
- Find the equation for the tangent line. Use angle C to find its slope.
- Randomly choose another incoming ray. Calculate the angles as before. Is the incoming ray tangent to the parabola at the point where the incoming ray strikes the graph? How do you know?
- What can you say about the direction a reflected ray takes whenever the incoming ray is parallel to the axis of the parabola? Can you see why the name "focus" is used and why television satellite antennas and other listening devices are made in the shape of a paraboloid?
Resources
Vocabulary
| Term | Definition |
|---|---|
| burning mirror | The burning mirror is a highly reflective parabolic dish used to focus sunlight onto a single point, the focus of the parabola. |
| Ellipse | Ellipses are conic sections that look like elongated circles. An ellipse represents all locations in two dimensions that are the same distance from two specified points called foci. |
| Ellipses | Ellipses are conic sections that look like elongated circles. An ellipse represents all locations in two dimensions that are the same distance from two specified points called foci. |
| Parabola | A parabola is the set of points that are equidistant from a fixed point on the interior of the curve, called the '''focus''', and a line on the exterior, called the '''directrix'''. The directrix is vertical or horizontal, depending on the orientation of the parabola. |
| parabolic dish | A parabolic dish is a surface generated by a parabola being revolved about its axis of symmetry. |
| Unidirectional | Unidirectional means focusing in one (uni-) specific direction. |