6.1: Basic Trigonometric Identities

The basic trigonometric identities are ones that can be logically deduced from the definitions and graphs of the six trigonometric functions. Previously, some of these identities have been used in a casual way, but now they will be formalized and added to the toolbox of trigonometric identities.

How can you use the trigonometric identities to simplify the following expression?

\(\left[\frac{\sin \left(\frac{\pi}{2}-\theta\right)}{\sin (-\theta)}\right]^{-1}\)

Trigonometric Identities

An identity is a mathematical sentence involving the symbol “=” that is always true for variables within the domains of the expressions on either side.

Reciprocal Identities

The reciprocal identities refer to the connections between the trigonometric functions like sine and cosecant. Sine is opposite over hypotenuse and cosecant is hypotenuse over opposite. This logic produces the following six identities.

• \(\sin \theta=\frac{1}{\csc \theta}\)
• \(\cos \theta=\frac{1}{\sec \theta}\)
• \(\tan \theta=\frac{1}{\cot \theta}\)
• \(\cot \theta=\frac{1}{\tan \theta}\)
• \(\sec \theta=\frac{1}{\cos \theta}\)
• \(\csc \theta=\frac{1}{\sin \theta}\)

Quotient Identities

The quotient identities follow from the definition of sine, cosine and tangent.

• \(\tan \theta=\frac{\sin \theta}{\cos \theta}\)
• \(\cot \theta=\frac{\cos \theta}{\sin \theta}\)

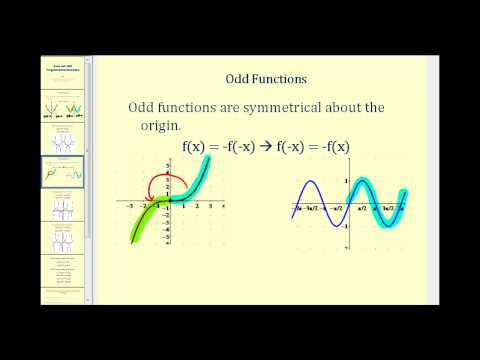
Odd/Even Identities

The odd-even identities follow from the fact that only cosine and its reciprocal secant are even and the rest of the trigonometric functions are odd.

• \(\sin (-\theta)=-\sin \theta\)
• \(\cos (-\theta)=\cos \theta\)
• \(\tan (-\theta)=-\tan \theta\)
• \(\cot (-\theta)=-\cot \theta\)
• \(\sec (-\theta)=\sec \theta\)
• \(\csc (-\theta)=-\csc \theta\)

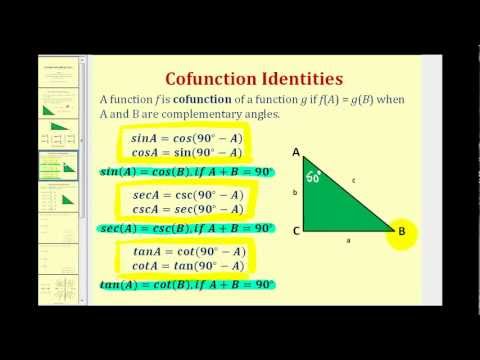
Cofunction Identities

The cofunction identities make the connection between trigonometric functions and their “co” counterparts like sine and cosine. Graphically, all of the cofunctions are reflections and horizontal shifts of each other.

• \(\cos \left(\frac{\pi}{2}-\theta\right)=\sin \theta\)
• \(\sin \left(\frac{\pi}{2}-\theta\right)=\cos \theta\)
• \(\tan \left(\frac{\pi}{2}-\theta\right)=\cot \theta\)
• \(\cot \left(\frac{\pi}{2}-\theta\right)=\tan \theta\)
• \(\sec \left(\frac{\pi}{2}-\theta\right)=\csc \theta\)
• \(\csc \left(\frac{\pi}{2}-\theta\right)=\sec \theta\)

Examples

Example 1

Earlier, you were asked how you could simplify the trigonometric expression:
\(\left[\frac{\sin \left(\frac{\pi}{2}-\theta\right)}{\sin (-\theta)}\right]^{-1}\)

It can be simplified to be equivalent to negative tangent as shown below:

\(
\begin{aligned}
\left[\frac{\sin \left(\frac{\pi}{2}-\theta\right)}{\sin (-\theta)}\right]^{-1} &=\frac{\sin (-\theta)}{\sin \left(\frac{\pi}{2}-\theta\right)} \\
&=\frac{-\sin \theta}{\cos \theta} \\
&=-\tan \theta
\end{aligned}
\)

Example 2

If \(\sin \theta=0.87,\) find \(\cos \left(\theta-\frac{\pi}{2}\right)\)

While it is possible to use a calculator to find \(\theta\), using identities works very well too.

First you should factor out the negative from the argument. Next you should note that cosine is even and apply the odd-even identity to discard the negative in the argument. Lastly recognize the cofunction identity.

\(
\cos \left(\theta-\frac{\pi}{2}\right)=\cos \left(-\left(\frac{\pi}{2}-\theta\right)\right)=\cos \left(\frac{\pi}{2}-\theta\right)=\sin \theta=0.87\)

Example 3

If \(\cos \left(\theta-\frac{\pi}{2}\right)=0.68\) then determine \(\csc (-\theta)\)

You need to show that \(\cos \left(\theta-\frac{\pi}{2}\right)=\cos \left(\frac{\pi}{2}-\theta\right)\)

\(
\begin{aligned}
0.68 &=\cos \left(\theta-\frac{\pi}{2}\right) \\
&=\cos \left(\frac{\pi}{2}-\theta\right) \\
&=\sin (\theta)
\end{aligned}
\)

Then, \(\csc (-\theta)=-\csc \theta\)

\(
\begin{array}{l}
=-\frac{1}{\sin \theta} \\
=-(0.68)^{-1} \\
\approx-1.47
\end{array}
\)

Example 4

Use identities to prove the following: \(\cot (-\beta) \cot \left(\frac{\pi}{2}-\beta\right) \sin (-\beta)=\cos \left(\beta-\frac{\pi}{2}\right)\).

When doing trigonometric proofs, it is vital that you start on one side and only work with that side until you derive what is on the other side. Sometimes it may be helpful to work from both sides and find where the two sides meet, but this work is not considered a proof. You will have to rewrite your steps so they follow from only one side. In this case, work with the left side and keep rewriting it until you have \(\cos \left(\beta-\frac{\pi}{2}\right)\)

\(
\begin{aligned}
\cot (-\beta) \cot \left(\frac{\pi}{2}-\beta\right) \sin (-\beta) &=-\cot \beta \tan \beta \cdot-\sin \beta \\
&=-1 \cdot-\sin \beta \\
&=\sin \beta \\
&=\cos \left(\frac{\pi}{2}-\beta\right) \\
&=\cos \left(-\left(\beta-\frac{\pi}{2}\right)\right) \\
&=\cos \left(\beta-\frac{\pi}{2}\right)
\end{aligned}
\)

Example 5

Prove the following trigonometric identity by working with only one side.

\(\cos x \sin x \tan x \cot x \sec x \csc x=1\)

\(
\begin{aligned}
\cos x \sin x \tan x \cot x \sec x \csc x &=\cos x \sin x \tan x \cdot \frac{1}{\tan x} \cdot \frac{1}{\cos x} \cdot \frac{1}{\sin x} \\
&=1
\end{aligned}
\)

Review

1. Prove the quotient identity for cotangent using sine and cosine.

2. Explain why \(\cos \left(\frac{\pi}{2}-\theta\right)=\sin \theta\) using graphs and transformations.

3. Explain why \(\sec \theta=\frac{1}{\cos \theta}\)

4. Prove that \(\tan \theta \cdot \cot \theta=1\).

5. Prove that \(\sin \theta \cdot \csc \theta=1\).

6. Prove that \(\sin \theta \cdot \sec \theta=\tan \theta\)

7. Prove that \(\cos \theta \cdot \csc \theta=\cot \theta\)

8. If \(\sin \theta=0.81,\) what is \(\sin (-\theta) ?\)

9. If \(\cos \theta=0.5,\) what is \(\cos (-\theta) ?\)

10. If \(\cos \theta=0.25,\) what is \(\sec (-\theta) ?\)

11. If \(\csc \theta=0.7,\) what is \(\sin (-\theta) ?\)

12. How can you tell from a graph if a function is even or odd?

13. Prove \(\frac{\tan x \cdot \sec x}{\csc x} \cdot \cot x=\tan x\)

14. Prove \(\frac{\sin ^{2} x \cdot \sec x}{\tan x} \cdot \csc x=1\).

15. Prove \(\cos x \cdot \tan x=\sin x\)