4.7: Mode
- Page ID
- 5721
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)The Mode
Before class begins, bring out the blocks that you and your classmates chose from the pail for the concept on mean. In addition, have the grid paper on display where each student in your class posted his or her number of blocks.
To begin the class, refer to the comments on the measures of central tendency that were recorded from the concept on mean, when the brainstorming session occurred. Highlight the comments that were made with regard to the mode of a set of data and discuss this measure of central tendency with your classmates. Once the discussion has been completed, choose a handful of blocks like you did before, with your classmates doing the same.
The mode of the set of blocks can be given as a quantitative value or as a qualitative value. You and your classmates can tell from the grid paper which number of blocks was picked most. The chart below shows that 5 students each picked 5 blocks from the pail. This is a mode for quantitative data, since the answer is in the form of a number.
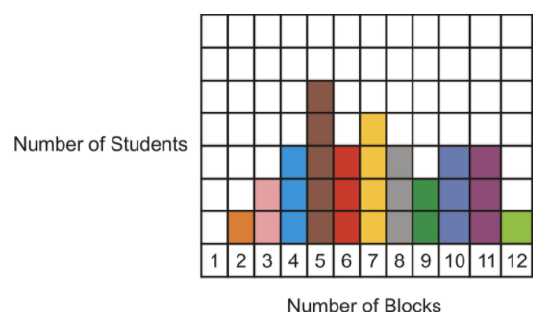
To extend the mode to include qualitative data, you and your classmates should each now determine the color or colors of block(s) that appear most often in each of your handfuls. To do this, group your blocks according to color and count them, and have your classmates do the same. There may be more than 1 color that occurs with the same highest frequency. The color(s) that appear most often for each handful of blocks is the mode for that particular handful.
The mode of a set of data is simply the value that appears most frequently in the set. If 2 or more values appear with the same frequency, each is a mode. The downside to using the mode as a measure of central tendency is that a set of data may have no mode or may have more than 1 mode. However, the same set of data will have only 1 mean and only 1 median. The word modal is often used when referring to the mode of a data set. If a data set has only 1 value that occurs most often, the set is called unimodal. Likewise, a data set that has 2 values that occur with the greatest frequency is referred to as bimodal. Finally, when a set of data has more than 2 values that occur with the same greatest frequency, the set is called multimodal. When determining the mode of a data set, calculations are not required, but keen observation is a must. The mode is a measure of central tendency that is simple to locate, but it is not used much in practical applications.
Finding the Mode
1. The posted speed limit along a busy highway is 65 miles per hour. The following values represent the speeds (in miles per hour) of 10 cars that were stopped for violating the speed limit:
76 81 79 80 78 83 77 79 82 75
What is the mode?
There is no need to organize the data, unless you think that it would be easier to locate the mode if the numbers were arranged from least to greatest. In the above data set, the number 79 appears twice, but all the other numbers appear only once. Since 79 appears with the greatest frequency, it is the mode of the data values.
Mode = 79 miles per hour
2. The weekly wages of 7 randomly selected employees of Wendy’s were $98.00, $125.00, $75.00, $120.00, $86.00, $92.00, and $110.00, respectively. What is the mode of these wages?
Each value in the above data set occurs only once. Therefore, this data has no mode.
3. 6 students attending a local swimming competition were asked what color bathing suit they were wearing. The responses were red, blue, black, pink, green, and blue.
What is the mode of these responses?
Remember that the mode can be determined for qualitative data as well as quantitative data, but the mean and the median can only be determined for quantitative data. The color blue was the only response that occurred more than once and is, therefore, the mode of this data set.
Mode = blue
Points to Consider
- Is reference made to the mode in any other branch of statistics?
- Can the mode be useful when presenting graphical representations of data?
Examples
Example 1
The ages of 12 randomly selected customers at a local coffee shop are listed below:
23,21,29,24,31,21,27,23,24,32,33,19
What is the mode of the above ages?
The above data set has 3 values that each occur with a frequency of 2. These values are 21, 23, and 24. All other values occur only once. Therefore, this set of data has 3 modes.
Modes - 21, 23, and 24
Example 2
The following table represents the number of times that 100 randomly selected students ate at the school cafeteria during the first month of school:
Number of Times Eating in the Cafeteria: 2 3 4 5 6 7 8
Number of Students: 3 8 22 29 20 8 10
What is the mode of the numbers of times that a student ate at the cafeteria?
When data is arranged in a frequency table, the mode is simply the value that has the highest frequency. Therefore, since the table shows that 29 students ate 5 times in the cafeteria, 5 is the mode of the data set.
Mode = 5 times
Review
- Which of the following measures can be determined for quantitative data only?
a. mean
b. median
c. mode
d. none of these - Which of the following measures can be calculated for qualitative data only?
a. mean
b. median
c. mode
d. all of these - What is the term used to describe the distribution of a data set that has 1 mode?
a. multimodal
b. unimodal
c. nonmodal
d. bimodal - What is the mode of the following numbers, which represent the ages of 8 hockey players? 12, 11, 14, 10, 8, 13, 11, 9
a. 11
b. 10
c. 14
d. 8 - Which of the following measures can have more than 1 value for a set of data?
a. median
b. mode
c. mean
d. none of these - What are the modes of the following sets of numbers?
- 3, 13, 6, 8, 10, 5, 6
- 12, 0, 15, 15, 13, 19, 16, 13, 16, 16
- A student recorded her scores on weekly English quizzes that were marked out of a possible 10 points. Her scores were as follows: 8, 5, 8, 5, 7, 6, 7, 7, 5, 7, 5, 5, 6, 6, 9, 8, 9, 7, 9, 9, 6, 8, 6, 6, 7
What is the mode of her scores on the weekly English quizzes? - The following table represents the number of minutes that students spent studying for a math test:
| Studying Time (minutes) | Number of Students |
|---|---|
| [0−10) | 2 |
| [10−20) | 10 |
| [20−30) | 6 |
| [30−40) | 4 |
| [40−50) | 3 |
What is the mode of the amounts of time spent studying for the math test?
- A pre-test for students entering high school mathematics was given to 48 students. The following table shows the number of questions attempted out of 50 by each of the students taking the
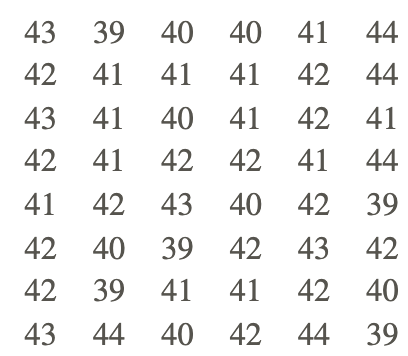
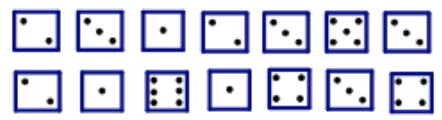
What number of questions was attempted the most by the students? - A die was tossed 14 times. What is the mode of the numbers that were rolled?
- The following table represents the number of times that 24 students attended school basketball games during the year:
Number of Games: 5 6 7 8 9
Number of Students: 1 5 3 9 6
What is the mode of the numbers of games that students attended?
- The newly-formed high school soccer team is playing its first season. The following table shows the number of goals it scored during each of its matches:
Number of Goals: 1 2 3
Number of Matches: 8 8 m
If the mean number of goals scored is 2.04, what is the smallest possible value of m if the mode of the numbers of goals scored is 3?
- What is the mode of the following numbers, and what word can be used to describe the distribution of the data set? 5,4,10,3,3,4,7,4,6,5,11,9,5,7
- List 3 examples of how mode could be useful in everyday life?
- The temperature in ∘F on 20 days during the month of June was as follows: 70∘F, 76∘F, 76∘F, 74∘F, 70∘F, 70∘F, 72∘F, 74∘F, 78∘F, 80∘F, 74∘F, 74∘F, 78∘F, 76∘F, 78∘F, 76∘F, 74∘F, 78∘F, 80∘F, 76∘F What is the mode of the temperatures for the month of June?
Additional Resources
PLIX: Play, Learn, Interact, eXplore - Mode: Kittens
Video: Mean, Median, and Mode
Activities: Mode Discussion Questions
Study Aid: Describing Data
Lesson Plans: The Mode Lesson Plan
Practice: Mode
Real World: Mode

