8.2: Angular Momentum

Last updated
Save as PDF

Page ID: 2864

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

So what makes an object more difficult to turn?

The difficulty it requires to push an object through space is called inertia or more precisely translational inertia. Inertia is equal to mass. The difficulty required to turn an object is called rotational inertia or sometimes “moment of inertia”. This is symbolized by the letter I (for inertia).

Try this out. Take a long object like a broomstick or baseball bat. Lay it flat and try to spin it with one hand. This can be difficult. Now instead, stand it upright and just give a twist with your fingers to turn it around. The same object is more difficult to spin one way than the other. Rotational inertia depends on both the mass and the mass distribution of an object. Mass closer to the axis is easier to turn. Mass farther from the axis is harder to turn.

Angular velocity is defined as how quickly an object is turning, and is symbolized by the Greek letter omega: ω. In physics, angular velocity is generally measured in one of two units:

Revolutions per second, or rev/s. A complete rotation or revolution is equivalent to motion through 360-degrees. An object that turns around 30 times in one minute has an angular velocity of 0.5 rev/s.
Radians per second or rad/s. A radian is the distance around the edge of a circle of radius 1. It takes 2π radians to complete one circle, so 2π−radians are equivalent to 1 revolution (360 degrees).

Use the simulation below to learn more about how a balancing pole can increase the rotational inertia of a tightrope walker and decrease his angular acceleration around the rope:

Interactive Element

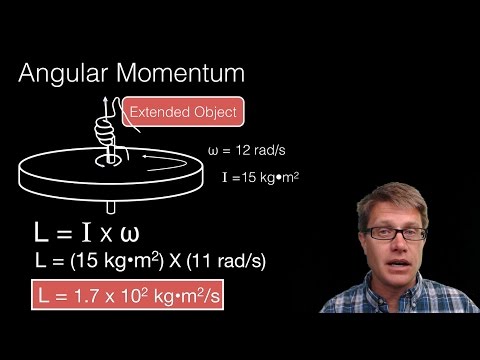
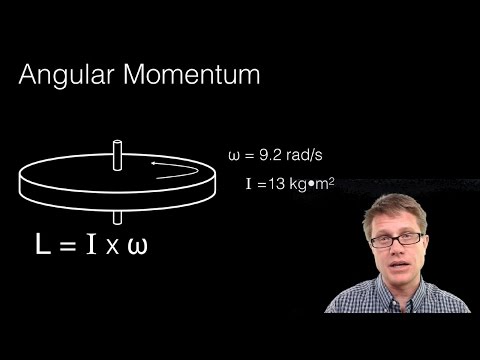
Linear momentum is defined as the product of mass and linear velocity (p=mv). In the same way, angular momentum is defined as the product of rotational inertia and angular velocity. The formula for angular momentum is stated as:

L=Iω

where I is the rotational inertia (a term related to the distribution of mass) and the Greek letter omega ω is the angular velocity. Just like momentum in a given direction, objects undergoing rotation obey a similar conservation principle called conservation of angular momentum, which can be expressed as Iiωi=Ifωf.

An important difference is that in linear momentum, the inertia is always the same. In angular momentum, the rotational inertia I and the angular velocity ω can change. Perhaps you’ve noticed that when a spinning figure skater pulls in her arms close to her body, her rotational velocity increases. Or perhaps you’ve seen a high driver spring off the diving board, tuck his legs close to his body, and spin quickly. What’s going on? In each case the person brings more of their mass closer to the axis about which their body spins. The result is that their angular velocity increases.

The conservation of angular momentum ensures that, should the mass in the system move closer to the axis of rotation, the system will spin (rotate) more quickly. A classic demonstration of the conservation of angular momentum is shown in the following video. As the student in the figure moves the weights inward toward his body, his angular velocity increases, but his angular momentum stays constant.

Summary

The angular momentum of an object is the product of rotational inertia and angular velocity.
The angular velocity of an object is how quickly an object is turning.
The rotational inertia is the difficultly required to turn an object.

Review

You have two coins; one is a standard U.S. quarter, and the other is a coin of equal mass and size, but with a hole cut out of the center.
1. Which coin has a higher moment of inertia?
2. Which coin would have the greater angular momentum if they are both spun at the same angular velocity?
A star is rotating with a period of 10.0 days. It collapses with no loss in mass to a white dwarf with a radius of .001 of its original radius.
1. What is its initial angular velocity?
2. What is its angular velocity after collapse?
A merry-go-round consists of a uniform solid disc of 225kg and a radius of 6.0m. A single 80kg person stands on the edge when it is coasting at 0.20 revolutions per sec. How fast would the device be rotating after the person has walked 3.5m toward the center. (The moments of inertia of compound objects add.)

Explore More

The system pictured in the video above (which includes the student, weights, and spinning seat) has an initial rotational inertia Ii and an initial angular velocity ωi 2.00 rev/s. After the student pulls the weights toward his chest, the final rotational inertia of the system is only 80% of its initial rotational inertia- that is 0.800 Ii.
Assuming that the angular momentum of the system is conserved, what is the final angular velocity of the system?

Additional Resources

Study Guide: Circular Motion Study Guide

Video: Angular Momentum - Overview

Interactives: Bowling Alley, Unicycle

Video: