11.2: Transverse Wave

Last updated
Save as PDF

Page ID: 2880

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)

This surfer rides a giant wave at the legendary big wave surf break known as as "Jaws" in Maui, HI. Massive waves, such as this one, transfer huge amounts of energy.

Transverse Waves

Types of Waves

Water waves and the waves that travel along a rope are mechanical waves. Mechanical waves require a material medium such as water, air, or rope. Light waves, however, are electromagnetic waves and travel without a material medium. They are not mechanical waves.

In all types of mechanical waves, energy moves from one place to another while the media carrying the wave only vibrates back and forth in position. One type of mechanical wave is the transverse wave. In the case of transverse waves, the movement of the medium is perpendicular to the direction of the energy movement.

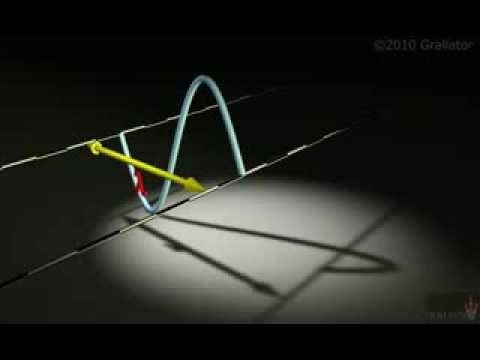
In the sketch above, consider the transverse wave produced when the boy jerks one end of a rope up and down while the other end is tied to a tree. The energy spent by the boy transfers permanently down the rope to the tree. The rope, however, only moves up and down. If we stuck a piece of tape somewhere on the rope, we would see that the particles of medium do not travel with the energy. After the wave has passed by, the piece of tape would still be in the same place it was before the wave approached. In all transverse waves, the movement media vibrates perpendicularly to the direction of wave motion, and the medium is not permanently moved from one place to another.

Frequency, Wavelength, and Velocity

Waves are identified by several characteristics. There is a center line where the medium would be if there were no wave, which is sometimes describes as the undisturbed position. The displacement of the medium above this undisturbed position is called a crest and the displacement below the undisturbed position is called a trough. The maximums of the crest and trough are equal and are called the amplitude. The distance between equivalent positions on succeeding waves is called the wavelength. The wavelength could be measured from a crest to the next crest or from a trough to the next trough, and is commonly represented with the Greek letter lambda, λ.

The time interval required for one complete wave to pass a point is called the period. During the period of the wave, an entire wavelength from one crest to the next crest passes a position. The number of waves that pass a single position in one second is called the frequency. The period of a wave and its frequency are reciprocals of each other.

f=1/T

The units for the period are seconds and the units for frequency are s^-1 or 1s. This unit has also been given the name Hertz (Hz).

Another important characteristic of a wave is its velocity. The wave velocity is different from the velocity of the medium; the wave velocity is the velocity of the linearly transferred energy. Since the energy travels one wavelength, λ, in one period, T, the velocity can be expressed as distance over time:

v=λ/T.

Since period and frequency are reciprocals, the speed of the wave could also be expressed as v=λf.

Examples

Example 11.2.1

A wave has a frequency of 262 Hz. What is the time lapse between successive wave crests?

Solution

The time lapse between successive crests would be the period and the period is the reciprocal of the frequency.

T=1/f=1262 s⁻¹=0.00382 s

Example 11.2.2

A wave has a frequency of 262 Hz has a wavelength of 1.29 m. What is the velocity of the wave?

Solution

v=λf=(1.29 m)(262 s⁻¹)=338 m/s

Do you think you could estimate the wavelength of ocean waves just by observing how frequently they hit the boardwalk? How would the wind affect this wavelength? Play around with the PLIX Interactive below to learn more about ocean waves:

Interactive Element

Summary

Mechanical waves require a material medium such as water, air, or rope.
In all types of mechanical waves, energy moves from one place to another while the media carrying the wave only vibrates back and forth in position.
One type of mechanical wave is the transverse wave, in which the movement of the medium is perpendicular to the direction of the energy propagation.
The maximum displacement of the medium is the distance from the undisturbed position to the top of a crest, or the amplitude.
The distance along the line of motion of the wave from equivalent positions on succeeding waves is the wavelength.
The time interval required for one entire wave to pass a point is the period.
The number of periods per second is the wave's frequency.
The period of a wave and its frequency are reciprocals of each other.
The velocity of the wave's energy transfer is given by v=λf or
v=λ/T.

Review

When a guitar string is plucked, in what direction does the wave travel? In what directions does the string vibrate?
If the speed of a wave is 2.5 × 10³ m/s and has a wavelength of 5.80 × 10^-7 m, what is its frequency?
A wave has a frequency of 350 Hz has a wavelength of 3.9 m. What is the velocity of the wave?