14.5: Concave Mirrors
( \newcommand{\kernel}{\mathrm{null}\,}\)

Concave mirrors are used in a number of applications. They form upright, enlarged images, and are therefore useful in makeup application or shaving. They are also used in flashlights and headlights because they project parallel beams of light, and in telescopes because they focus light to produce greatly enlarged images.
The photograph above shows the grinding of the primary mirror in the Hubble space telescope. The Hubble Space Telescope is a reflecting telescope with a mirror approximately eight feet in diameter, and was deployed from the Space Shuttle Discovery on April 25, 1990.
Image in a Concave Mirror
Reflecting surfaces do not have to be flat. The most common curved mirrors are spherical. A spherical mirror is called a concave mirror if the center of the mirror is further from the viewer than the edges are. A spherical mirror is called a convex mirror if the center of the mirror is closer to the viewer than the edges are.
To see how a concave mirror forms an image, consider an object that is very far from the mirror so that the incoming rays are essentially parallel. For an object that is infinitely far away, the incoming rays would be exactly parallel. Each ray would strike the mirror and reflect according to the law of reflection (angle of reflection is equal to the angle of incidence). As long as the section of mirror is small compared to its radius of curvature, all the reflected rays will pass through a common point, called the focal point (f).

If too large a piece of the mirror is used, the rays reflected from the top and bottom edges of the mirror will not pass through the focal point and the image will be blurry. This flaw is called spherical aberration and can be avoided either by using very small pieces of the spherical mirror or by using parabolic mirrors.
A line drawn to the exact center of the mirror and perpendicular to the mirror at that point is called the principal axis. The distance along the principle axis from the mirror to the focal point is called the focal length. The focal length is also exactly one-half of the radius of curvature of the spherical mirror. That is, if the spherical mirror has a radius of 8 cm, then the focal length will be 4 cm.
Objects Outside the Center Point

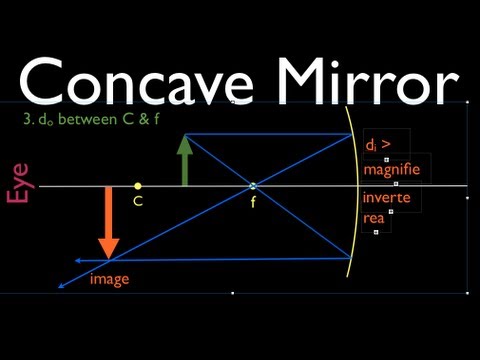
Above is a spherical mirror with the principle axis, the focal point, and the center of curvature (C) identified on the image. An object has been placed well beyond C, and we will treat this object as if it were infinitely far away. There are two rays of light leaving any point on the object that can be traced without any drawing tools or measuring devices. The first is a ray that leaves the object and strikes the mirror parallel to the principle axis that will reflect through the focal point. The second is a ray that leaves the object and strikes the mirror by passing through the focal point; this ray will reflect parallel to the principle axis.
These two rays can be seen in the image below. The two reflected rays intersect after reflection at a point between C and F. Since these two rays come from the tip of the object, they will form the tip of the image.

If the image is actually drawn in at the intersection of the two rays, it will be smaller and inverted, as shown below. Rays from every point on the object could be drawn so that every point could be located to draw the image. The result would be the same as shown here. This is true for all concave mirrors with the object outside C: the image will be between C and F, and the image will be inverted and diminished (smaller than the object).

The heights of the object and the image are related to their distances from the mirror. In fact, the ratio of their heights is the same ratio as their distances from the mirror. If do is object distance, di the image distance, ho the object height and hi the image height, then
ho/hi=do/di.
It can also be shown that do/di=(do−f)/f and from this, we can derive the mirror equation,
(1/do)+(1/di)=(1/f).
In this equation, f is the focal length do is the object distance, and di is the image distance.
The magnification equation for a mirror is the image size divided by the object size, where m gives the magnification of the image.
m=−di/do
Example 14.5.1
A 1.50 cm tall object is placed 20.0 cm from a concave mirror whose radius of curvature is 30.0 cm. What is the image distance and what is the image height?
Solution
(1/do)+(1/di)=(1/f) The focal length is one-half the center of curvature so it is 15.0 cm. (1/20.0)+(1/x)=(1/15) multiply both sides by 60x to get 3x+60=4x and x=60.0 cm.
The image distance is 60.0 cm. The image is 3 times as far from the mirror as the object so it will be 3 times as large, or 4.5 cm tall.
Objects Between the Center Point and the Focal Point
Regardless of where the object is, its image's size and location can be determined using the equations given earlier in this section. Nonetheless, patterns emerge in these characteristics. We already know that the image of an object outside the center point is closer and smaller than the object. When an object is between the center point and the focal point, the image is larger and closer. These characteristics can also be determined by drawing the rays coming off of the object; this is called a ray diagram.

Look again at the image above that was shown earlier in the lesson. If you consider the smaller arrow to be the object and follow the rays backward, the ray diagram makes it clear that if an object is located between the center point and focal point, the image is inverted, larger, and at a greater distance.
Example 14.5.2
If an 3.00 cm tall object is held 15.00 cm away from a concave mirror with a radius of 20.00 cm, describe its image.
Solution
To solve this problem, we must determine the height of the image and the distance from the mirror to the image. To find the distance, use the mirror equation:
(1/di)=(1/f)−(1/do)=(1/10)−(1/15)=(1/30)=30cm
Next determine the height of the image:
(ho/hi)=(do/di)=(3/hi)=(15/30)
Using this equation, we can determine that the height of the image is 6 cm.
This image is a real image, which means that the rays of light are real rays. These are represented in the ray diagram as solid lines, while virtual rays are dotted lines.
Objects Inside the Focal Point
Consider what happens when the object for a concave mirror is placed between the focal point and the mirror. This situation is sketched at below.

Once again, we can trace two rays to locate the image. A ray that originates at the focal point and passes through the tip of the object will reflect parallel to the principal axis. The second ray we trace is the ray that leaves the tip of the object and strikes the mirror parallel to the principal axis.
Below is the ray diagram for this situation. The rays are reflected from the mirror and as they leave the mirror, they diverge. These two rays will never come back together and so a real image is not possible. When an observer looks into the mirror, however, the eye will trace the rays backward as if they had followed a straight line. The dotted lines in the sketch show the lines the rays would have followed behind the mirror. The eye will see an image behind the mirror just as if the rays of light had originated behind the mirror. The image seen will be enlarged and upright. Since the light does not actually pass through this image position, the image is virtual.

Example 14.5.3
A 1.00 cm tall object is placed 10.0 cm from a concave mirror whose radius of curvature is 30.0 cm. Determine the image distance and image size.
Solution
Since the radius of curvature is 30.0 cm, the focal length is 15.0 cm. The object distance of 10.0 cm tells us that the object is between the focal point and the mirror.
(1/do)+(1/di)=(1/f) and plugging known values yields (1/10.0)+(1/x)=(1/15)
Multiplying both sides of the equation by 30x yields 3x+30=2x and x=−30.0 cm.
The negative image distance indicates that the image is behind the mirror. We know the image is virtual because it is behind the mirror. Since the image is 3 times as far from the mirror as the object, it will be 3 times as tall. Therefore, the image height is 3.00 cm.
A Cassegrain telescope uses a concave primary mirror to magnify light from very distant objects to form an image we can see. Play around with a Cassegrain telescope in the simulation below and see if you can until you get a clear view of Jupiter in the eyepiece:
Interactive Element
Summary
- A spherical mirror is concave if the center of the mirror is further from the viewer than the edges.
- For an object that is infinitely far away, incoming rays would be exactly parallel.
- As long as the section of mirror is small compared to its radius of curvature, all the reflected rays will pass through a common point, called the focal point.
- The distance along the principle axis from the mirror to the focal point is called the focal length, f. This is also exactly one-half of the radius of curvature.
- The mirror equation is (1/do)+(1/di)=1/f.
- The magnification equation is m=−di/do.
- For concave mirrors, when the object is outside C, the image will be between C and F and the image will be inverted and diminished (smaller than the object).
- For concave mirrors, when the object is between C and F, the image will be beyond C and will be enlarged and inverted.
- For concave mirrors, when the object is between F and the mirror, the image will be behind the mirror and will be enlarged and upright.
Review
- A concave mirror is designed so that a person 20.0 cm in front of the mirror sees an upright image magnified by a factor of two. What is the radius of curvature of this mirror?
- If you have a concave mirror whose focal length is 100.0 cm, and you want an image that is upright and 10.0 times as tall as the object, where should you place the object?
- A concave mirror has a radius of curvature of 20.0 cm. Locate the image for an object distance of 40.0 cm. Indicate whether the image is real or virtual, enlarged or diminished, and upright or inverted.
- A dentist uses a concave mirror to examine a tooth that is 1.00 cm in front of the mirror. The image of the tooth forms 10.0 cm behind the mirror.
- What is the mirror’s radius of curvature?
- What is the magnification of the image?
- When a man stands 1.52 m in front of a shaving mirror, the image produced is inverted and has an image distance of 18.0 cm. How close to the mirror must the man place his face if he wants an upright image with a magnification of 2.0?
Explore More
Use this resource to answer the questions that follow.
- What are the two main rays you need to draw a real image?
- What is the third ray? What can you use it for?
- How do light rays through the focal point and vertex behave the same? How are they different?
Additional Resources
Study Guide: Geometric Optics Study Guide
Real World Application: Distorted Images
Video: