3.5.3: Simple and Compound Interest

Last updated
Save as PDF

Page ID: 14383

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

Simple and Compound Interest

Suppose you are re-negotiating an allowance with your parents. Currently you are given $25 per week, but it is the first of June, and you have started mowing the lawn and taking out the trash every week, and you think your allowance should be increased.

Your father considers the situation and makes you the following offer:

"I tell you what, son. I will give you three options for your allowance, you tell me which you would like"

"Option A: You keep the $25 per week"

"Option B: You take $15 this week, then $16 next week, and so on. I'll continue adding $1 per week until New Year's."

"Option C: I'll give you 1 penny this week, and then double your allowance each week until the first of October, then keep it at that rate."

Which option would you choose?

Simple and Compound Interest

Simple interest is interest which accrues based only on the principal of an investment or loan. The simple interest is calculated as a percent of the principal.

Simple Interest: i=p⋅r⋅t.

Variable i is interest, p represents the principal amount, r represents the interest rate, and t represents the amount of time the interest has been accruing. For example, say you borrow $2,000 from a family member, and you insist on repaying with interest. You agree to pay 5% interest, and to pay the money back in 3 years.

The interest you will owe will be 2000(0.05)(3) = $300. This means that when you repay your loan, you will pay $2300. Note that the interest you pay after 3 years is not 5% of the original loan, but 15%, as you paid 5% of $2000 each year for 3 years.

Now let’s consider an example in which interest is compounded. Say that you invest $2000 in a bank account, and it earns 5% interest annually. How much is in the account after 3 years?

Compound interest: A(t)=p⋅(1+r)^t

Here, A(t) is the Amount in the account after a given time in years, principal is the initial investment, and rate is the interest rate. Note that we use (1+r) instead of just r, so we can find the entire amount in the account, not just the interest paid.

A(t)=2000⋅(1.05)³

After three years, you will have $2315.25 in the account, which means that you will have earned $315.25 in interest.

Compounding results in more interest because the principal on which the interest is calculated increased each year. Another way to look at it is that compounding creates more interest because you are earning interest on interest, and not just on the principal.

Examples

Example 1

Earlier, you were asked which allowance option you would choose.

Solution

Assume you want to make the most money possible by the end of the year. Assume also that there are 24 weeks left.

Option A = 25⋅24=$600 total

Option B = 15+16+17...+39=$609 total

Option C (assuming 16 weeks until Oct.) = 1⋅(2¹⁶)=$655.36 each week after Oct 1.

It is entirely possible that dear old dad didn't take exponential growth seriously enough, he may need a second job!

Example 2

Use the formula for compound interest to determine the amount of money in an investment after 20 years, if you invest $2000, and the interest rate is 5% compounded annually.

Solution

The investment will be worth $5306.60.

A(t) = P(1 + r)^t

A(20) = 2000(1.05)²⁰

A(20) = $5306.60

Example 3

How long will it take for $2000, invested at 5% compounded annually, to reach $7,000?

Solution

If we graph the function A(t) = 2000(1.05)^t, we can see the values for any number of years.

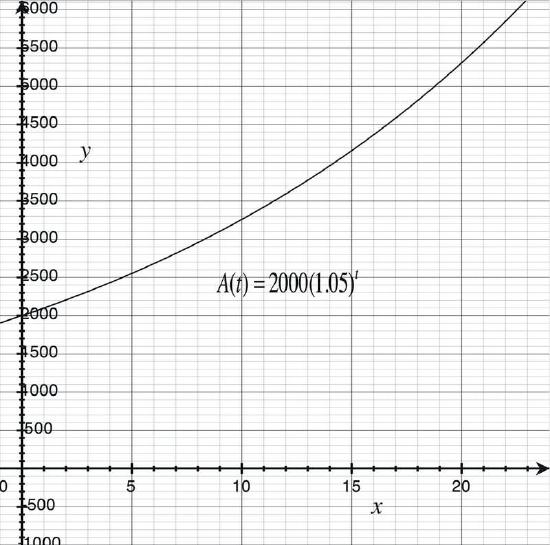
f-d_a23602657452017baf351aa4df822f1860746c3d7780daeef299368a+IMAGE_TINY+IMAGE_TINY.jpg

If you graph this function using a graphing calculator, you can determine the value of the investment by tracing along the function, or by pressing <TRACE> on your graphing calculator and then entering an x value.

You can also choose an investment value you would like to reach, and then determine the number of years it would take to reach that amount. Find the intersection of the exponential function with the line y = 7000.

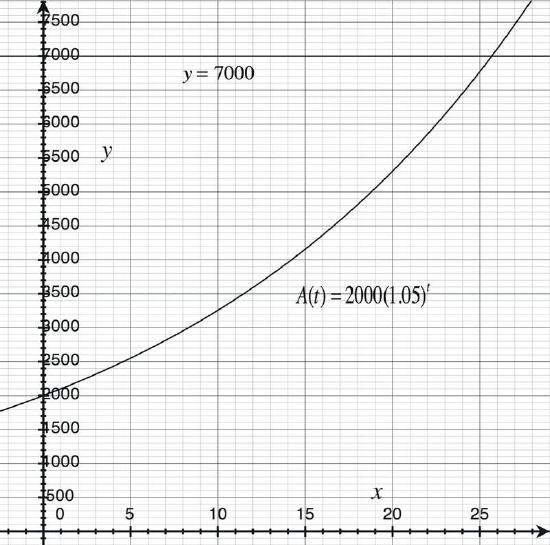
f-d_60e70477d28bbfb91d02e3009beb7ad00e364aaa5b784a2bb761e637+IMAGE_TINY+IMAGE_TINY.jpg

You can see here that the line and the curve intersect at a little less than x = 26. Therefore it would take almost 26 years for the investment to reach $7000.

Example 4

What is the value of an investment after 20 years, if you invest $2000, and the interest rate is 5% compounded continuously?

Solution

The more often interest is compounded, the more it increases, but there is a limit. Each time you increase the number of compoundings, you decrease the fraction of the annual interest that is applied to each compounding. Eventually, the differences become so small as to be negligible. This is known as continuous compounding.

The function A(t) = Pe^rt is the formula we use to calculate the amount of money when interest is continuously compounded, rather than interest that is compounded at discrete intervals, such as monthly or quarterly.

A(t) = Pe^rt

A(20) = 2000e^.05(20)

A(20) = 2000e¹

A(20) = $5436.56

Example 5

Compare the values of the investments shown in the table. If everything else is held constant, how does the compounding influence the value of the investment?

	Principal	r	n	t
a.	$4,000	.05	1 (annual)	8
b.	$4,000	.05	4 (quarterly)	8
c.	$4,000	.05	12 (monthly)	8
d.	$4,000	.05	365 (daily)	8
e.	$4,000	.05	8760 (hourly)	8

Solution

Use the compound interest formula. For this example, the n is the quantity that changes:

$\ A(8)=4000\left(1+\frac{.05}{n}\right)^{8 n}$

	Principal	r	n	t	A
a.	$4,000	.05	1 (annual)	8	$5909.82
b.	$4,000	.05	4 (quarterly)	8	$5952.52
c.	$4,000	.05	12 (monthly)	8	$5962.34
d.	$4,000	.05	365 (daily)	8	$5967.14
e.	$4,000	.05	8760 (hourly)	8	$5967.29

A graph of the function $\ f(x)=4000\left(1+\frac{.05}{x}\right)^{8 x}$ is shown below:

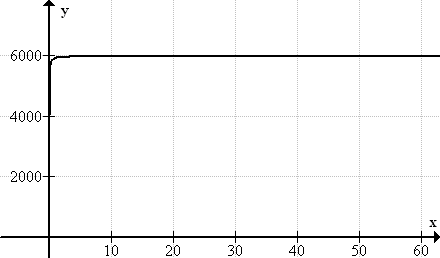
f-d_ca6f57a2a507871152cdb17706dfeff541cf792e949f6ec755207ae1+IMAGE_TINY+IMAGE_TINY.png

The graph seems to indicate that the function has a horizontal asymptote at $6000. However, if we zoom in, we can see that the horizontal asymptote is closer to 5967.

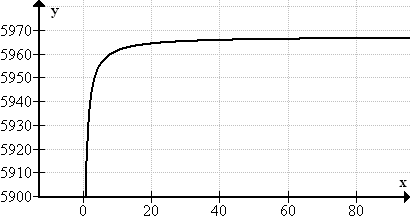f-d_1cc29873fce763dbe22a7504fb82c895e88ef6019207d0eb0468ec0f+IMAGE_TINY+IMAGE_TINY.png

What does this mean? This means that for the investment of $4000, at 5% interest, for 8 years, compounding more and more frequently will never result in more than about $5968.00.

Example 6

Determine the value of each investment.

You invest $5000 in an account that gives 6% interest, compounded monthly. How much money do you have after 10 years?
You invest $10,000 in an account that gives 2.5% interest, compounded quarterly. How much money do you have after 10 years?

Solution

$5000, invested for 10 years at 6% interest, compounded monthly.
$\ A(t)=P\left(1+\frac{r}{n}\right)^{n t}$

$\ A(10)=5000\left(1+\frac{.06}{12}\right)^{12 \cdot 10}$

$\ A(10)=5000(1.005)^{120}$

A(10)=$9096.98
$10000, invested for 10 years at 2.5% interest, compounded quarterly.
Quarterly compounding means that interest is compounded four times per year. So in the equation, n = 4.

$\ A(t)=P\left(1+\frac{r}{n}\right)^{n t}$

$\ A(10)=6000\left(1+\frac{.025}{4}\right)^{4 \cdot 10}$

$\ A(10)=6000(1.00625)^{40}$

$\ A(10)=$12,830.30$

In each example, the value of the investment after 10 years depends on three quantities: the principal of the investment, the number of compoundings per year, and the interest rate.

Example 7

How long will it take $2000 to grow to $25,000 at a 5% interest rate?

Solution

It will take about 50 years:

A(t) = Pe^rt
25,000 = 2000e ^.05(t)
12.5 = e ^.05(t)	Divide both sides by 2000
ln 12.5 = ln e ^.05(^t⁾	Take the ln of both sides
ln 12.5 = .05t ln e	Use the power property of logs
ln 12.5 = .05t × 1	ln e = 1
ln 12.5 = 0.5t	Isolate t
$\ t=\frac{\ln 12.5}{.05} \approx 50.5$

Review

What is the formula for figuring simple interest?
What is the formula for figuring compound interest?
If someone invested $4500.00, how much would they have earned after 4 years, at a simple interest rate of 2%?
Kyle opened up a savings account in July. He deposited $900.00. The bank pays a simple interest rate of 5% annually. What is Kyle's balance at the end of 4 years?
After having an account for 6 years, how much money does Roberta have in the account, if her original deposit was $11,000, and her bank's yearly simple interest rate is 8.4%?
Tom called his bank today to check on his savings account balance. he was surprised to find a balance of $6600, when he started the account with just $5000.00 8 years ago. Based on this data, what percentage rate has the bank been paying on the account?
Julie opened a 4% interest account with a bank that compounds the interest quarterly. If Julie were to deposit $3000.00 into the account at the beginning of the year, how much could she expect to have at the end of the year?
Susan has had a saving account for a few years now. The bank has been paying her simple interest at a rate of 5%. She has earned $45.00 on her initial deposit of $300.00. How long has she had the account?
What is the balance on a deposit of $818.00 earning 5% interest compounded semiannually for 5 years?
Karen made a decent investment. After 4 years she had $3250.00 in her account and expects to have $16,250, after another 4 years. Her savings account is a compounding interest account. How much was her original deposit?
What is the yearly simple interest rate that Ken earns, if after only three months he earned $16.00 on an initial $800.00 deposit?
Write an expression that correctly represents the balance on an account after 7 years, if the account was compounded yearly at a rate of 5%, with an initial balance of $1000.00
Caryl gives each of his three kids $3000.00 each, and they each use it to open up saving accounts at three different banks. Georgia, his oldest, is earning 3% annually at her bank. Kirk earns 7% annually at his bank. Lottie's bank is paying her an annual rate of 4%. At the end of 6 years show much will each of them have in their respective accounts.
Kathy receives an inheritance check for $3000.00 and decides to put it in a saving account so she can send her daughter to college when she gets older. After looking she finds an account that pays compounding interest annually at a rate of $14%. The balance on the account can be represented by a function, where x is the time in years. Write a function, and then use it to determine how much will be in the account at the end of 7 years.
Stan is late on his car payment. The finance company charges 3% interest per month it is late. His monthly payment is $300.00. What is the total amount he will owe if he pays the August first bill October first? (assuming he was able to make his September bill on - time)

Today, you get your first credit card. It charges 12.49% interest on all purchases and compounds that interest monthly. Within one day you max out the credit limit of $1,200.00.

If you pay the monthly accrued interest plus $50.00 towards the initial $1,200 amount every month, how much will you still owe at the end of the first 12 months?
How much will you have paid in total at the end of the year?

You are preparing for retirement. You invest $10,000 for 5 years, in an account that compounds monthly at 12% per year. However, unless this money is in an IRA or other tax-free vehicle, with zero inflation, you also have an annual tax payment of 30% on the earned interest.

How much will you have in 5 years?
Now take into account that the money loses 3% spending value per year due to inflation, how much is what you have saved really worth at the end of the 5 years?

Review (Answers)

To see the Review answers, open this PDF file and look for section 3.11.

Vocabulary

Term	Definition
Accrue	Accrue means "increase in amount or value over time." If interest accrues on a bank account, you will have more money in your account. If interest accrues on a loan, you will owe more money to your lender.
Compound interest	Compound interest refers to interest earned on the total amount at the time it is compounded, including previously earned interest.
Continuous compounding	Continuous compounding refers to a loan or investment with interest that is compounded constantly, rather than on a specific schedule. It is equivalent to infinitely many but infinitely small compounding periods.
Principal	The principal is the amount of the original loan or original deposit.
Rate	The rate is the percentage at which interest accrues.

Image Attributions

[Figure 1]
Credit: CK-12
Source: CK-12
[Figure 2]
Credit: CK-12
Source: CK-12