6.1.2: Equation of an Ellipse

Equation of an Ellipse

Ken is having a disagreement with his friend Scott. Scott says that the track that they run on at school is not really an ellipse, but an oval. Ken thinks that is a silly distinction, since ovals and ellipses are the same thing. Who is correct?

---

Equation of an Ellipse

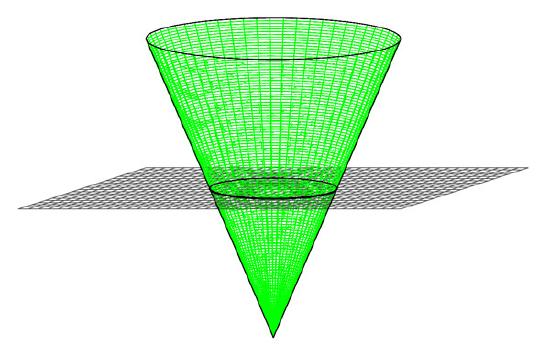
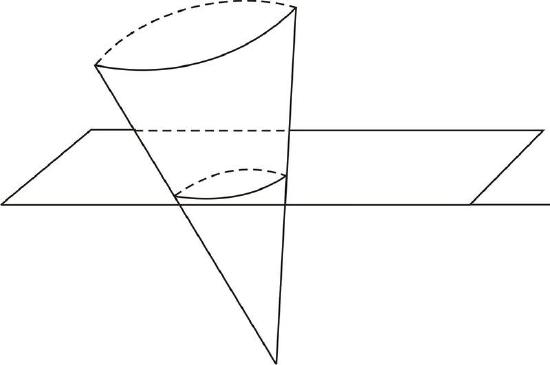
When a plane "slices through" one side of a cone, we get either a circle or an “oval-shaped” object as shown below. It turns out that this is no ordinary oval, but something called an ellipse, a shape with special properties.

a. Cone "sliced through" by a plane 90^o to the axis of the cone to form a circle.		b. Cone "sliced through" by a plane at an angle to form an ellipse.

Ellipses can be defined by some of their properties. One way to define an ellipse is as a “stretched out circle”. It’s the shape you would get if you sketched a circle on a deflated balloon and then stretched out the balloon evenly in two opposite directions:

It’s also the shape of the surface of water that results when you tilt a round glass:

Or an ellipse could be thought of as the shape of a circle drawn on a piece of paper when it is viewed at an angle.

Equations of Ellipses

This “stretching” can be represented algebraically. For simplicity, take the circle of radius 1 centered at the origin (0,0). The distance formula tells us that this is the set of points (x,y) that is a distance 1 unit away from the origin.

\(\ \begin{aligned}
D &=\sqrt{\left(x_{1}-x_{2}\right)^{2}+\left(y_{1}-y_{2}\right)^{2}} \\
1 &=\sqrt{(x-0)^{2}+(y-0)^{2}} \\
1 &=x^{2}+y^{2}
\end{aligned}\)

This equation, \(\ x^{2}+y^{2}=1\), can be altered to stretch the circle in the horizontal (i.e. x−axis) direction by dividing the \(\ x\) variable by a constant \(\ a>1\),

\(\ \left(\frac{x}{a}\right)^{2}+y^{2}=1\)

Why does this stretch the circle horizontally? Well, the effect of dividing \(\ x\) by \(\ a\) is that for each \(\ y\)−value in an ordered pair \(\ (x, y)\) that satisfies the original equation, the corresponding \(\ x\) value must be multiplied by \(\ a\) in order for the pair to make a solution to the altered equation. So solutions \(\ (x, y)\) of the circle are in one-to-one correspondence with solutions \(\ (ax, y)\) of the altered equation, hence stretching the corresponding graph to the left and right by a factor of \(\ a\).

Here is the graph of \(\ \left(\frac{x}{2}\right)^{2}+y^{2}=1\):

Generalizing the equation by allowing a stretch in the vertical direction, we get the following.

\(\ \left(\frac{x}{a}\right)^{2}+\left(\frac{y}{b}\right)^{2}=1\)

The factor \(\ a\) stretches the circle in the horizontal direction and the factor \(\ b\) stretches the circle in the vertical direction. If \(\ a=b\), this is just a circle. When \(\ a \neq b\), this equation represents an ellipse. The ellipse is stretched in the horizontal direction if \(\ b<a\) and it is stretched in the vertical direction if \(\ a<b\).

Often the above equation is written as follows.

\(\ \frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1\)

This is called the standard form of the equation of an ellipse, assuming that the ellipse is centered at (0, 0).

To sketch a graph of an ellipse with the equation \(\ \frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1\), start by plotting the four axes intercepts, which are easy to find by plugging in 0 for x and then for y. Then sketch the ellipse freehand, or with a graphing program or calculator.

The Foci

In every ellipse there are two special points called the foci (foci is plural, focus is singular), which lie inside the ellipse and which can be used to define the shape. For an ellipse centered at (0,0) that is wider than it is tall, its major axis is horizontal and its foci are at \(\ \left(\sqrt{a^{2}-b^{2}}, 0\right)\) and \(\ \left(-\sqrt{a^{2}-b^{2}}, 0\right)\).

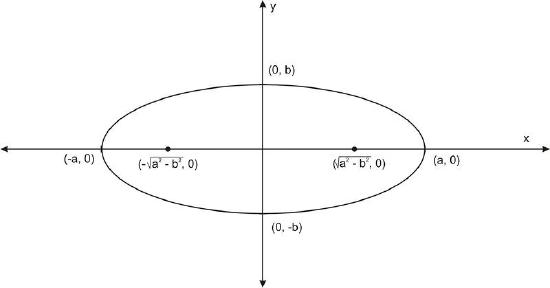
What is the significance of these points? Ellipses relate to these points much as a circle relates to its center. Remember a circle can be thought of as the set of points in a plane that are a certain distance from the center point, in fact, that is typically the definition of a circle. Well, the foci act like the center except that there are two of them. An ellipse is the set of points where the sum of the distance between each point on the ellipse and each of the two foci is a constant number. In the diagram below, for any point \(\ P\) on the ellipse, \(\ F_{1} P+F_{2} P=d\), where \(\ F_{1}\) and \(\ F_{2}\) are the foci and \(\ d\) is a constant.

---

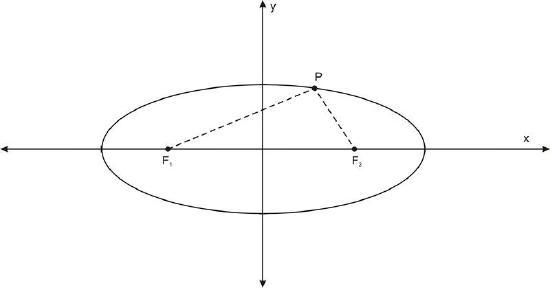
Examples

Example 1

Earlier, you were given a question about Ken and Scott's disagreement.

Solution

Scott is correct. Ovals are not the same as ellipses.

Example 2

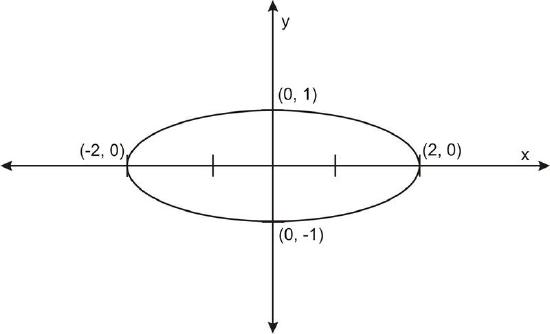
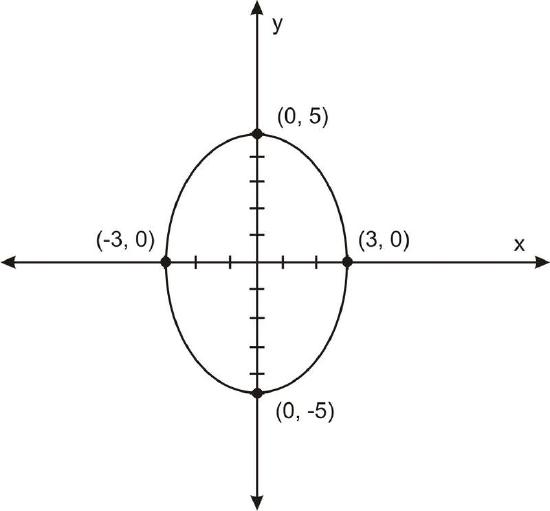
Sketch the graph of \(\ \frac{x^{2}}{4}+\frac{y^{2}}{9}=1\).

Solution

This equation can be rewritten as \(\ \frac{x^{2}}{2^{2}}+\frac{y^{2}}{3^{2}}=1\).

To sketch, we set \(\ x=0\) and solve for \(\ y\) to find the \(\ x\)-intercepts and set \(\ y=0\) and solve for \(\ x\) to find the \(\ y\)− intercepts.

The four points we get are (0, 3), (0, -3), (2, 0), and (-2, 0).

Plot those points and sketch the ellipse:

Example 3

Sketch the graph of \(\ \frac{x^{2}}{16}+y^{2}=1\).

Solution

This can be rewritten as \(\ \frac{x^{2}}{4^{2}}+\frac{y^{2}}{1^{2}}=1\). After finding the intercepts and sketching the graph with the same process as above, we have:

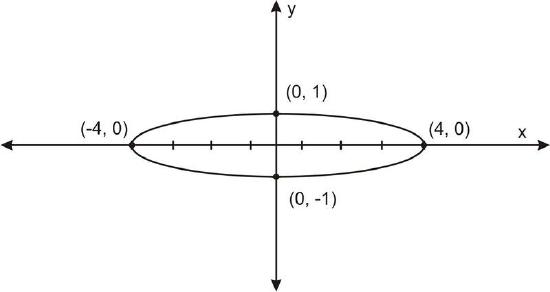
The segment spanning the long direction of the ellipse is called the major axis, and the segment spanning the short direction of the ellipse is called the minor axis. So in the last example the major axis is the segment from (-4, 0) to (4, 0) and the minor axis is the segment from (0, -1) to (0, 1).

The major and minor axes are examples of what are sometimes called reference lines. Apollonius, the Ancient Greek mathematician who wrote an early treatise on conics, used these and other reference lines to orient conic sections. Though the Greeks did not use a coordinate plane to discuss geometry, these reference lines offer a framing perspective that is similar to the Cartesian plane that we use today. Apollonius’ way of framing conics with reference lines was the closest mathematics came to the system of coordinate geometry that you know so well until Descartes’ and Fermat’s systematic work in the seventeenth century.

Example 4

What is the full range of the eccentricity of an ellipse? What does it look like near the extremes of this range?

Solution

Foci can be used to measure how far an ellipse is “stretched” from a circle. The symbol \(\ \varepsilon\) stands for the eccentricity of an ellipse, and it is defined by the distance between the foci divided by the length of the major axis, or \(\ \frac{\sqrt{a^{2}-b^{2}}}{a}\) for horizontally oriented ellipses and \(\ \frac{\sqrt{b^{2}-a^{2}}}{b}\) for vertically oriented ellipses. Since a circle is an ellipse where \(\ a=b\), circles have an eccentricity of 0.

The interval of possible values is \(\ \varepsilon \in[0,1)\). At \(\ \varepsilon=0\), the ellipse is a circle; as the eccentricity approaches 1 it becomes more and more elongated.

Example 5

Not all equations for ellipses start off in the standard form above. For example, \(\ 25 x^{2}+9 y^{2}=225\) is an ellipse. Put it in the proper form and graph it.

Solution

To graph \(\ 25 x^{2}+9 y^{2}=225\) as an ellipse:

\(\ \frac{x^{2}}{9}+\frac{y^{2}}{25}=1\)..... First, divide both sides by 225

\(\ \frac{x^{2}}{3^{2}}+\frac{y^{2}}{5^{2}}=1\)..... Find the intercepts

Graphing, we have:

Example 6

It was mentioned above that when a round glass of water is tilted, the surface of the water is an ellipse. Using our working definition of an ellipse as “stretched out circle”, explain why you think the water takes this shape.

Solution

Answers may vary, but should explain why the shape that results stretches a circle in one direction because the width of the glass is constant.

Example 7

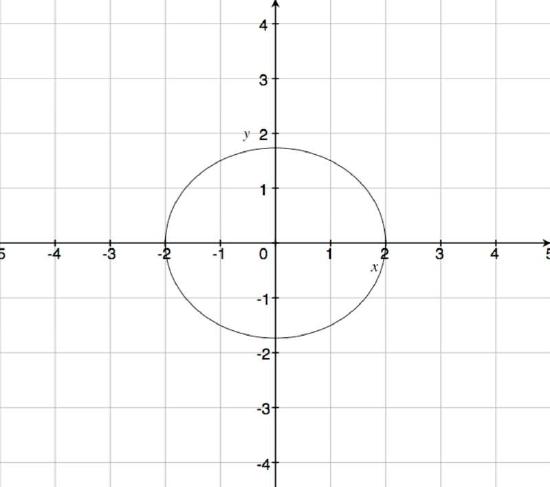
Try to sketch this ellipse where the numbers don’t turn out to be so neat: \(\ 3 x^{2}+4 y^{2}=12\).

Solution

To graph \(\ 3 x^{2}+4 y^{2}=12\):

\(\ \frac{x^{2}}{4}+\frac{y^{2}}{3}=1\)..... First, divide both sides by 12

\(\ \frac{x^{2}}{2^{2}}+\frac{y^{2}}{\sqrt{3}^{2}}=1\)..... Find the intercepts

Graphing, we get:

---

Review

Convert each equation to the standard form of an ellipse.

1. \(\ x^{2}+4 y^{2}=4\)
2. \(\ 9 x^{2}+16 y^{2}=144\)
3. \(\ 25 x^{2}+36 y^{2}=900\)
4. \(\ 36 x^{2}+9 y^{2}=324\)
5. \(\ 49 x^{2}+y^{2}=49\)
6. \(\ 16 x^{2}+4 y^{2}=64\)
7. \(\ x^{2}+2 y^{2}=2\)
8. \(\ 3 x^{2}+4 y^{2}=12\)
9. \(\ 5 x^{2}+6 y^{2}=30\)
10. \(\ 6 x^{2}+3 y^{2}=18\)
11. \(\ 7 x^{2}+y^{2}=7\)
12. \(\ 4 x^{2}+2 y^{2}=8\)

Graph the following ellipses.

13. \(\ 9 x^{2}+16 y^{2}=144\)
14. \(\ 49 x^{2}+y^{2}=49\)
15. \(\ x^{2}+2 y^{2}=2\)
16. \(\ 7 x^{2}+y^{2}=7\)

---

Review (Answers)

To see the Review answers, open this PDF file and look for section 6.1.

---

Vocabulary

Term	Definition
Cone	A cone is a solid three-dimensional figure with a circular base and one vertex.
Conic	Conic sections are those curves that can be created by the intersection of a double cone and a plane. They include circles, ellipses, parabolas, and hyperbolas.
Eccentricity	The eccentricity of a conic section is a measure of how much the conic section deviates from being circular. The eccentricity of circles is 0, the eccentricity of ellipses is between 0 and 1, the eccentricity of parabolas is 1, and the eccentricity of hyperbolas is greater than 1. For ellipses and hyperbolas, \(\ e=\frac{c}{a}\).
Ellipse	Ellipses are conic sections that look like elongated circles. An ellipse represents all locations in two dimensions that are the same distance from two specified points called foci.
Ellipses	Ellipses are conic sections that look like elongated circles. An ellipse represents all locations in two dimensions that are the same distance from two specified points called foci.
Foci	The foci of an ellipse are the two points that define the ellipse. The sum of the distances from any point on the ellipse to the foci is constant.
Major Axis	The major axis of an ellipse is the longest diameter of the ellipse.
minor axis	The minor axis of an ellipse is the shortest diameter of the ellipse.
standard form	The standard form of an ellipse centered at \(\ (h, k)\) is \(\ \frac{(x-h)^{2}}{a^{2}}+\frac{(y-k)^{2}}{b^{2}}=1\).