1.5: Evaluate Limits Using Substitution

Last updated
Save as PDF

Page ID: 5676

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Finding limits for the vast majority of points for a given function is as simple as substituting the number that x approaches into the function. Since this turns evaluating limits into an algebra-level substitution, most questions involving limits focus on the cases where substituting does not work. How can you decide if substitution is an appropriate analytical tool for finding a limit?

Using Substitution to Find Limits

Finding a limit analytically means finding the limit using algebraic means. In order to evaluate many limits, you can substitute the value that x approaches into the function and evaluate the result. This works perfectly when there are no holes or asymptotes at that particular x value. You can be confident this method works as long as you don’t end up dividing by zero when you substitute.

If the function f(x) has no holes or asymptote at x=a then:

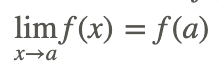
Screen Shot 2020-08-19 at 6.19.34 PM.png

Occasionally there will be a hole at x=a. The limit in this case is the height of the function if the hole did not exist. In other words, if the function is a rational expression with factors that can be canceled, cancel the term algebraically and then substitute into the resulting expression. If no factors can be canceled, it could be that the limit does not exist at that point due to asymptotes.

Look at the following two limits:

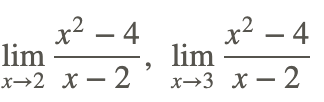
Screen Shot 2020-08-19 at 6.21.59 PM.png

The limit on the left cannot be evaluated by direct substitution because if 2 is substituted in, then you end up dividing by zero.The limit on the right can be evaluated using direct substitution because the hole exists at x=2 not x=3. Thus, the limit is:

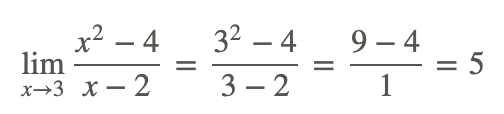
Screen Shot 2020-08-19 at 6.22.22 PM.png

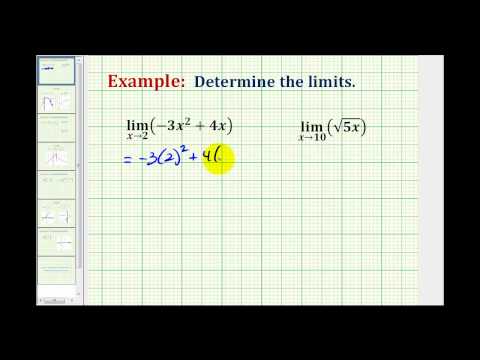
Examples

Example 1

Earlier, you were asked how to determine if you should use substitution to solve a limit. In order to decide whether substitution is an appropriate first step you can always just try it. You’ll know it won’t work if you end up trying to evaluate an expression with a denominator equal to zero. If this happens, go back and try to factor and cancel, and then try substituting again.

Example 2

Evaluate the following limit by canceling first and then using substitution.

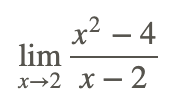
Screen Shot 2020-08-19 at 6.34.15 PM.png

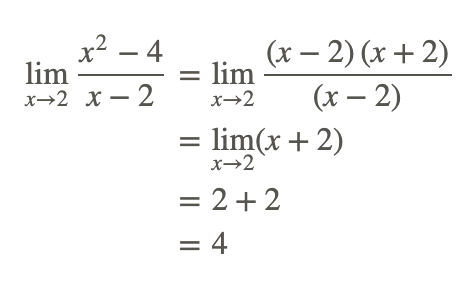
Screen Shot 2020-08-24 at 7.54.15 AM.png

Example 3

Evaluate the following limit analytically:

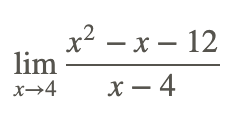
Screen Shot 2020-08-24 at 7.55.46 AM.png

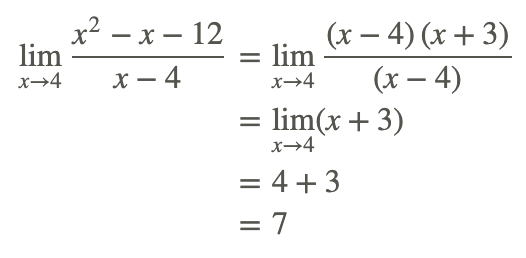
Screen Shot 2020-08-24 at 7.56.38 AM.png

Example 4

Evaluate the following limit analytically.

Screen Shot 2020-08-24 at 7.57.26 AM.png

Example 5

Evaluate the following limit analytically.

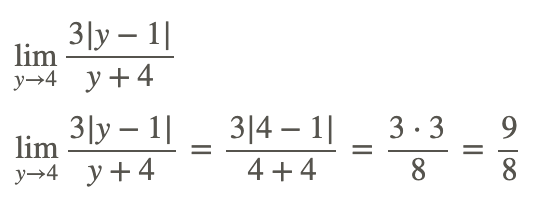
Screen Shot 2020-08-24 at 7.59.43 AM.png

Review

Evaluate the following limits analytically.

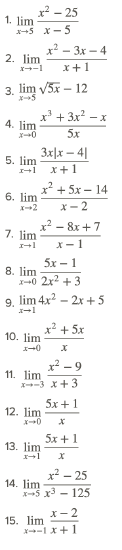
Screen Shot 2020-08-24 at 8.09.29 AM.png

Review (Answers)

To see the Review answers, open this PDF file and look for section 14.4.

Vocabulary

Term	Definition
analytically	One way to evaluate a limit analytically is by substitution. Substitution is a method of determining limits where the value that x is approaching is substituted into the function and the result is evaluated.
Asymptotes	An asymptote is a line on the graph of a function representing a value toward which the function may approach, but does not reach (with certain exceptions).
Hole	A hole exists on the graph of a rational function at any input value that causes both the numerator and denominator of the function to be equal to zero.
limit	A limit is the value that the output of a function approaches as the input of the function approaches a given value.

Additional Resources

Practice: Evaluate Limits Using Substitution

Real World: The Limits of Tolerance