1.6: Properties of Limit
- Page ID
- 5677
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\(\newcommand{\longvect}{\overrightarrow}\)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\ket}[1]{\left| #1 \right>}\)
\(\newcommand{\bra}[1]{\left< #1 \right|}\)
\(\newcommand{\braket}[2]{\left< #1 \vphantom{#2} \right| \left. #2 \vphantom{#1} \right>}\)
\(\newcommand{\braopket}[3]{\left< #1 \vphantom{#2}\vphantom{#3} \right| #2 \vphantom{#1}\vphantom{#3} \left| #3 \vphantom{#1}\vphantom{#2} \right>}\)
\(\newcommand{\qmvec}[1]{\mathbf{\vec{#1}}}\)
\(\newcommand{\op}[1]{\hat{\mathbf{#1}}}\)
\(\newcommand{\expect}[1]{\langle #1 \rangle}\)
\(\newcommand{\dfn}[1]{\emph{\textbf{#1}}}\)
You're familiar with the idea of a limit of a function, and that some limits are computed using numerical and graphical methods. Limits can also be evaluated using the properties of limits. How would you find
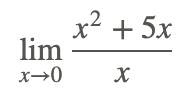
without using a graph or using a table of values?
Properties of Limits
Let’s begin with some observations about limits of some simple functions. Consider the following limit problems:
We note that each of these functions is defined for all real numbers. If we apply our intuition for finding the limits we conclude correctly that:
The above results can be encapsulated in the following limit properties:
Basic Limit Properties:
Many functions can be expressed as the sums, differences, products, quotients, powers and roots of other more simple functions. The following properties are also useful in evaluating limits:
More Basic Limit Properties:
Knowing these properties, allows evaluation of the limits of a wide range of functions.
Take the problem:
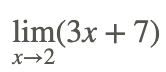
Based on the properties above, the limit can be evaluated in the following steps:
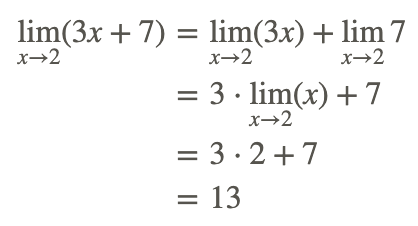
Therefore:
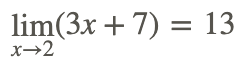
Note that the application of the basic limit properties results, in this case, in a limit value that is the same as direct substitution of x=2 in the function.
Now, evaluate
Based on the properties of limits, the limit can be evaluated in the following steps:
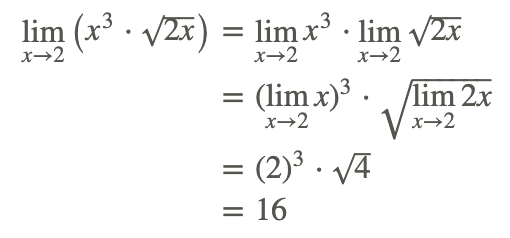
Therefore:
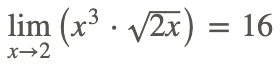
Again, note that the application of the basic limit properties results, in this case, in a limit value that is the same as direct substitution of x=2 in the function.
Examples
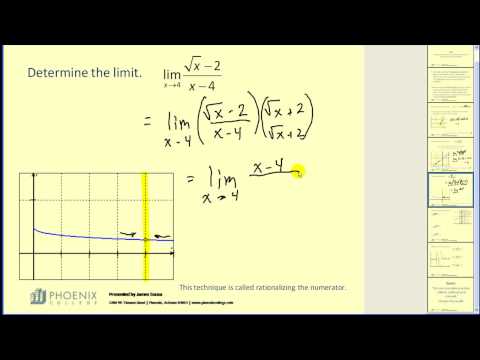
Example 1
Earlier, you were asked to find
without using a graph or a table of values. This is a case where direct substitution to evaluate the limit gives the indeterminate form 0/0. But, reducing the fraction and then applying the basic limit properties above, we can evaluate the limit:
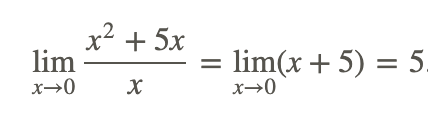
Example 2
Evaluate
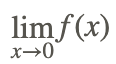
where f(x) is the rational function
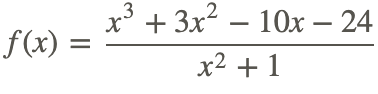
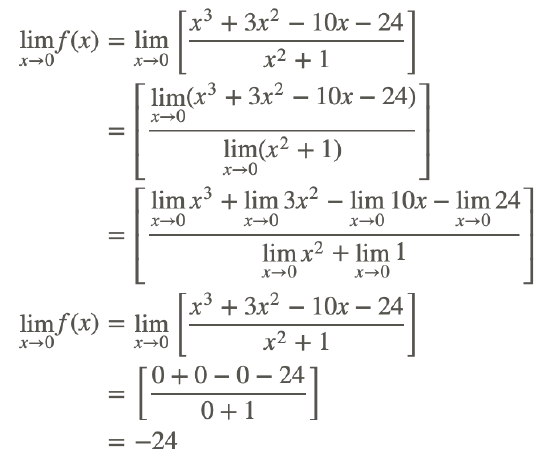
Example 3
Find the following limit if it exists:
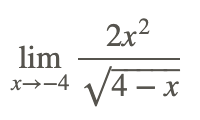
Let’s apply the basic quotient rule to evaluate this limit.
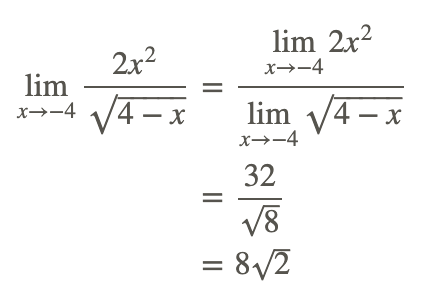
Therefore:
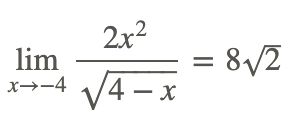
Again, the limit result is the same as using direct substitution of x=−4 in the function.
Review
Vocabulary
| Term | Definition |
|---|---|
| indeterminate | In mathematics, an expression is indeterminate if it is not precisely defined. There are seven indeterminate forms: 0/0,0⋅∞,∞∞,∞−∞,00,∞0, and 1∞. |
| limit | A limit is the value that the output of a function approaches as the input of the function approaches a given value. |
Additional Resources
Practice: Properties of Limits