6.18: Tangent Lines
( \newcommand{\kernel}{\mathrm{null}\,}\)
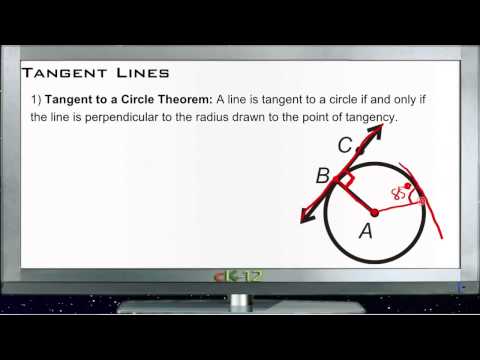
Lines perpendicular to the radius drawn to the point of tangency.
Tangent Line Theorems
There are two important theorems about tangent lines.
1. Tangent to a Circle Theorem: A line is tangent to a circle if and only if the line is perpendicular to the radius drawn to the point of tangency.
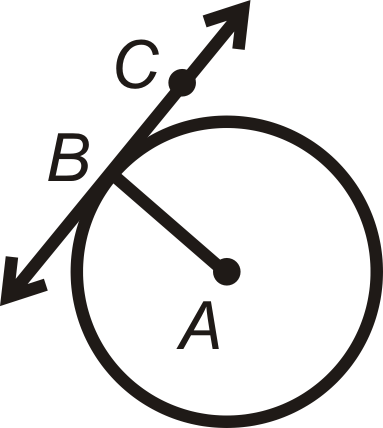
↔BC is tangent at point B if and only if ↔BC⊥¯AB.
This theorem uses the words “if and only if,” making it a biconditional statement, which means the converse of this theorem is also true.
2. Two Tangents Theorem: If two tangent segments are drawn to one circle from the same external point, then they are congruent.
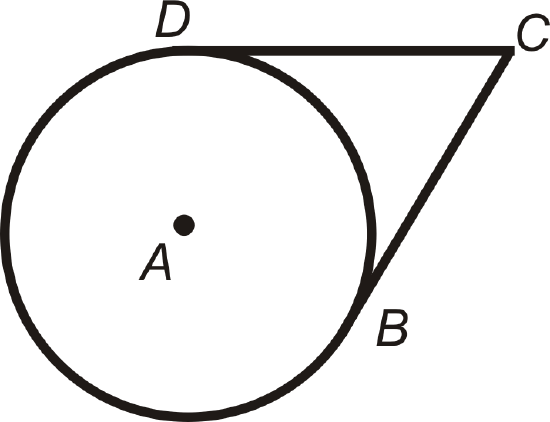
¯BC and ¯DC have C as an endpoint and are tangent; ¯BC≅¯DC.
What if a line were drawn outside a circle that appeared to touch the circle at only one point? How could you determine if that line were actually a tangent?
Example 6.18.1
Determine if the triangle below is a right triangle.
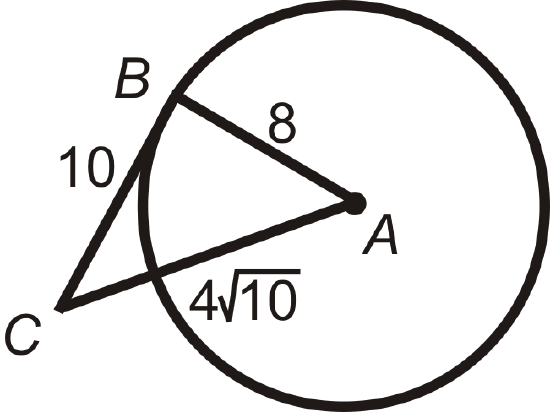
Solution
Use the Pythagorean Theorem. 4√10 is the longest side, so it will be c.
Does
82+102=(4√10)2?64+100≠160
ΔABC is not a right triangle. From this, we also find that ¯CB is not tangent to ⨀A.
Example 6.18.2
If D and C are the centers and AE is tangent to both circles, find DC.
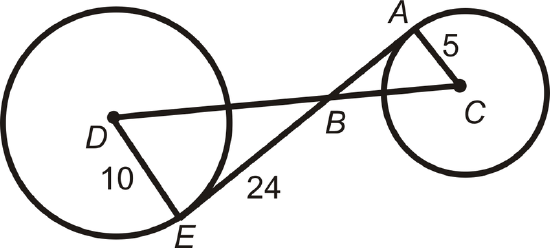
Solution
¯AE⊥¯DE and ¯AE⊥¯AC and ΔABC∼ΔDBE by AA Similarity.
To find DB, use the Pythagorean Theorem.
102+242=DB2100+576=676DB=√676=26
To find BC, use similar triangles.
510=BC26→BC=13.DC=DB+BC=26+13=39
Example 6.18.3
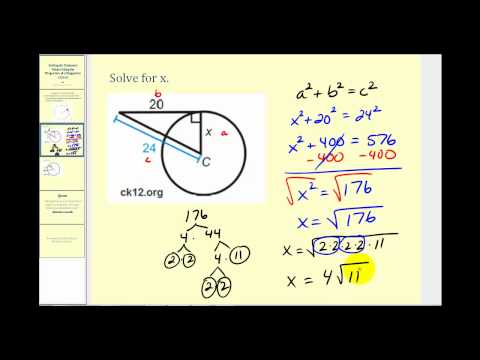
¯CB is tangent to ⨀A at point B. Find AC. Reduce any radicals.
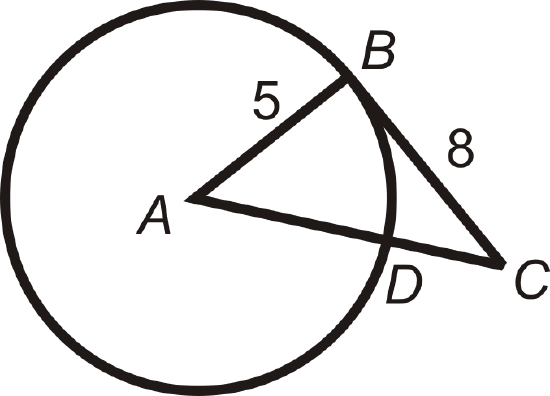
Solution
¯CB is tangent, so ¯AB⊥¯CB and ΔABC a right triangle. Use the Pythagorean Theorem to find AC.
52+82=AC225+64=AC289=AC2AC=√89
Example 6.18.4
Using the answer from Example A above, find DC in ⨀A. Round your answer to the nearest hundredth.
Solution
DC=AC−ADDC=√89−5≈4.43
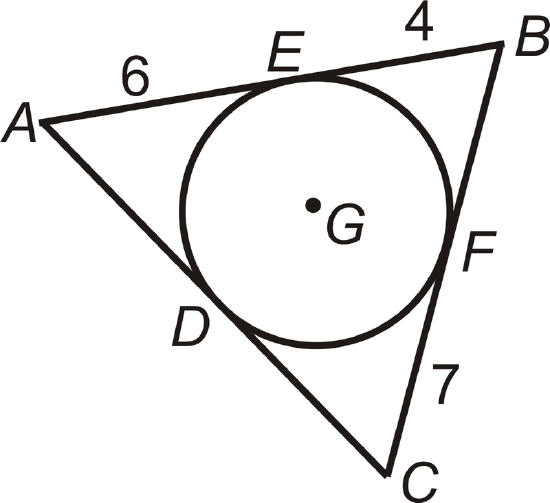
Example 6.18.5
Find the perimeter of ΔABC.
Solution
AE=AD, EB=BF, and CF=CD. Therefore, the perimeter of
ΔABC=6+6+4+4+7+7=34.
⨀G is inscribed in ΔABC. A circle is inscribed in a polygon if every side of the polygon is tangent to the circle.
Review
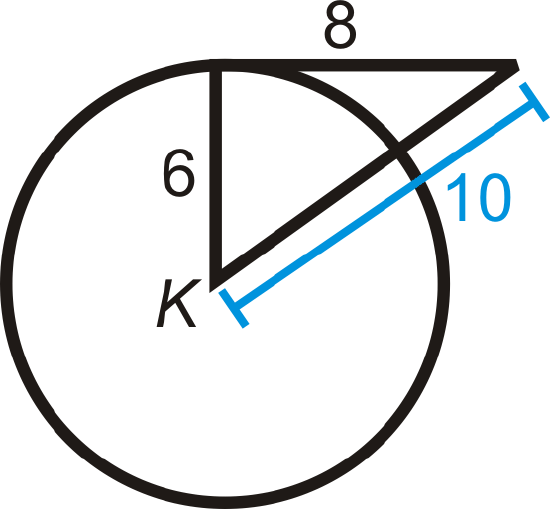
Determine whether the given segment is tangent to ⨀K.
-
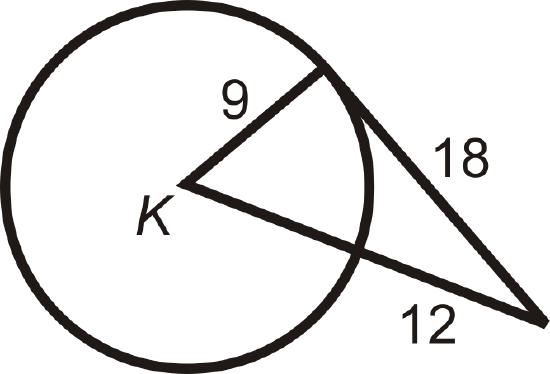
Figure 6.18.8 -
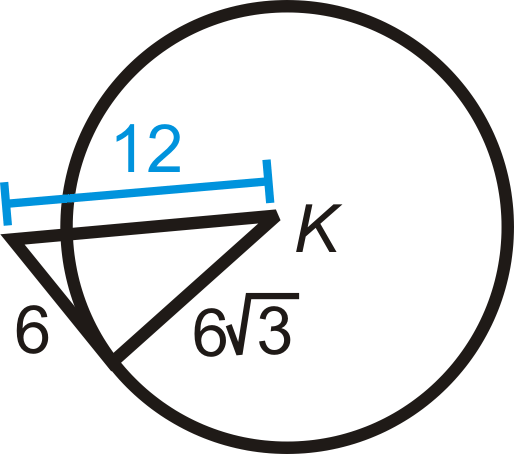
Figure 6.18.9 -
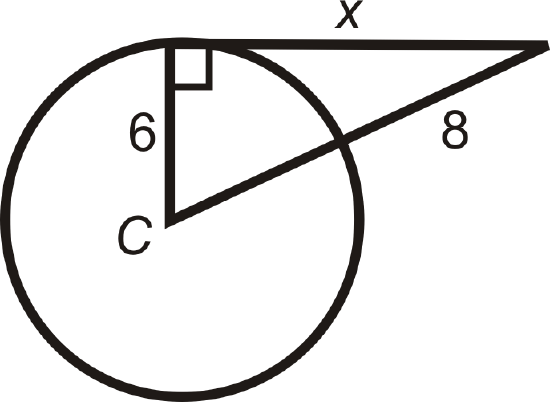
Figure 6.18.10
Find the value of the indicated length(s) in ⨀C. A and B are points of tangency. Simplify all radicals.
-
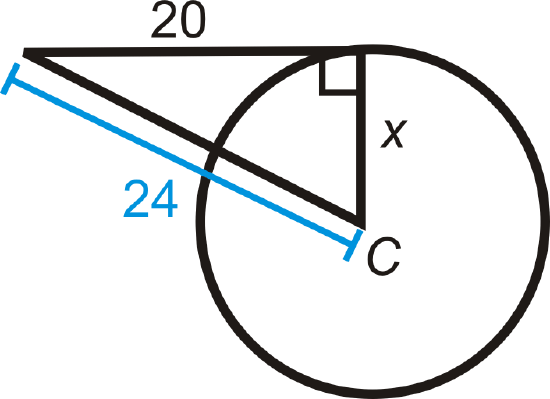
Figure 6.18.11 -
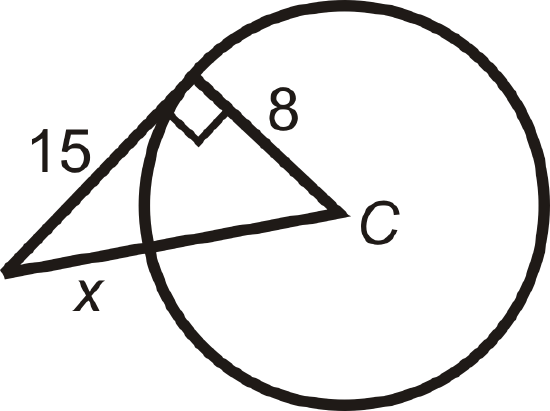
Figure 6.18.12 -
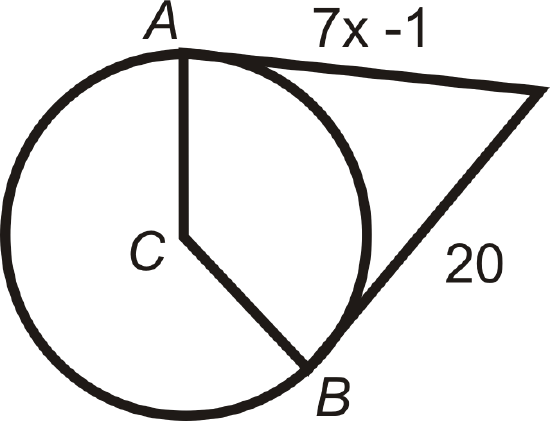
Figure 6.18.13 -
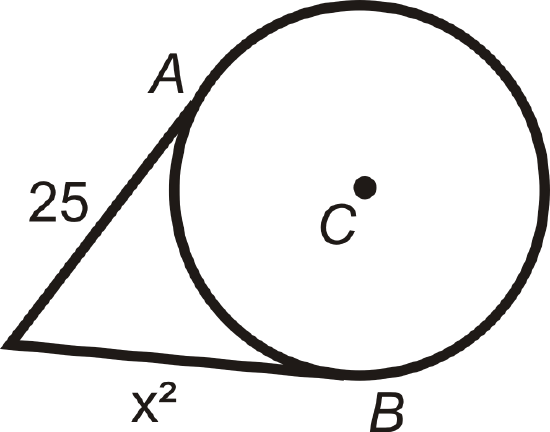
Figure 6.18.14 -
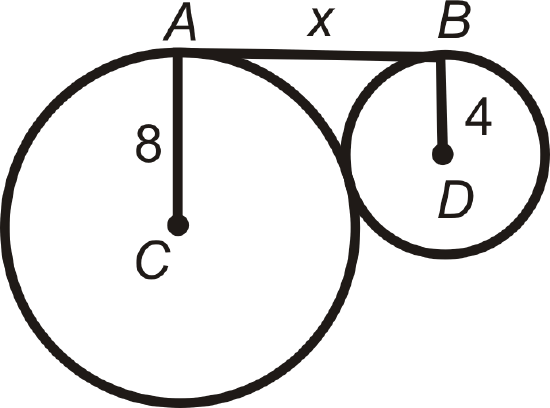
Figure 6.18.15 -

Figure 6.18.16
A and B are points of tangency for ⨀C and ⨀D.
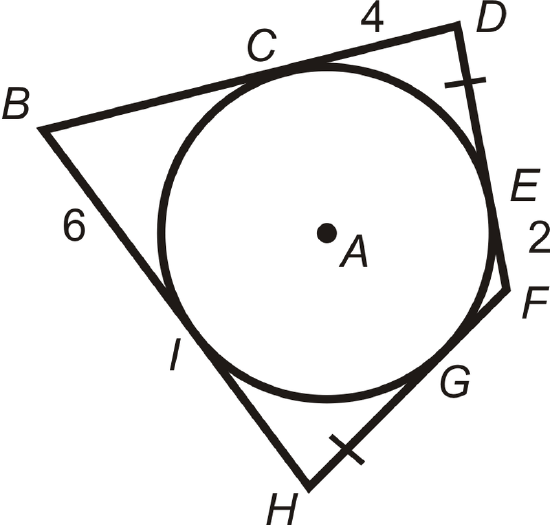
- Is ΔAEC∼ΔBED? Why?
- Find CE.
- Find BE.
- Find ED.
- Find BC and AD.
⨀A is inscribed in BDFH.
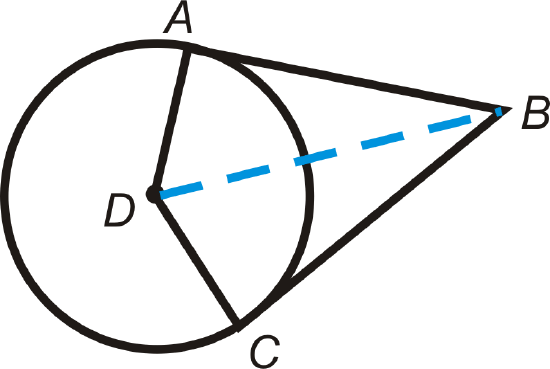
- Find the perimeter of BDFH.
- What type of quadrilateral is BDFH? How do you know?
- Draw a circle inscribed in a square. If the radius of the circle is 5, what is the perimeter of the square?
- Can a circle be inscribed in a rectangle? If so, draw it. If not, explain.
- Draw a triangle with two sides tangent to a circle, but the third side is not.
- Can a circle be inscribed in an obtuse triangle? If so, draw it. If not, explain.
- Fill in the blanks in the proof of the Two Tangents Theorem.
Given: ¯AB and ¯CB with points of tangency at A and C. ¯AD and ¯DC are radii.
Prove: ¯AB≅¯CB
| Statement | Reason |
|---|---|
| 1. | 1. |
| 2. ¯AD≅¯DC | 2. |
| 3. ¯DA⊥¯AB and ¯DC⊥¯CB | 3. |
| 4. | 4. Definition of perpendicular lines |
| 5. | 5. Connecting two existing points |
| 6. ΔADB and ΔDCB are right triangles | 6. |
| 7.¯DB≅¯DB | 7. |
| 8.ΔABD≅ΔCBD | 8. |
| 9.¯AB≅¯CB | 9. |
- Fill in the blanks, using the proof from #21.
- ABCD is a _____________ (type of quadrilateral).
- The line that connects the ___________ and the external point B __________ ∠ABC.
- Points A, B, and C are points of tangency for the three tangent circles. Explain why ¯AT≅¯BT≅¯CT.
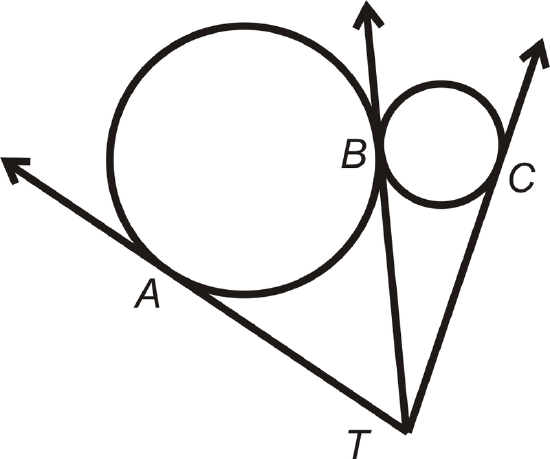
Figure 6.18.19
Review (Answers)
To see the Review answers, open this PDF file and look for section 9.2.
Resources
Vocabulary
| Term | Definition |
|---|---|
| circle | The set of all points that are the same distance away from a specific point, called the center. |
| diameter | A chord that passes through the center of the circle. The length of a diameter is two times the length of a radius. |
| point of tangency | The point where the tangent line touches the circle. |
| radius | The distance from the center to the outer rim of a circle. |
| Tangent | The tangent of an angle in a right triangle is a value found by dividing the length of the side opposite the given angle by the length of the side adjacent to the given angle. |
| Tangent to a Circle Theorem | A line is tangent to a circle if and only if the line is perpendicular to the radius drawn to the point of tangency. |
| Two Tangent Theorem | The Two-Tangent Theorem states that if two tangent segments are drawn to one circle from the same external point, then they are congruent. |
Additional Resources
Interactive Element
Video: Tangent Lines Principles - Basic
Activities: Tangent Lines Discussion Questions
Study Aids: Properties of a Circle Study Guide
Practice: Tangent Lines
Real World: Swing Rides