13.7: Single Slit Diffraction
- Page ID
- 4904
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)
Though it looks like a double slit interference pattern, the pattern on the screen are actually the results of light diffracting through a single slit with the ensuing interference.
Single Slit Interference
Interference patterns are produced not only by double slits but also by single slits, otherwise known as single slit interference. In the case of a single slit, the particles of medium at both corners of the slit act as point sources, producing circular waves from both edges. These circular waves move across to the back wall and interfere in the same way that interference patterns were produced by double slits.
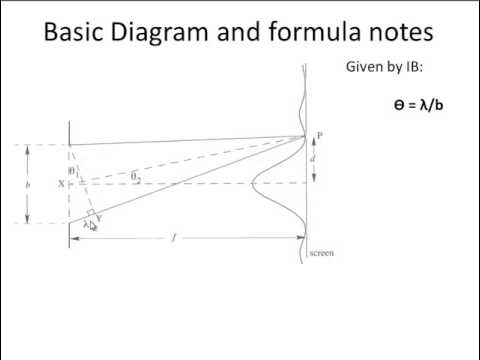
In the sketch at below, the black lines intersect at the center of the pattern on the back wall. This center point is equidistanct from both edges of the slit. Therefore, the waves striking at this position will be in phase; that is, the waves will produce constructive interference. Also shown in the sketch, just above the central bright spot where the red lines intersect, is a position where destructive interference occurs. One of these red lines is one-half wavelength longer than the other, causing the two waves to hit the wall out of phase and undergo destructive interference. A dark bank appears at this position.
Just as in double slit interference, a pair of similar triangles can be constructed in the interference pattern. The pertinent values from these triangles are the width of the slit, w, the wavelength, λ, the distance from the central bright spot to the first dark band, x, and the distance from the center of the slit to back wall, L. The relationship of these four values is
λ/w=x/L or λ=wx/L.
Example 13.7.1
Monochromatic light of wavelength 605 nm falls on a slit of width 0.095 mm. The slit is located 85 cm from a screen. How far is the center of the central bright band to the first dark band?
Solution
x=λL/w=(6.05×10−7 m)(0.85 m)/(9.5×10−5 m)=0.0054 m
Launch the PLIX Interactive below to observe light passing through a single slit and try to use the resulting interference pattern to determine the light’s wavelength:
Interactive Element
Summary
- Interference patterns can also be produced by single slits.
- In the case of a single slit, the particles of medium at both corners of the slit act as point sources, and produce circular waves from both edges.
- The wavelength can be determined by this equation: λ/w=x/L or λ=wx/L.
Review
- The same set up is used for two different single slit diffraction experiments. In one of the experiments, yellow light is used, and in the other experiment, green light is used. Green light has a shorter wavelength than yellow light. Which of the following statements is true?
- The two experiments will have the same distance between the central bright band and the first dark band.
- The green light experiment will have a greater distance between the central bright band and the first dark band.
- The yellow light experiment will have a greater distance between the central bright band and the first dark band.
- Why are the edges of shadows often fuzzy?
- Interference occurs on the wall on which the shadow is falling.
- Light diffracts around the edges of the object casting the shadow.
- The edges of the object casting the shadow is fuzzy.
- Light naturally spreads out.
- Monochromatic, coherent light passing through a double slit will produce exactly the same interference pattern as when it passes through a single slit.
- True
- False
- If monochromatic light passes through a 0.050 mm slit and is projected onto a screen 0.70 m away with a distance of 8.00 mm between the central bright band and the first dark band, what is the wavelength of the light?
- A krypton ion laser with a wavelength of 524.5 nm illuminates a 0.0450 mm wide slit. If the screen is 1.10 m away, what is the distance between the central bright band and the first dark band?
- Light from a He-Ne laser (λ=632.8 nm) falls on a slit of unknown width. In the pattern formed on a screen 1.15 m away, the first dark band is 7.50 mm from the center of the central bright band. How wide is the slit?
Explore More
Use this resource to answer the question that follows.
- What are interference patterns caused by?
- What does the placement of light and dark bands or fringes depend on?
- How do the fringes change as you move away from the center?
- What happens when white light is used instead of monochromatic light?
Additional Resources
Study Guide: Wave Optics Study Guide
Video: Physical Optics: Polarization, Diffraction and Interference - Overview
Real World Application: 3-D photographs
Interactive: The Marina