13.8: Double Slit Diffraction
- Page ID
- 4905
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)When waves strike a small slit in a wall, they create circular wave patterns on the other side of the barrier. This is seen in the image above, where ocean waves create precise circular waves. The circular waves undergo constructive and destructive interference, which generates a regular interference pattern.
Diffraction and Interference
When a series of straight waves strike an impenetrable barrier, the waves stop at the barrier. However, the last particle of the medium at the back corner of the barrier will create circular waves from that point, called the point source. This can be seen in the image below. This phenomenon is called diffraction, and it occurs in liquid, sound, and light waves. While the waves become circular waves at the point source, they continue as straight waves where the barrier does not interfere with the waves.
Any two waves in the same medium undergo wave interference as they pass each other. At the location where the two waves collide, the result is essentially a summation of the two waves. In some places, a wave crest from one source will overlap a wave crest from the other source. Since both waves are lifting the medium, the combined wave crest will be twice as high as the original crests. Nearby, a wave trough will overlap another wave trough and the new trough will be twice as deep as the original. This is called constructive interference because the resultant wave is larger than the original waves. Within the interference pattern, the amplitude will be twice the original amplitude. Once the waves pass through each other and are alone again, their amplitudes return to their original values.
In other parts of the wave pattern, crests from one wave will overlap troughs from another wave. When the two waves have the same amplitude, this interaction causes them to cancel each other out. Instead of a crest or a trough, there is nothing. When this cancellation occurs, it is called destructive interference.
It is easy to see how waves emanating from multiple sources, such as drops of rainwater in still water, create interference patterns. But a single source of waves can create interference patterns with itself as a result of diffraction.
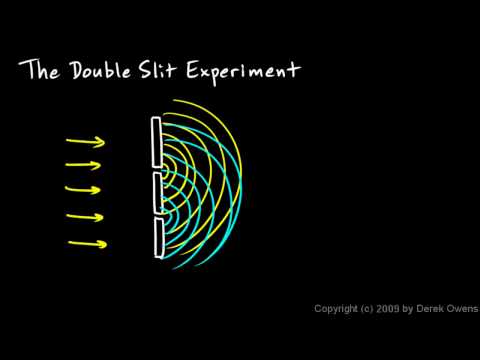
The Double Slit Experiment
A similar situation to the raindrops above occurs when straight waves strike a barrier containing two slits. These waves are cut off everywhere except for where the waves that pass through the two slits. The medium in the slits again acts as a point source to produce circular waves on the far side of the barrier.
As long as these two circular waves have the same wavelength, they interfere constructively and destructively in a specific pattern. This pattern is called the wave interference pattern and is characterized by light and dark bands. The light bands are a result of constructive interference and the dark bands occur because of destructive interference.
In the early 1800s, light was assumed to be a particle. There was a significant amount of evidence to point to that conclusion, and famous scientist Isaac Newton's calculations all support the particle theory. In 1803, however, Thomas Young performed his famous Double Slit Experiment to prove that light was a wave. Young shined a light onto the side of a sealed box with two slits in it, creating an interference pattern on the inside of the box opposite the slits. As seen above, interference patterns are characterized by alternating bright and dark lines. The bright lines are a result of constructive interference, while the dark lines are a result of destructive interference. By creating this interference pattern, Young proved light is a wave and changed the course of physics.
Calculating Wavelength from Double Slit Pattern
Using the characteristics of the double slit interference pattern, it is possible to calculate the wavelength of light used to produce the interference. To complete this calculation, it is only necessary to measure a few distances. As can be seen below, five distances are measured. In the sketch, L is the distance from the two slits to the back wall where the interference pattern can be seen. d is the distance between the two slits. To understand x, look again at the interference pattern shown above. The middle line, which is the brightest, is called the central line. The remaining lines are called fringes. The lines on either side of the central line are called the first order fringes, the next lines are called the second order fringes, and so on. x is the distance from the central line to the first order fringe.
r1 and r2 are the distances from the slits to the first order fringe. We know that the fringes are a result of constructive interference, and that the fringe is a result of the crest of two waves interfering. If we assume that r2 is a whole number of wavelengths (confirm for yourself that this is a logical assumption), then r1 must be one more wavelength. This is because r1 and r2 are the distances to the first order fringe. Mathematically, we can let
r2=nλ and r1=nλ+λ, where λ is the wavelength and n is a constant.
Using this relationship, we determine that r1−r2=λ.
Looking again at the diagram, the red and blue triangles are similar, which means that the ratios of corresponding sides are the same. The ratio of x to L in the red triangle is equal to the ratio of λ to d in the blue triangle. From this, we can determine that the wavelength is dependent on x, d, and L:
λ=xd/L
Example 13.8.1
Monochromatic light falls on two narrow slits that are 0.0190 mm apart. A first order fringe is 21.1 mm from the central line. The screen (back wall) is 0.600 m from the slits. What is the wavelength of the light?
Solution
λ=xd/L=(0.021 m)(0.000019 m)/(0.600 m)=6.68×10−7 m
Use the Marina simulation below to interact with waves that are traveling at a steady speed and wavelength toward the shore. The peaks of these waves are represented as horizontal lines at the top of the screen. The point in the middle between two lines indicates a wave trough, where the water is lowest. If you adjust the wavelength with the slider you will notice that shorter wavelengths are represented by lines closer together. Have fun learning about the concept of double slit diffraction in this real-world setting:
Interactive Element
Summary
- The last particle of medium at the back corner of an impenetrable barrier will act as a point source and produce circular waves.
- Diffraction is the bending of waves around a corner.
- Constructive interference occurs when two wave crests overlap, doubling the wave amplitude at that location.
- Destructive interference occurs when a wave crest overlaps with a trough, causing them to cancel out.
- Light is a wave, and creates an interference pattern in the double slit experiment.
- An interference pattern consists of alternating bright and dark lines; the bright lines are called fringes.
- In a double slit experiment, the wavelength can be calculated using this equation: λ=xd/L
Review
- Destructive interference in waves occurs when
- two troughs overlap.
- crests and troughs align.
- two crests overlap.
- a crest and a trough overlap.
- Bright bands in interference patterns result from
- destructive diffraction.
- destructive interference.
- constructive diffraction.
- constructive interference.
- In a double slit experiment with slits 1.00×10−5 m apart, light casts the first bright band 3.00×10−2 m from the central bright spot. If the screen is 0.650 m away, what is the wavelength of this light?
- 510 nm
- 390 nm
- 430 nm
- 460 nm
- Violet light falls on two slits separated by 1.90×10−5 m. A first order bright line appears 13.2 mm from the central bright spot on a screen 0.600 m from the slits. What is the wavelength of the violet light?
- Suppose in the previous problem, the light was changed to yellow light with a wavelength of 5.96×10−7 m while the slit separation and distance from screen to slits remained the same. What would be the distance from the central bright spot to the first order line?
- Light with a wavelength of 6.33×10−7 m is used in a double slit experiment. The screen is placed 1.00 m from the slits and the first order line is found 65.5 mm from the central bright spot. What is the separation between the slits?
Explore More
Use this resource to answer the questions that follow.
- When the amplitude of waves add, it is called _________________ interference.
- When the amplitude of waves subtract, it is called _________________ interference.
- What do we call the phenomenon of light bending around a corner?
Additional Resources
Study Guide: Wave Optics Study Guide
PLIX: Play, Learn, Interact, eXplore: Interference Patterns
Videos: