1.6: Points that Partition Line Segments
- Page ID
- 2123
Section formula finds coordinates of a point that splits a line segment in a given ratio.
Recall that a median of a triangle is a line segment that connects a vertex of the triangle to the midpoint of the side opposite the vertex. All triangles have three medians and these three medians intersect in one point called the centroid, shown below. The centroid partitions each median in a 2:1 ratio.
 Figure \(\PageIndex{1}\)
Figure \(\PageIndex{1}\)Find the coordinates of the centroid, given the coordinates of the vertices of the triangle as shown.
Partitions
Suppose you have a line segment \(\overline{AB}\). A point \(P\) divides this line segment into two parts such that \(AP=mk\) and \(PB=nk\). You can say that point \(P\) partitions segment \(AB\) in a \(m:n\) ratio. (Note that \(\dfrac{mk}{nk}=mn\), a ratio of \(m:n\).)
 Figure \(\PageIndex{2}\)
Figure \(\PageIndex{2}\)A natural question to ask is, what are the coordinates of point \(P\)? It turns out that with the help of similar triangles and algebra, you can come up with a formula that will give you the coordinates of point \(P\) based on the coordinates of \(A\), the coordinates of \(B\), and the ratio \(m:n\). This formula is sometimes referred to as the section formula.
Section Formula: Given \(\overline{AB}\) with \(A=(x_1, y_1)\) and \(B=(x_2, y_2)\), if point \(P\) partitions \(\overline{AB}\) in a \(m:n\) ratio, then the coordinates of point P are:
\(P=\left (\dfrac{mx_{2}+nx_{1}}{m+n}, \dfrac{my_{2}+ny_{1}}{m+n}\right )\)
Proving Triangle Similarity
For segment \(\overline{AB}\) below, draw two right triangles, one with hypotenuse \(\overline{AP}\) and one with hypotenuse \(\overline{PB}\). Show that these triangles are similar.
 Figure \(\PageIndex{3}\)
Figure \(\PageIndex{3}\)Start by drawing the right triangles. Below, the base and height of each triangle has been labeled in green.
 Figure \(\PageIndex{4}\)
Figure \(\PageIndex{4}\)Clearly these triangles have one pair of congruent angles (the right angles). What other information do you have about the triangles? You know that for each triangle, the ratio \(\dfrac{height}{base}\) is the slope of \(\overline{AB}\). Because these two triangles are attached to the same line segment with the same slope, it means that \(\dfrac{h_1}{b_1} = {h_2}{b_2}\). This is equivalent to \(\dfrac{b_2}{b_1}=\dfrac{h_2}{h_1}\). Two pairs of sides are in the same ratio.
Not only is there one pair of congruent angles, but there are also two pairs of corresponding sides with the same ratio. The triangles are similar by \(SAS\)∼.
Finding Length and Height
Find the lengths of the bases and heights of each triangle. Use the fact that the triangles are similar to set up and solve proportions for \(x\) and then for \(y\) in order to find the coordinates of point \(P\).
 Figure \(\PageIndex{5}\)
Figure \(\PageIndex{5}\)The bases and heights can be found in terms of \(x_1\), \(y_1\), \(x\), \(y\), \(x_2\), \(y_2\).
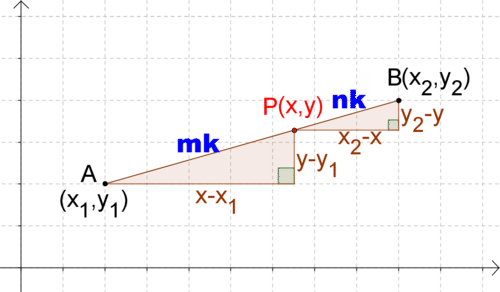 Figure \(\PageIndex{6}\)
Figure \(\PageIndex{6}\)Because the triangles are similar, the ratios between pairs of corresponding sides are equal. In particular, you know:
- \(\dfrac{mk}{nk}=\dfrac{x−x_1}{x_2−x}\)
- \(\dfrac{mk}{nk}=\dfrac{y−y_1}{y_2−y}\)
You can use algebra to solve the first equation for \(x\) and the second equation for \(y\).
- \(\dfrac{mk}{nk}=\dfrac{x−x_1}{x_2−x}\) \(\rightarrow \dfrac{m}{n}=\dfrac{x−x_1}{x_2−x} \)
\(\rightarrow mx_2−mx=nx−nx_1\)
\(\rightarrow mx_2+mx_1=mx+nx \)
\(\rightarrow mx_2−nx_1=x(m+n)\)
\(\rightarrow \dfrac{mx_2−nx_1}{m+n}=\dfrac{x(m+n)}{m+n}\)
\(\rightarrow x=\dfrac{mx_2+nx_1}{m+n}\)
- \(\dfrac{mk}{nk}=\dfrac{y−y_1}{y_2−y}\) \(\rightarrow\dfrac{m}{n}=\dfrac{y−y_1}{y_2−y} \)
\(\rightarrow my_2−my=ny−ny_1\)
\(\rightarrow my_2+my_1=my+ny\)
\(\rightarrow my_2−ny_1=y(m+n)\)
\(\rightarrow \dfrac{my_2−ny_1}{m+n}=\dfrac{y(m+n)}{m+n}\)
\(\rightarrow x=\dfrac{my_2+ny_1}{m+n}\)
Point \(P\) is at:
\(P=\left(\dfrac{mx_2+nx_1}{m+n} ,\dfrac{my_2+ny_1}{m+n}\right)\)
Finding the Coordinates of a Point
Consider \(\overline{AB}\) with \(A=(10, 2)\) and \(B=(4, 1)\). \(P\) partitions \(\overline{AB}\) in a ratio of \(2:3\). Find the coordinates of point \(P\).
You can use the section formula with \((x_1,y_1)=(10, 2)\), \((x_2, y_2)=(4, 1)\), \(m=2\), \(n=3\).
\(P=\left(\dfrac{mx_2+nx_1}{m+n} ,\dfrac{my_2+ny_1}{m+n}\right)=\left(\dfrac{2 \cdot 4+3 \cdot10}{2+3}, \dfrac{2 \cdot 1+3\cdot 2}{2+3}\right)=\left(7.6,1.6\right)\)
You can plot points \(A\), \(B\), and \(P\) to see if this answer is realistic.
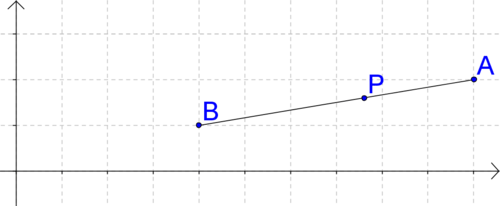 Figure \(\PageIndex{7}\)
Figure \(\PageIndex{7}\)This does look like \(P\) partitions the segment from \(A\) to \(B\) in a ratio of \(2:3\). Note that the answer would be different if you were looking for the point that partitioned the segment from \(B\) to \(A\). The order of the letters and “direction” of the segment matters.
Example \(\PageIndex{1}\)
Earlier, you were asked to find the coordinates of the centroid, given the coordinates of the vertices of the triangle as shown.
 Figure \(\PageIndex{8}\)
Figure \(\PageIndex{8}\)Solution
One way to find the coordinates of the centroid is to use the section formula. You can focus on any of the three medians. Here, look at the median from point A. First, you will need to find the coordinates of the midpoint of \(\overline{BC}\) (the midpoint formula, a special case of the section formula, is derived in Guided Practice #1 and #2):
\(\left(\dfrac{x_2+x_1}{2}, \dfrac{y_2+y_1}{2}\right)=\left (\dfrac{5+6}{2}, \dfrac{5+1}{2}\right)=\left(5.5,3\right )\)
\(\left(\dfrac{mx_2+nx_1}{m+n}, \dfrac{my_2+ny_1}{m+n}\right)=\left(\dfrac{2 \cdot 5.5+1 \cdot 2}{2+1}, \dfrac{2 \cdot 3+1 \cdot 6}{2+1}\right)=\left(\dfrac{13}{3}, 4\right)\)
Looking at the picture, these coordinates for the centroid are realistic.
Example \(\PageIndex{2}\)
The midpoint of a line segment is the point exactly in the middle of the line segment. In what ratio does a midpoint partition a segment?
Solution
1:1, because the segments connecting the midpoint to each endpoint will be the same length.
Example \(\PageIndex{3}\)
The midpoint formula is a special case of the section formula where m=n=1. Derive a formula that calculates the midpoint of the segment connecting \((x_1,y_1)\) with \((x_2, y_2)\).
Solution
For a midpoint, \(m=n=1\). The section formula becomes:
\(\left(\dfrac{mx_2+nx_1}{m+n}, \dfrac{my_2+ny_1}{m+n}\right)=\left(\dfrac{x_2+x_1}{2}, \dfrac{y_2+y_1}{2}\right)
This is the midpoint formula.
Example \(\PageIndex{4}\)
Consider \(\overline{BA}\) with \(B=(4, 1)\) and \(A=(10, 2)\). \(P\) partitions the segment in a ratio of \(2:3\). Find the coordinates of point \(P\). How and why is this answer different from the answer to Example \(C\)?
Solution
\((x_1, y_1)=(4,1)\) and \((x_2, y_2)=(10,2)\). \(m=2\) and \(n=3\).
\(P=\left(\dfrac{mx_2+nx_1}{m+n}, \dfrac{my_2+ny_1}{m+n}\right)=\left(\dfrac{2 \cdot 10+3 \cdot 4}{2+3}, \dfrac{2 \cdot 2+3 \cdot 1}{2+3}\right)=\left(6.4,1.4\right)\)
This answer is different from the answer to Example C because in this case point P is partitioning the segment in a \(2:3\) ratio starting from point \(B\). In Example \(C\), you were starting from point \(A\).
Review
Find the midpoint of each of the following segments defined by the given endpoints.
1. \((2, 6)\) and \((−4, 8)\)
2. \((1, 9)\) and \((−2, 5)\)
3. \((11, 24)\) and \((8, 12)\)
4. \((1, 3)\) is the midpoint of \(\overline{AB}\) with \(A=(−2, 1)\). Find the coordinates of \(B\).
5. \((2, 4)\) is the midpoint of \(\overline{CD}\) with \(C=(−5, 9)\). Find the coordinates of \(D\).
6. \((4, 23)\) is the midpoint of \(\overline{EF}\) with \(E=(7, 11)\). Find the coordinates of \(F\).
Consider \(A=(−9, 4)\) and \(B=(11, 17)\).
7. Point \(P_1\) partitions the segment from \(A\) to \(B\) in a \(3:5\) ratio. Find the coordinates of point \(P_1\).
8. Point \(P_2\) partitions the segment from \(B\) to \(A\) in a \(3:5\) ratio. Find the coordinates of point \(P_2\).
9. Why are the answers to 7 and 8 different?
10. Find the length of \(AP_1\) and \(P_2B\). Why should these lengths be the same?
Consider \(C=(−6, −1)\) and \(D=(4, 8)\).
11. Point \(P_3\) partitions the segment from \(A\) to \(B\) in a \(1:2\) ratio. Find the coordinates of point \(P_3\).
12. Point \(P_4\) partitions the segment from \(B\) to \(A\) in a \(4:5\) ratio. Find the coordinates of point \(P_4\).
13. Point \(P=(1, 2)\) partitions the segment from \(E=(9, 6)\) to \(F\) in a \(2:5\) ratio. Find the coordinates of point F.
14. Point \(P=(−6, −4)\) partitions the segment from \(G=(−4, 6)\) to \(H\) in a \(5:3\) ratio. Find the coordinates of point \(H\).
15. Point \(P=(6,8)\) partitions the segment from \(I=(−2,1)\) to \(J\) in a \(6:7\) ratio. Find the coordinates of point \(J\).
16. A triangle is defined by the points \((5, 6)\), \((9, 17)\), and \((−2, 1)\). Find the coordinates of the centroid of the triangle.
Review (Answers)
To see the Review answers, open this PDF file and look for section 10.6.
Vocabulary
| Term | Definition |
|---|---|
| centroid | The centroid is the point of intersection of the medians in a triangle. |
| Median | The median of a triangle is the line segment that connects a vertex to the opposite side's midpoint. |
| midpoint | The midpoint of a line segment is the point on the line segment that splits the segment into two congruent parts. |
| Midpoint Formula | The midpoint formula says that for endpoints \((x_{1},y_{1})\) and \((x_{2},y_{2})\), the midpoint is \(\left (\dfrac{x_1+x_2}{2}, \dfrac{y_1+y_2}{2}\right)\). |
| Partitions | To partition is to divide into parts. |
| Section Formula | The section formula states how to find the coordinates of a point that partitions a line segment in a given ratio. |
Additional Resource
Interactive Element
Practice: Points that Partition Line Segments

