3.11: Probability
- Page ID
- 1456
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)
What are the odds of landing on 25 again?
Not as high as inheriting an allele from a parent. Probability plays a big role in determining the chance of inheriting an allele from a parent. It is similar to tossing a coin. What's the chance of the coin landing on heads?
Probability
Assume you are a plant breeder trying to develop a new variety of plant that is more useful to humans. You plan to cross-pollinate an insect-resistant plant with a plant that grows rapidly. Your goal is to produce a variety of plant that is both insect resistant and fast growing. What percentage of the offspring would you expect to have both characteristics? Mendel’s laws can be used to find out. However, to understand how Mendel’s laws can be used in this way, you first need to know about probability.
Probability is the likelihood, or chance, that a certain event will occur. The easiest way to understand probability is with coin tosses (see the Figure below). When you toss a coin, the chance of a head turning up is 50 percent. This is because a coin has only two sides, so there is an equal chance of a head or tail turning up on any given toss.
Tossing a Coin. Competitions often begin with the toss of a coin. Why is this a fair way to decide who goes first? If you choose heads, what is the chance that the toss will go your way?If you toss a coin twice, you might expect to get one head and one tail. But each time you toss the coin, the chance of a head is still 50 percent. Therefore, it’s quite likely that you will get two or even several heads (or tails) in a row. What if you tossed a coin ten times? You would probably get more or less than the expected five heads. For example, you might get seven heads (70 percent) and three tails (30 percent). The more times you toss the coin, however, the closer you will get to 50 percent heads. For example, if you tossed a coin 1000 times, you might get 510 heads and 490 tails.
Probability and Inheritance
The same rules of probability in coin tossing apply to the main events that determine the genotypes of offspring. These events are the formation of gametes during meiosis and the union of gametes during fertilization.
Probability and Gamete Formation
How is gamete formation like tossing a coin? Consider Mendel’s purple-flowered pea plants again. Assume that a plant is heterozygous for the flower-color allele, so it has the genotype Bb (see the Figure below). During meiosis, homologous chromosomes, and the alleles they carry, segregate and go to different gametes. Therefore, when the Bb pea plant forms gametes, the B and b alleles segregate and go to different gametes. As a result, half the gametes produced by the Bb parent will have the B allele and half will have the b allele. Based on the rules of probability, any given gamete of this parent has a 50 percent chance of having the B allele and a 50 percent chance of having the b allele.
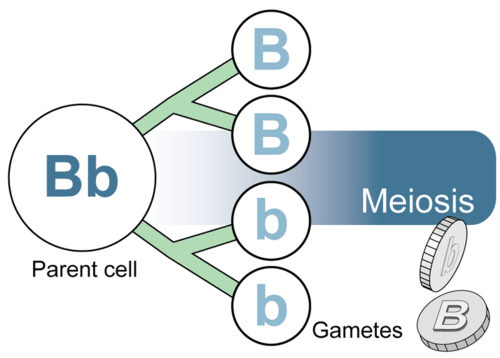Formation of gametes by meiosis. Paired alleles always separate and go to different gametes during meiosis.Probability and Fertilization
Which of these gametes joins in fertilization with the gamete of another parent plant? This is a matter of chance, like tossing a coin. Thus, we can assume that either type of gamete—one with the B allele or one with the b allele—has an equal chance of uniting with any of the gametes produced by the other parent. Now assume that the other parent is also Bb. If gametes of two Bb parents unite, what is the chance of the offspring having one of each allele like the parents (Bb)? What is the chance of them having a different combination of alleles than the parents (either BB or bb)? To answer these questions, geneticists use a simple tool called a Punnett square, which is the focus of the next concept.
Summary
- Probability is the chance that a certain event will occur. For example, the probability of a head turning up on any given coin toss is 50 percent.
- Probability can be used to predict the chance of gametes and offspring having certain alleles.
Review
- Define probability. Apply the term to a coin toss.
- How is gamete formation like tossing a coin?
- With a BB homozygote, what is the chance of a gamete having the B allele? The b allele?
| Image | Reference | Attributions |
 |
[Figure 1] | Credit: Mariana Ruiz Villarreal (LadyofHats) for CK-12 Foundation Source: CK-12 Foundation License: CC BY-NC 3.0 |
 |
[Figure 2] | Credit: Image copyright Anneka, 2014 Source: http://www.shutterstock.com License: Used under license from Shutterstock.com |
 |
[Figure 3] | Credit: Mariana Ruiz Villarreal (LadyofHats) for CK-12 Foundation Source: CK-12 Foundation License: CC BY-NC 3.0 |