5.27: Interior Angles in Convex Polygons

Last updated
Save as PDF

Page ID: 5012

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Use the formula \((x - 2)180\) to find the sum of the interior angles of any polygon.

The interior angle of a polygon is one of the angles on the inside, as shown in the picture below. A polygon has the same number of interior angles as it does sides.

f-d_389ff0723ea49e71f9ec7b5c21806d212be78a36ee4921973da5c040+IMAGE_TINY+IMAGE_TINY.png — Figure \(\PageIndex{1}\)

The sum of the interior angles in a polygon depends on the number of sides it has. The Polygon Sum Formula states that for any n−gon, the interior angles add up to \((n−2)\times 180^{\circ}\).

f-d_dc6113c85bfcaef0605b41b9ea2e6f06809d9fc3633e6525fcd7b83b+IMAGE_TINY+IMAGE_TINY.png — Figure \(\PageIndex{2}\)

\(\begin{aligned}\rightarrow n=8 \\ (8−2) &\times 180^{\circ} \\ 6 &\times 180^{\circ} \\ &1080^{\circ}\end{aligned}\)

Once you know the sum of the interior angles in a polygon it is easy to find the measure of ONE interior angle if the polygon is regular: all sides are congruent and all angles are congruent. Just divide the sum of the angles by the number of sides.

Regular Polygon Interior Angle Formula: For any equiangular n−gon, the measure of each angle is \(\dfrac{(n−2)\times 180^{\circ}}{n}\).

f-d_c35906e4638039ad92c1150cea19cce1d19012c9780b4e444b8b1185+IMAGE_TINY+IMAGE_TINY.png — Figure \(\PageIndex{3}\)

In the picture below, if all eight angles are congruent then each angle is \(\dfrac{(8−2)\times 180^{\circ}}{8}=\dfrac{6\times 180^{\circ}}{8}=\dfrac{1080^{\circ}}{8}=135^{\circ}\).

What if you were given an equiangular seven-sided convex polygon? How could you determine the measure of its interior angles?

Example \(\PageIndex{1}\)

The interior angles of a pentagon are \(x^{\circ}\), \(x^{\circ}\), \(2x^{\circ}\), \(2x^{\circ}\), and \(2x^{\circ}\). What is \(x\)?

Solution

From the Polygon Sum Formula we know that a pentagon has interior angles that sum to \((5−2)\times 180^{\circ}=540^{\circ}\).

Write an equation and solve for x.

\(\begin{aligned} x^{\circ}+x^{\circ}+2x^{\circ}+2x^{\circ}+2x^{\circ}&=540^{\circ} \\ 8x&=540 \\ x&=67.5\end{aligned}\)

Example \(\PageIndex{2}\)

What is the sum of the interior angles in a 100-gon?

Solution

Use the Polygon Sum Formula. \((100−2)\times 180^{\circ}=17,640^{\circ}\).

Example \(\PageIndex{3}\)

The interior angles of a polygon add up to \(1980^{\circ}\). How many sides does it have?

Solution

Use the Polygon Sum Formula and solve for n\).

\(\begin{aligned} (n−2)\times 180^{\circ}&=1980^{\circ} \\ 180^{\circ}n−360^{\circ}&=1980^{\circ} \\ 180^{\circ}n&=2340^{\circ} \\ n&=13\end{aligned}\)

The polygon has 13 sides.

Example \(\PageIndex{4}\)

How many degrees does each angle in an equiangular nonagon have?

Solution

First we need to find the sum of the interior angles; set \(n=9\).

\((9−2)\times 180^{\circ}=7\times 180^{\circ}=1260^{\circ}\)

“Equiangular” tells us every angle is equal. So, each angle is \(\dfrac{1260^{\circ}}{9}=140^{\circ}\).

Example \(\PageIndex{5}\)

An interior angle in a regular polygon is \(135^{\circ}\). How many sides does this polygon have?

Solution

Here, we will set the Regular Polygon Interior Angle Formula equal to \(135^{\circ}\) and solve for n.

\(\begin{aligned} \dfrac{(n−2)\times 180^{\circ}}{n}&=135^{\circ} \\ 180^{\circ}n−360^{\circ}−360^{\circ}&=135^{\circ}n \\ n&=−45^{\circ} \\ n&=8\qquad \text{The polygon is an octagon.} \end{aligned}\)

Review

Fill in the table.

# of sides	Sum of the Interior Angles	Measure of Each Interior Angle in a Regular n−gon
3		\(60^{\circ}\)
4	\(360^{\circ}\)
5	\(540^{\circ}\)	\(108^{\circ}\)
6		\(120^{\circ}\)
7
8
9
10
11
12

What is the sum of the angles in a 15-gon?
What is the sum of the angles in a 23-gon?
The sum of the interior angles of a polygon is \(4320^{\circ}\). How many sides does the polygon have?
The sum of the interior angles of a polygon is \(3240^{\circ}\). How many sides does the polygon have?
What is the measure of each angle in a regular 16-gon?
What is the measure of each angle in an equiangular 24-gon?
Each interior angle in a regular polygon is \(156^{\circ}\). How many sides does it have?
Each interior angle in an equiangular polygon is \(90^{\circ}\). How many sides does it have?

For questions 10-18, find the value of the missing variable(s).

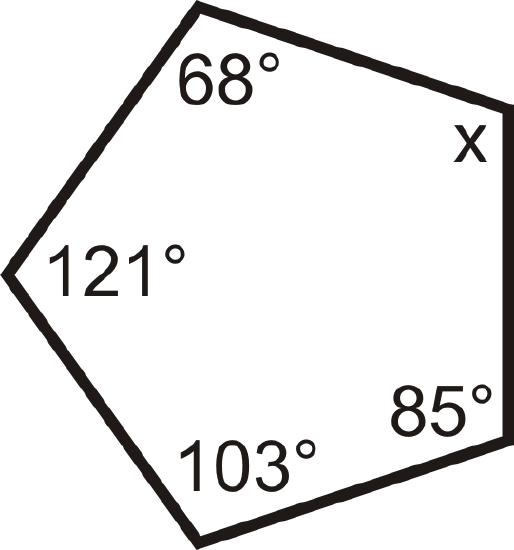
Figure \(\PageIndex{5}\)
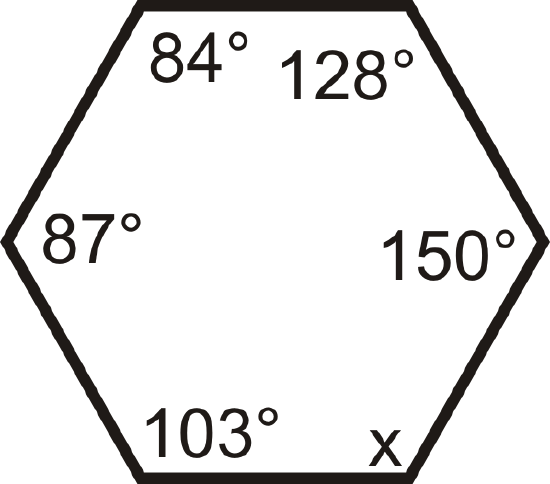
Figure \(\PageIndex{6}\)
Figure \(\PageIndex{7}\)
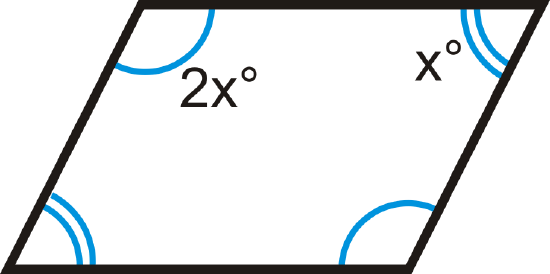
Figure \(\PageIndex{8}\)
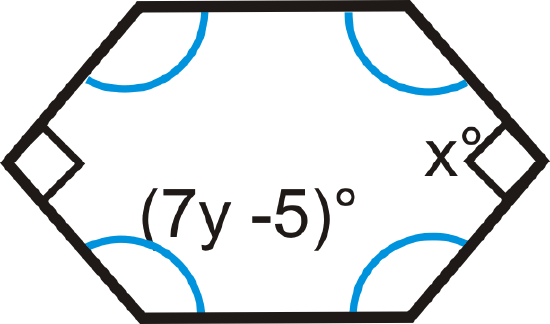
Figure \(\PageIndex{9}\)
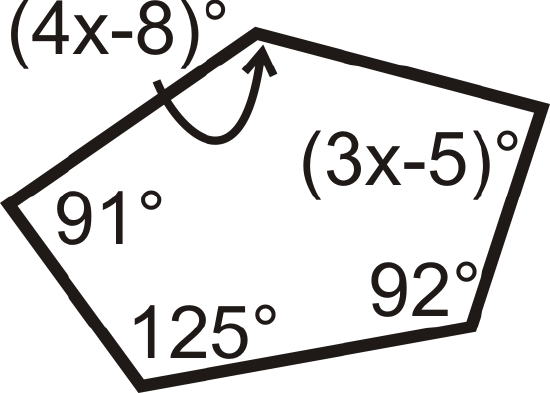
Figure \(\PageIndex{10}\)
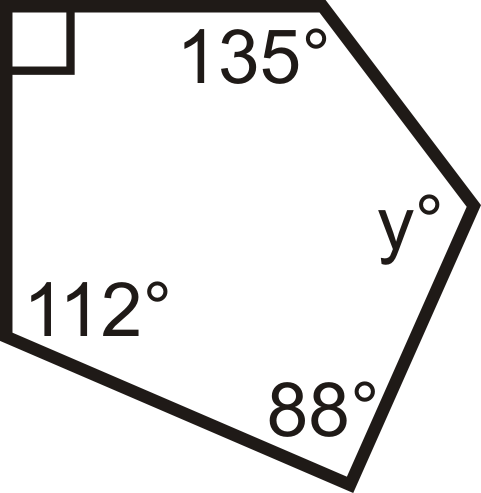
Figure \(\PageIndex{11}\)
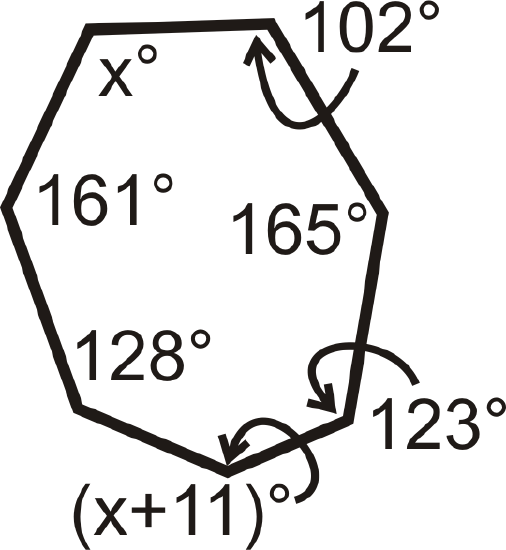
Figure \(\PageIndex{12}\)
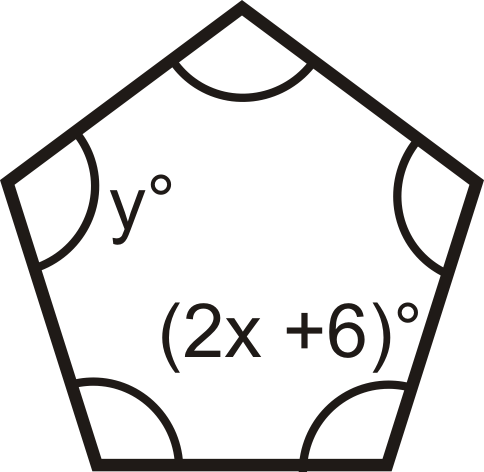
Figure \(\PageIndex{13}\)

The interior angles of a hexagon are \(x^{\circ}\), \((x+1)^{\circ}\), \((x+2)^{\circ}\), \((x+3)^{\circ}\),\((x+4)^{\circ}\), and \((x+5)^{\circ}\). What is \(x\)?

Review (Answers)

To see the Review answers, open this PDF file and look for section 6.1.

Resources

Vocabulary

Term	Definition
Interior angles	Interior angles are the angles inside a figure.
Polygon Sum Formula	The Polygon Sum Formula states that for any polygon with n sides, the interior angles add up to \((n−2)\times 180\) degrees.

Additional Resources

Interactive Element

Video: Interior and Exterior Angles of a Polygon

Activities: Interior Angles in Convex Polygons Discussion Questions

Study Aids: Polygons Study Guide

Practice: Interior Angles in Convex Polygons

Real World: Interior Angles In Convex Polygons