8.4: Rotation Symmetry
- Page ID
- 2163
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\(\newcommand{\longvect}{\overrightarrow}\)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\ket}[1]{\left| #1 \right>}\)
\(\newcommand{\bra}[1]{\left< #1 \right|}\)
\(\newcommand{\braket}[2]{\left< #1 \vphantom{#2} \right| \left. #2 \vphantom{#1} \right>}\)
\(\newcommand{\braopket}[3]{\left< #1 \vphantom{#2}\vphantom{#3} \right| #2 \vphantom{#1}\vphantom{#3} \left| #3 \vphantom{#1}\vphantom{#2} \right>}\)
\(\newcommand{\qmvec}[1]{\mathbf{\vec{#1}}}\)
\(\newcommand{\op}[1]{\hat{\mathbf{#1}}}\)
\(\newcommand{\expect}[1]{\langle #1 \rangle}\)
\(\newcommand{\dfn}[1]{\emph{\textbf{#1}}}\)
Rotation less than 360 degrees that carries a shape onto itself.
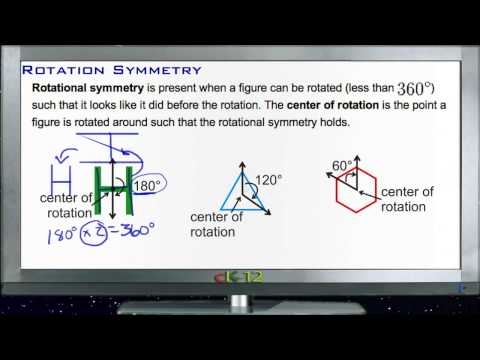
Rotational symmetry is present when a figure can be rotated (less than \(360^{\circ}\)) such that it looks like it did before the rotation. The center of rotation is the point a figure is rotated around such that the rotational symmetry holds.
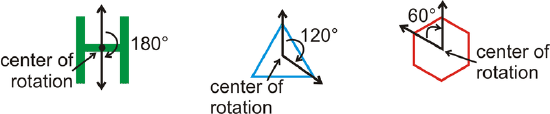
For the \(H\), we can rotate it twice, the triangle can be rotated 3 times and still look the same and the hexagon can be rotated 6 times.
What if you had a six-pointed star and you rotated that star less than \(360^{\circ}\)? If the rotated star looked exactly the same as the original star, what would that say about the star?
Example \(\PageIndex{1}\)
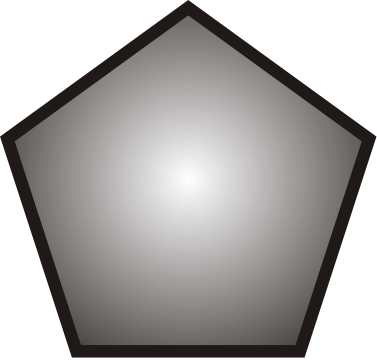
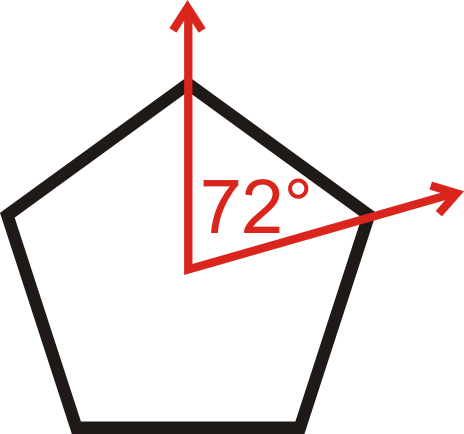
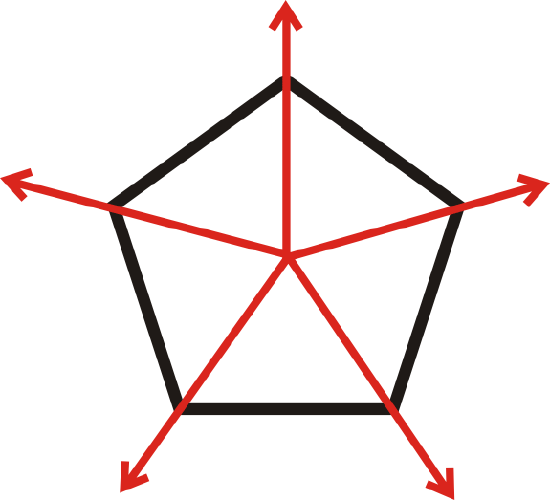
Determine if the figure below has rotational symmetry. Find the angle and how many times it can be rotated.
Solution
The pentagon can be rotated 5 times. Because there are 5 lines of rotational symmetry, the angle would be \(\dfrac{360^{\circ}}{5}=72^{\circ}\).
Example \(\PageIndex{2}\)
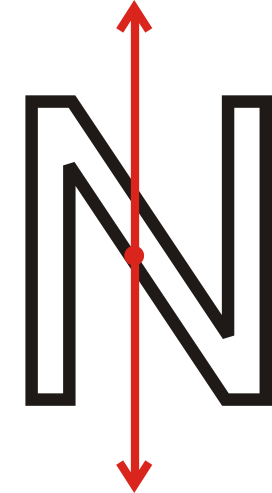
Determine if the figure below has rotational symmetry. Find the angle and how many times it can be rotated.
Solution
The N can be rotated twice. This means the angle of rotation is \(180^{\circ}\).
Example \(\PageIndex{3}\)
Determine if the figure below has rotational symmetry. Find the angle and how many times it can be rotated.
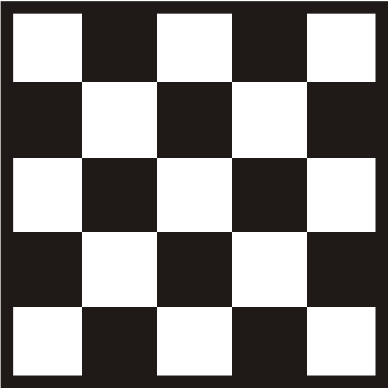
Solution
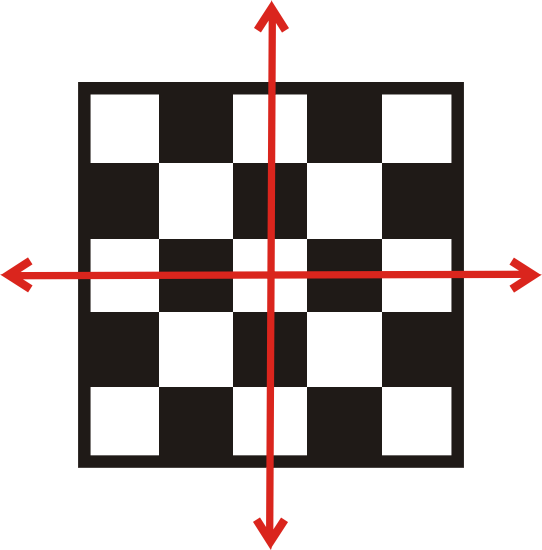
The checkerboard can be rotated 4 times. There are 4 lines of rotational symmetry, so the angle of rotation is \(\dfrac{360^{\circ}}{4}=90^{\circ}\).
Example \(\PageIndex{4}\)
Find the angle of rotation and the number of times each figure can rotate.
Solution
The parallelogram can be rotated twice. The angle of rotation is \(180^{\circ}\).
Example \(\PageIndex{5}\)
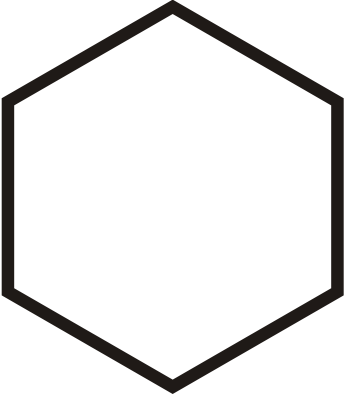
Solution
The hexagon can be rotated six times. The angle of rotation is \(60^{\circ}\).
Review
- If a figure has 3 lines of rotational symmetry, it can be rotated _______ times.
- If a figure can be rotated 6 times, it has _______ lines of rotational symmetry.
- If a figure can be rotated n times, it has _______ lines of rotational symmetry.
- To find the angle of rotation, divide \(360^{\circ}\) by the total number of _____________.
- Every square has an angle of rotation of _________.
Determine whether each statement is true or false.
- Every parallelogram has rotational symmetry.
- Every figure that has line symmetry also has rotational symmetry.
Determine whether the words below have rotation symmetry.
- OHIO
- MOW
- WOW
- KICK
- pod
Find the angle of rotation and the number of times each figure can rotate.
-
Figure \(\PageIndex{12}\) -
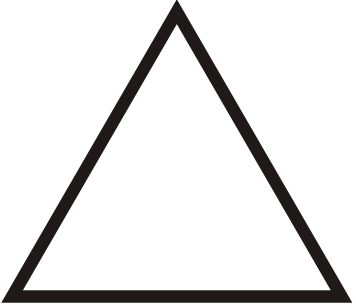
Figure \(\PageIndex{13}\) -
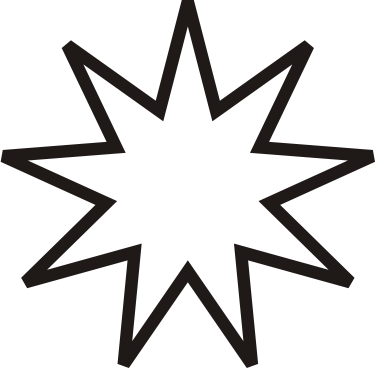
Figure \(\PageIndex{14}\)
Determine if the figures below have rotation symmetry. Identify the angle of rotation.
-
Figure \(\PageIndex{15}\) -
Figure \(\PageIndex{16}\) -
Figure \(\PageIndex{17}\)
Review (Answers)
To see the Review answers, open this PDF file and look for section 12.2.
Vocabulary
| Term | Definition |
|---|---|
| rotational symmetry | When a figure can be rotated (less than 360^{\circ}\)) such that it looks like it did before the rotation. The center of rotation is the point a figure is rotated around such that the rotational symmetry holds. |
| Center of Rotation | In a rotation, the center of rotation is the point that does not move. The rest of the plane rotates around this fixed point. |
| Rotation | A rotation is a transformation that turns a figure on the coordinate plane a certain number of degrees about a given point without changing the shape or size of the figure. |
| Rotation Symmetry | A figure has rotational symmetry if it can be rotated less than \(360^{\circ}\) around its center point and look exactly the same as it did before the rotation. |
| Symmetry | A figure has symmetry if it can be transformed and still look the same. |
Additional Resources
Interactive Element
Video: Rotation Symmetry Principles - Basic
Activities: Rotation Symmetry Discussion Questions
Study Aids: Symmetry and Tessellations Study Guide
Practice: Rotation Symmetry
Real World: This End Up