9.2 Parabolas
- Page ID
- 1033
When working with parabolas in the past you probably used vertex form and analyzed the graph by finding its roots and intercepts. There is another way of defining a parabola that turns out to be more useful in the real world. One of the many uses of parabolic shapes in the real world is satellite dishes. In these shapes it is vital to know where the receptor point should be placed so that it can absorb all the signals being reflected from the dish.
Where should the receptor be located on a satellite dish that is four feet wide and nine inches deep?
Graphing Parabolas
The definition of a parabola is the collection of points equidistant from a point called the focus and a line called the directrix.
Notice how the three points \(P_{1}, P_{2}, P_{3}\) are each connected by a blue line to the focus point \(F\) and the directrix line \(L\)
\(\overline{F P_{1}}=\overline{P_{1} Q_{1}}\)
\(\overline{F P_{2}}=\overline{P_{2} Q_{2}}\)
\(\overline{F P_{3}}=\overline{P_{3} Q_{3}}\)
There are two graphing equations for parabolas that will be used in this concept. The only difference is one equation graphs parabolas opening vertically and one equation graphs parabolas opening horizontally. You can recognize the parabolas opening vertically because they have an \(x^{2}\) term. Likewise, parabolas opening horizontally have a \(y^{2}\) term.
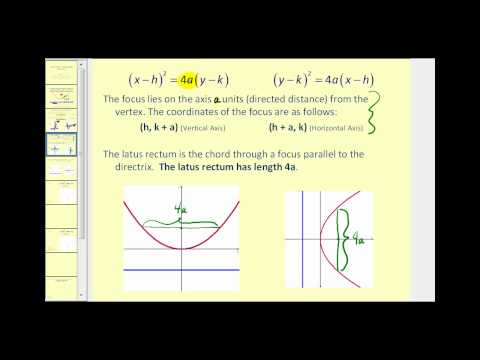
The general equation for a parabola opening vertically is \((x-h)^{2}=\pm 4 p(y-k)\). The general equation for a parabola opening horizontally is \((y-k)^{2}=\pm 4 p(x-h)\).
Note that the vertex is still \((h, k)\). The parabola opens upwards or to the right if the \(4 p\) is positive. The parabola opens down or to the left if the \(4 p\) is negative. The focus is just a point that is distance \(p\) away from the vertex. The directrix is just a line that is distance \(p\) away from the vertex in the opposite direction. You can sketch how wide the parabola is by noting the focal width is \(|4 p|\).
Once you put the parabola into this graphing form you can sketch the parabola by plotting the vertex, identifying \(p\) and plotting the focus and directrix and lastly determining the focal width and sketching the curve.
Take the conic:
\(2 x^{2}+16 x+y=0\)
This is a parabola because the \(y^{2}\) coefficient is zero.
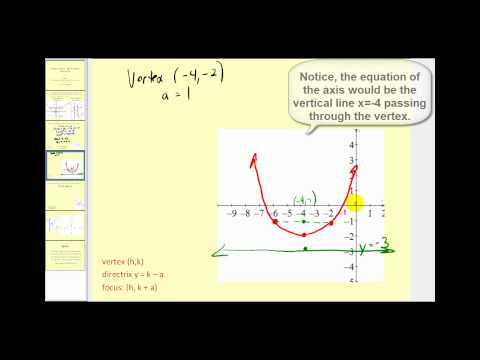
\(\begin{aligned} x^{2}+8 x &=-\frac{1}{2} y \\ x^{2}+8 x+16 &=-\frac{1}{2} y+16 \\ (x+4)^{2} &=-\frac{1}{2}(y-32) \\ (x+4)^{2} &=-4 \cdot \frac{1}{8}(y-32) \end{aligned}\)
The vertex is \((-4,32) .\) The focal length is \(p=\frac{1}{8}\). This parabola opens down which means that the focus is at \(\left(-4,32-\frac{1}{8}\right)\) and the directrix is horizontal at \(y=32+\frac{1}{8} .\) The focal width is \(\frac{1}{2}\).
Examples
Earlier, you were asked where the receptor should be located on a satellite dish that is four feet wide and nine inches deep.
Since real world problems do not come with a predetermined coordinate system, you can choose to make the vertex of the parabola at (0, 0). Then, if everything is done in inches, another point on the parabola will be (24, 9). (Many people might mistakenly believe the point (48, 9) is on the parabola but remember that half this width stretches to (-24, 9) as well.) Using these two points, the focal width can be found.
\(\begin{aligned}(x-0)^{2} &=4 p(y-0)\\(24-0)^{2} &=4 p(9-0) \\ \frac{24^{2}}{4 \cdot 9} &=p \\ 16 &=p \end{aligned}\)
The receptor should be sixteen inches away from the vertex of the parabolic dish.
Sketch the following parabola and identify the important pieces of information.
\((y+1)^{2}=4 \cdot \frac{1}{2} \cdot(x+3)\)
The vertex is at (-3,-1) . The parabola is sideways because there is a \(y^{2}\) term. The parabola opens to the right because the \(4 p\) is positive. The focal length is \(p=\frac{1}{2}\) which means the focus is \(\frac{1}{2}\) to the right of the vertex at (-2.5,-1) and the directrix is \(\frac{1}{2}\) to the left of the vertex at \(x=-3.5\). The focal width is 2 which is why the width of the parabola stretches from (-2.5,0) to (-2.5,-2) .
What is the equation of a parabola that has a focus at (4,3) and a directrix of \(y=-1 ?\)
It would probably be useful to graph the information that you have in order to reason about where the vertex is.
The vertex must be halfway between the focus and the directrix. This places it at (4, 1). The focal length is 2. The parabola opens upwards. This is all the information you need to create the equation.
\((x-4)^{2}=4 \cdot 2 \cdot(y-1)\)
OR \((x-4)^{2}=8(y-1)\)
What is the equation of a parabola that opens to the right with focal width from (6,-7) to (6,12)\(?\) The focus is in the middle of the focal width. The focus is \(\left(6, \frac{5}{2}\right)\). The focal width is 19 which is four times the focal length so the focal length must be \(\frac{19}{4}\). The vertex must be a focal length to the left of the focus, so the vertex is at \(\left(6-\frac{19}{4}, \frac{5}{2}\right)\). This is enough information to write the equation of the parabola.
\(\left(y-\frac{5}{2}\right)^{2}=4 \cdot \frac{19}{4} \cdot\left(x-6+\frac{19}{4}\right)\)
Sketch the following conic by putting it into graphing form and identifying important information.
\(y^{2}-4 y+12 x-32=0\)
\(\begin{aligned} y^{2}-4 y &=-12 x+32 \\ y^{2}-4 y+4 &=-12 x+32+4 \\ (y-2)^{2} &=-12(x-3) \\ (y-2)^{2} &=-4 \cdot 3 \cdot(x-3) \end{aligned}\)
The vertex is at (3,2) . The focus is at (0,2) . The directrix is at \(x=6\).
1. What is the equation of a parabola with focus at (1,4) and directrix at \(y=-2 ?\)
2. What is the equation of a parabola that opens to the left with focal width from (-2,5) to (-2,-7)\(?\)
3. What is the equation of a parabola that opens to the right with vertex at (5,4) and focal width of \(12 ?\)
4. What is the equation of a parabola with vertex at (1,8) and directrix at \(y=12\) ?
5. What is the equation of a parabola with focus at (-2,4) and directrix at \(x=4 ?\)
6. What is the equation of a parabola that opens downward with a focal width from (-4,9) to (16,9)\(?\)
7. What is the equation of a parabola that opens upward with vertex at (1,11) and focal width of \(4 ?\)
Sketch the following parabolas by putting them into graphing form and identifying important information.
8. \(y^{2}+2 y-8 x+33=0\)
9. \(x^{2}-8 x+20 y+36=0\)
10. \(x^{2}+6 x-12 y-15=0\)
11. \(y^{2}-12 y+8 x+4=0\)
12. \(x^{2}+6 x-4 y+21=0\)
13. \(y^{2}+14 y-2 x+59=0\)
14. \(x^{2}+12 x-\frac{8}{3} y+\frac{92}{3}=0\)
15. \(x^{2}+2 x-\frac{4}{5} y+1=0\)