9.1: Null and Alternative Hypotheses
- Page ID
- 5783
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Hypothesis Testing
Null and Alternative Hypotheses
Hypothesis testing involves testing the difference between a hypothesized value of a population parameter and the estimate of that parameter which is calculated from a sample. If the parameter of interest is the mean of the populations in hypothesis testing, we are essentially determining the magnitude of the difference between the mean of the sample and they hypothesized mean of the population. If the difference is very large, we reject our hypothesis about the population. If the difference is very small, we do not. Below is an overview of this process.
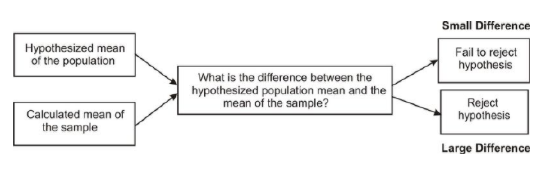
In statistics, the hypothesis to be tested is called the null hypothesis and given the symbol H0. The alternative hypothesis is given the symbol Ha
The null hypothesis defines a specific value of the population parameter that is of interest. Therefore, the null hypothesis always includes the possibility of equality. Consider
H0:μ=3.2
Ha:μ≠3.2
In this situation if our sample mean, x̄, is very different from 3.2 we would reject H0. That is, we would reject H0 if x̄ is much larger than 3.2 or much smaller than 3.2. This is called a 2-tailed test. An x̄ that is very unlikely if H0 is true is considered to be good evidence that the claim H0 is not true. Consider H0:μ≤3.2 Ha:μ>3.2. In this situation we would reject H0 for very large values of x̄. This is called a one tail test. If, for this test, our data gives x̄=15, it would be highly unlikely that finding x̄ this different from 3.2 would occur by chance and so we would probably reject the null hypothesis in favor of the alternative hypothesis.
Testing the Null Hypothesis
If we were to test the hypothesis that the seniors had a mean SAT score of 1100 our null hypothesis would be that the SAT score would be equal to 1100 or:
H0:μ=1100
We test the null hypothesis against an alternative hypothesis, which is given the symbol Ha and includes the outcomes not covered by the null hypothesis. Basically, the alternative hypothesis states that there is a difference between the hypothesized population mean and the sample mean. The alternative hypothesis can be supported only by rejecting the null hypothesis. In our example above about the SAT scores of graduating seniors, our alternative hypothesis would state that there is a difference between the null and alternative hypotheses or:
Ha:μ≠1100
Let’s take a look at examples and develop a few null and alternative hypotheses.
Developing Null and Alternative Hypotheses
1. We have a medicine that is being manufactured and each pill is supposed to have 14 milligrams of the active ingredient. What are our null and alternative hypotheses?
Solution:
H0:μ=14
Ha:μ≠14
Our null hypothesis states that the population has a mean equal to 14 milligrams. Our alternative hypothesis states that the population has a mean that is different than 14 milligrams. This is two-tailed.
2. The school principal wants to test if it is true what teachers say -- that high school juniors use the computer an average 3.2 hours a day. What are our null and alternative hypotheses?
H0:μ=3.2
Ha:μ≠3.2
Our null hypothesis states that the population has a mean equal to 3.2 hours. Our alternative hypothesis states that the population has a mean that differs from 3.2 hours. This is two-tailed.
Example
Example 1
eHealthInsurance claims that in 2011, the average monthly premium paid for individual health coverage was $183. Suppose you are suspicious that the average, or mean, cost is actually higher. State the null and alternative hypothesis you would use to test this.
The original claim from eHealthInsurance is that μ=183. Your theory is that μ>183. Since the original claim has equality in it, we'll put that in the null hypothesis.
H0:μ = 183
Ha:μ>183
Review
- If the difference between the hypothesized population mean and the mean of the sample is large, we ___ the null hypothesis. If the difference between the hypothesized population mean and the mean of the sample is small, we ___ the null hypothesis.
- At the Chrysler manufacturing plant, there is a part that is supposed to weigh precisely 19 pounds. The engineers take a sample of parts and want to know if they meet the weight specifications. What are our null and alternative hypotheses?
For 3-5, determine whether each of the following is a null or an alternative hypothesis.
- The average weight of golden retriever dogs is the same as the average weight of pit bull dogs.
- The proportion of books in the library that are novels is higher than the proportion of books in the library that are nonfiction.
- The average price of wool coats in San Francisco is lower in the summer than in the winter.
For 6-9, for each of the following write the alternative hypothesis.
- H0:p=0.30 and the test is two sided.
- H0:p=0.35 and the test is left sided.
- H0:p=0.55 and the test is right sided.
- H0:μ=500 and the test is two sided.
- Suppose the present success rate in treating a certain type of lung cancer is .75. A research group hopes to demonstrate that the success rate of a new treatment of this cancer is better. Write the null and alternative hypotheses.
Vocabulary
| Term | Definition |
|---|---|
| alternative hypothesis | The alternative hypothesis, H1, is the clear and concise statement of the initial claim. |
| Hypothesis | A hypothesis is an educated or reasonable guess based on an idea that one would like to figure out. |
| hypothesis testing | Hypothesis testing involves testing the difference between a hypothesized value of a population parameter and the estimate of that parameter which is calculated from a sample. |
| null hypothesis | The null hypothesis, Ho, is the opposite of what you are hoping to claim. |
Additional Resources
Video: Null and Alternative Hypotheses
Practice: Null and Alternative Hypotheses
Real World: In the Grippe of the Flu