2.3.4: Coterminal Angles
- Page ID
- 14353
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Set of angles with the same terminal or end side.
While playing a game with friends, you use a spinner that looks like this:

As you can see, the angle that the spinner makes with the horizontal is \(60^{\circ}\). Is it possible to represent the angle any other way?
Coterminal Angles
Consider the angle \(30^{\circ}\), in standard position.
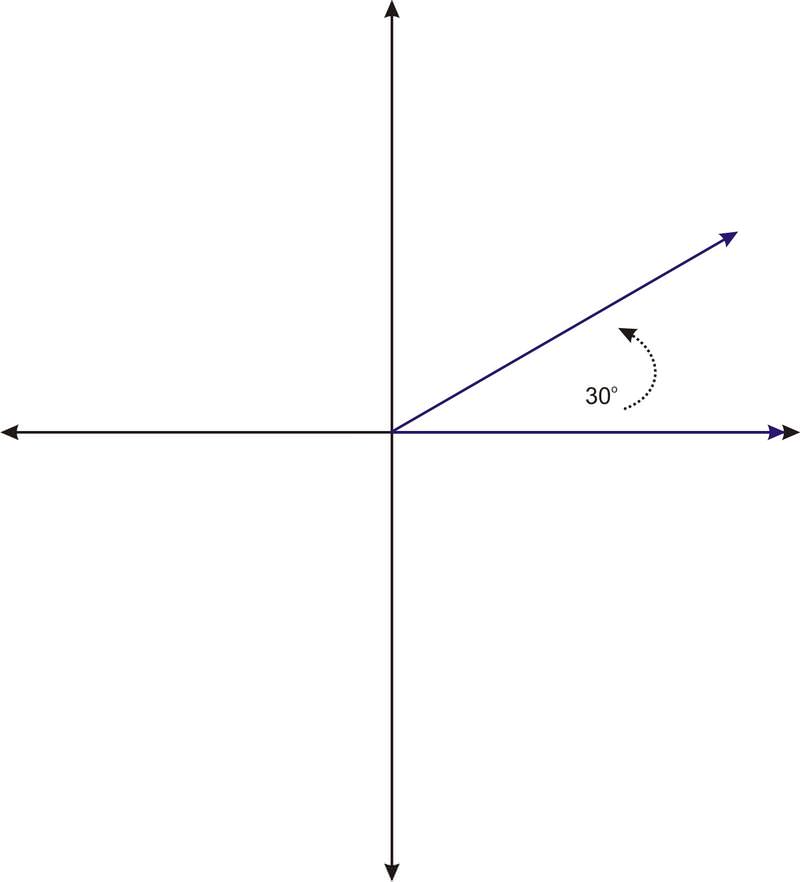
Now consider the angle \(390^{\circ}\). We can think of this angle as a full rotation (\(360^{\circ}\)), plus an additional 30 degrees.
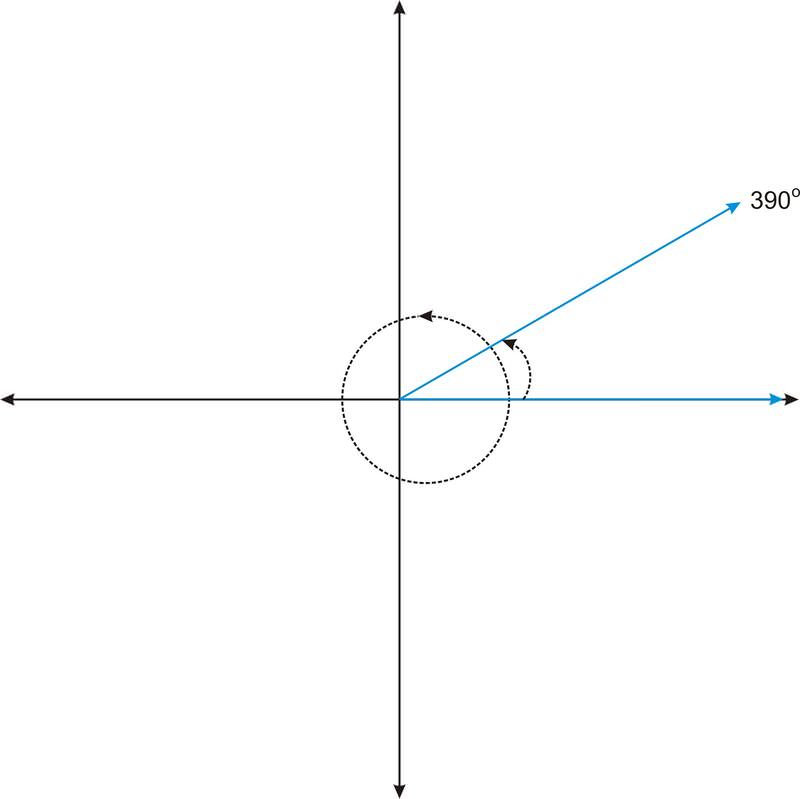
Notice that \(390^{\circ}\) looks the same as \(30^{\circ}\). Formally, we say that the angles share the same terminal side. Therefore we call the angles co-terminal. Not only are these two angles co-terminal, but there are infinitely many angles that are co-terminal with these two angles. For example, if we rotate another \(360^{\circ}\), we get the angle \(750^{\circ}\). Or, if we create the angle in the negative direction (clockwise), we get the angle \(−330^{\circ}\). Because we can rotate in either direction, and we can rotate as many times as we want, we can continuously generate angles that are co-terminal with \(30^{\circ}\).
Identifying Co-Terminal Angles
For the following questions, determine if the angle is co-terminal with \(45^{\circ}\).
1. \(−45^{\circ}\)
No, it is not co-terminal with \(45^{\circ}\)
2. \(405^{\circ}\)
Yes, \(405^{\circ}\) is co-terminal with \(45^{\circ}\).
3. \(−315^{\circ}\)
Yes, \(−315^{\circ}\) is co-terminal with \(45^{\circ}\).
Earlier, you were asked if it is possible to represent the angle any other way.
Solution
You can either think of \(60^{\circ}\) as \(420^{\circ}\) if you rotate all the way around the circle once and continue the rotation to where the spinner has stopped, or as \(−300^{\circ}\) if you rotate clockwise around the circle instead of counterclockwise to where the spinner has stopped.
Find a coterminal angle to \(23^{\circ}\)
Solution
A coterminal angle would be an angle that is at the same terminal place as \(23^{\circ}\) but has a different value. In this case, \(−337^{\circ}\) is a coterminal angle.
Find a coterminal angle to \(−90^{\circ}\)
Solution
A coterminal angle would be an angle that is at the same terminal place as −90^{\circ}\) but has a different value. In this case, \(270^{\circ}\) is a coterminal angle.
Find two coterminal angles to \(70^{\circ}\) by rotating in the positive direction around the circle.
Solution
Rotating once around the circle gives a coterminal angle of \(430^{\circ}\). Rotating again around the circle gives a coterminal angle of \(790^{\circ}\).
Review
- Is \(315^{\circ}\) co-terminal with \(−45^{\circ}\)?
- Is \(90^{\circ}\) co-terminal with \(−90^{\circ}\)?
- Is \(350^{\circ}\) co-terminal with \(−370^{\circ}\)?
- Is \(15^{\circ}\) co-terminal with \(1095^{\circ}\)?
- Is \(85^{\circ}\) co-terminal with \(1880^{\circ}\)?
For each diagram, name the angle in 3 ways. At least one way should use negative degrees.
-

Figure \(\PageIndex{4}\) -
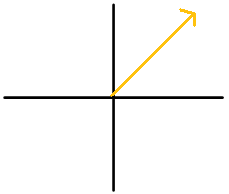
Figure \(\PageIndex{5}\) -

Figure \(\PageIndex{6}\) -
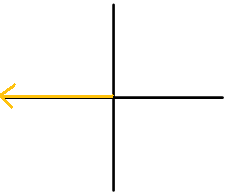
Figure \(\PageIndex{7}\) -
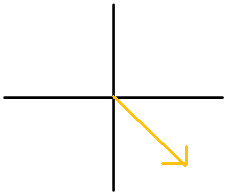
Figure \(\PageIndex{8}\) - Name the angle of the 8 on a standard clock two different ways.
- Name the angle of the 11 on a standard clock two different ways.
- Name the angle of the 4 on a standard clock two different ways.
- Explain how to determine whether or not two angles are co-terminal.
- How many rotations is \(4680^{\circ}\)?
Review (Answers)
To see the Review answers, open this PDF file and look for section 1.16.
Vocabulary
| Term | Definition |
|---|---|
| Coterminal Angles | A set of coterminal angles are angles with the same terminal side but expressed differently, such as a different number of complete rotations around the unit circle or angles being expressed as positive versus negative angle measurements. |
Additional Resources
Video: Example: Determine if Two Angles are Coterminal
Practice: Coterminal Angles

