3.5.3: Triple-Angle Formulas and Linear Combinations
- Page ID
- 4241
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Combination of the sum and double angle formulas; set of terms added or subtracted with a constant multiplier.
In other lessons you've dealt with double angle formulas. This was useful for finding the value of an angle that was double your well known value. Now consider the idea of a "triple angle formula". If someone gave you a problem like this:
\(\sin 135^{\circ}\)
Could you compute its value?
Triple Angle Formulas and Linear Combinations
Double angle formulas are great for computing the value of a trig function in certain cases. However, sometimes different multiples than two times and angle are desired. For example, it might be desirable to have three times the value of an angle to use as the argument of a trig function.
By combining the sum formula and the double angle formula, formulas for triple angles and more can be found.
Here, we take an equation which takes a linear combination of sine and cosine and converts it into a simpler cosine function.
\(A\cos x+B\sin x=C\cos (x−D)\), where \(C=\sqrt{A^2+B^2}\), \(\cos D=\dfrac{A}{C}\) and \(\sin D=\dfrac{B}{C}\).
You can also use the TI-83 to solve trigonometric equations. It is sometimes easier than solving the equation algebraically. Just be careful with the directions and make sure your final answer is in the form that is called for. You calculator cannot put radians in terms of \pi .
Finding Formulas
Find the formula for \(\sin 3x\)
Use both the double angle formula and the sum formula.
\(\begin{aligned} \sin 3x&=\sin (2x+x) \\ &=\sin (2x)\cos x+\cos (2x)\sin x \\&=(2\sin x\cos x)\cos x+(\cos 2x−\sin 2x)\sin x \\ &=2\sin x\cos 2x+\cos 2x\sin x−\sin 3x \\&=3\sin x\cos 2x−\sin 3x \\&=3\sin x(1−\sin 2x)−\sin 3x \\&=3\sin x−4\sin 3x \end{aligned}\)
Performing Transformations
Transform \(3\cos 2x−4\sin 2x\) into the form \(C\cos (2x−D)\)
\(A=3\) and \(B=−4\), so \(C=\sqrt{3^2+(−4)^2}=5\). Therefore \(\cos D=\dfrac{3}{5}\) and \(\sin D=−\dfrac{4}{5}\) which makes the reference angle is \(−53.1^{\circ}\) or \(−0.927\) radians. Since cosine is positive and sine is negative, the angle must be a fourth quadrant angle. \(D\) must therefore be \(306.9^{\circ} \) or \(5.36\) radians. The final answer is \(3\cos 2x−4\sin 2x=5\cos (2x−5.36)\).
Solving for Unknown Values
Solve \(\sin x=2\cos x\) such that \(0\leq x\leq 2\pi\) using a graphing calculator.
Solution: In \(y=\), graph \(y_1=\sin x\) and \(y_2=2\cos x\).
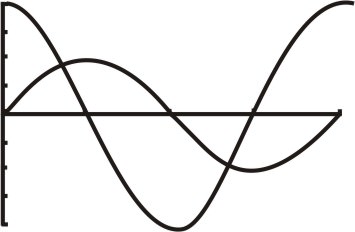
Next, use CALC to find the intersection points of the graphs.

Earlier, you were asked to solve sin \(135^{\circ} \).
Solution
Using the triple angle formula we learned in this lesson for the sine function, we can break the angle down into three times a well known angle:
\(\sin 3x=3\sin x−4\sin ^3x\)
we can solve this problem.
\(\begin{aligned} \sin (3×45^{\circ} )&=3\sin 45^{\circ} −4\sin ^3 45^{\circ} \\&=3\dfrac{\sqrt{2} }{2}−4\left(\dfrac{\sqrt{2} }{2}\right)^3 \\ &=3\dfrac{\sqrt{2} }{2}−\left(\dfrac{4(2)^{2/3}}{8}\right) \\ &=\dfrac{3\sqrt{2} −2\sqrt{2} }{2} \\ &=\dfrac{\sqrt{2} }{2} \end{aligned}\)
Transform \(5\cos x−5\sin x\) to the form \(C\cos (x−D)\)
Solution
If \(5\cos x−5\sin x\), then \(A=5\) and \(B=−5\). By the Pythagorean Theorem, \(C=5\sqrt{2}\) and \(\cos D=55\sqrt{2} =1\sqrt{2} =\dfrac{\sqrt{2} }{2}\). So, because \(B\) is negative, \(D\) is in Quadrant IV. Therefore, \(D=\dfrac{7\pi }{4}\). Our final answer is \(5\sqrt{2} \cos \left(x−\dfrac{7\pi }{4}\right)\).
Transform \(−15\cos 3x−8\sin 3x\) to the form \(C\cos (x−D)\)
Solution
If \(−15\cos 3x−8\sin 3x\), then \(A=−15\) and \(B=−8\). By the Pythagorean Theorem, \(C=17\). Because \(A\) and \(B\) are both negative, \(D\) is in Quadrant III, which means \(D=\cos ^{−1} \left(\dfrac{15}{17}\right)=0.49+\pi =3.63 \text{ rad}\). Our final answer is \(17\cos 3(x−3.63)\).
Derive a formula for \(\tan 4x\).
Solution
\(\begin{aligned}
\tan 4 x &=\tan (2 x+2 x) \\
&=\dfrac{\tan 2 x+\tan 2 x}{1-\tan 2 x \tan 2 x} \\
&=\dfrac{2 \tan 2 x}{1-\tan ^{2} 2 x} \\
&=\dfrac{2 \cdot \dfrac{2 \tan x}{1-\tan ^{2} x}}{1-\left(\dfrac{2 \tan x}{1-\tan ^{2} x}\right)^{2}} \\
&=\dfrac{4 \tan x}{1-\tan ^{2} x} \div \dfrac{\left(1-\tan ^{2} x\right)^{2}-4 \tan ^{2} x}{\left(1-\tan ^{2} x\right)^{2}} \\
&=\dfrac{4 \tan x}{1-\tan ^{2} x} \div \dfrac{1-2 \tan ^{2} x+\tan ^{4} x-4 \tan ^{2} x}{\left(1-\tan ^{2} x\right)^{2}} \\
&=\dfrac{4 \tan x}{1-\tan ^{2} x} \cdot \dfrac{\left(1-\tan ^{2} x\right)^{2}}{1-6 \tan ^{2} x+\tan ^{4} x} \\
&=\dfrac{4 \tan x-4 \tan ^{3} x}{1-6 \tan ^{2} x+\tan ^{4} x}
\end{aligned}\)
Review
Transform each expression to the form \( C \cos (x−D)\).
- \(3\cos x−2\sin x\)
- \(2\cos x−\sin x\)
- \(−4\cos x+5\sin x\)
- \(7\cos x−6\sin x\)
- \(11\cos x+9\sin x\)
- \(14\cos x+2\sin x\)
- \(−2\cos x−4\sin x\)
Derive a formula for each expression.
- \(\sin 4x\)
- \(\cos 6x\)
- \(\cos 4x\)
- \(\csc 2x\)
- \(\cot 2x\)
Find all solutions to each equation in the interval \([0,2\pi )\).
- \(\cos x+\cos 3x=0\)
- \(\sin 2x=\cos 3x\)
- \(\cos 2x+\cos 4x=0\)
Review (Answers)
To see the Review answers, open this PDF file and look for section 3.15.
Vocabulary
| Term | Definition |
|---|---|
| Linear Combination | A linear combination is a set of terms that are added or subtracted from each other with a multiplicative constant in front of each term. |
| Triple Angle Identity | A triple angle identity (also referred to as a triple angle formula) relates a trigonometric function of three times an argument to a set of trigonometric functions, each containing the original argument. Examples include: the Triple Angle Formula for sine \(\sin (3\theta )=3\sin \theta −4\sin ^3\theta \), the Triple Angle Formula for cosine \(\cos (3\theta )=−3 \cos \theta +4 \cos ^3\theta \), and the Triple Angle Formula for tangent \(\tan (3\theta )=\dfrac{3 \tan \theta − \tan ^3\theta }{1−3 \tan ^2\theta }\). |

