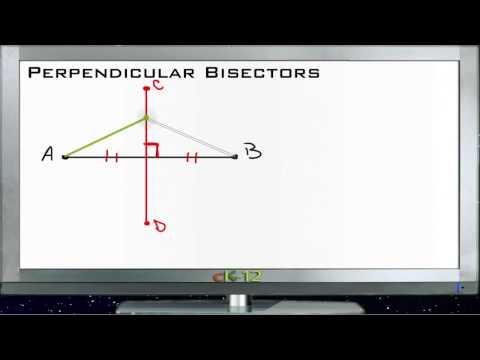
4.20: Perpendicular Bisectors
- Page ID
- 4817
Intersect line segments at their midpoints and form 90 degree angles with them.
Perpendicular Bisector Theorem
A perpendicular bisector is a line that intersects a line segment at its midpoint and is perpendicular to that line segment, as shown in the construction below.
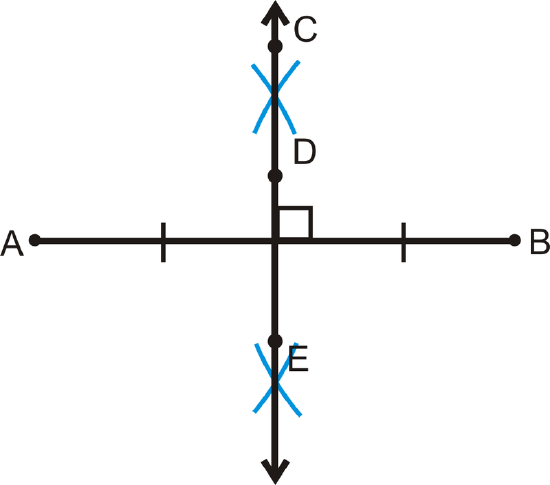
One important property related to perpendicular bisectors is that if a point is on the perpendicular bisector of a segment, then it is equidistant from the endpoints of the segment. This is called the Perpendicular Bisector Theorem.
If \(\overleftrightarrow{CD}\perp \overline{AB}\) and \(AD=DB\), then \(AC=CB\).
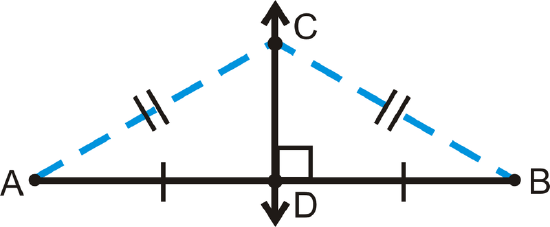
In addition to the Perpendicular Bisector Theorem, the converse is also true.
Perpendicular Bisector Theorem Converse: If a point is equidistant from the endpoints of a segment, then the point is on the perpendicular bisector of the segment.
Using the picture above: If \(AC=CB\), then \(\overleftrightarrow{CD}\perp \overline{AB}\) and \(AD=DB\).
When we construct perpendicular bisectors for the sides of a triangle, they meet in one point. This point is called the circumcenter of the triangle.
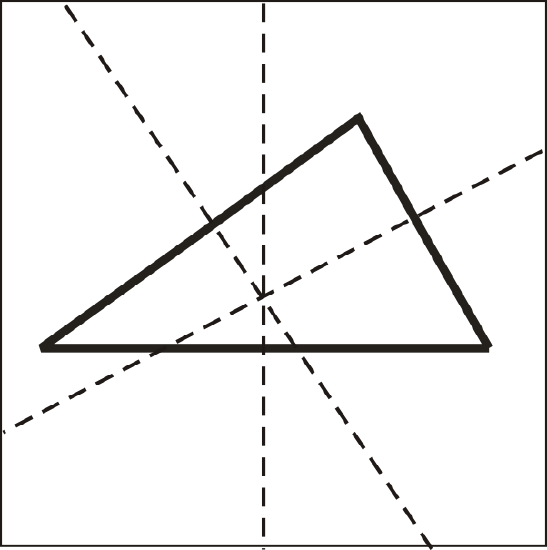
What if you were given \(\Delta FGH\) and told that \(\overleftrightarrow{GJ}\) was the perpendicular bisector of \(\overline{FH}\)? How could you find the length of FG given the length of GH\)?
Example \(\PageIndex{1}\)
\(\overleftrightarrow{OQ}\) is the perpendicular bisector of \(\overline{MP}\).
Which line segments are equal? Find \(x\). Is \(L\) on \(\overleftrightarrow{OQ}\)? How do you know?
Solution
\(ML=LP\), \(MO=OP\), and \(MQ=QP\).
\(\begin{align*} 4x+3&=11 \\ 4x&=8 \\ x&=2\end{align*} \)
Yes, \(L\) is on \(\overleftrightarrow{OQ}\) because \(ML=LP\) (the Perpendicular Bisector Theorem Converse).
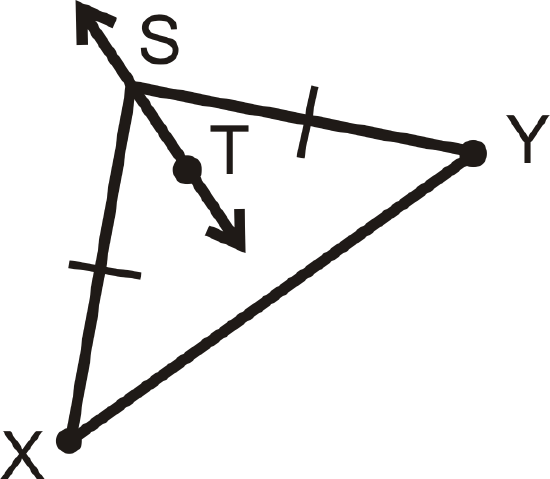
Example \(\PageIndex{2}\)
Determine if \(\overleftrightarrow{ST}\) is the perpendicular bisector of \(\overline{XY}\). Explain why or why not.
Solution
\(\overleftrightarrow{ST}\) is not necessarily the perpendicular bisector of \(\overline{XY}\) because not enough information is given in the diagram. There is no way to know from the diagram if \(\overleftrightarrow{ST}\) will extend to make a right angle with \(\overline{XY}\).
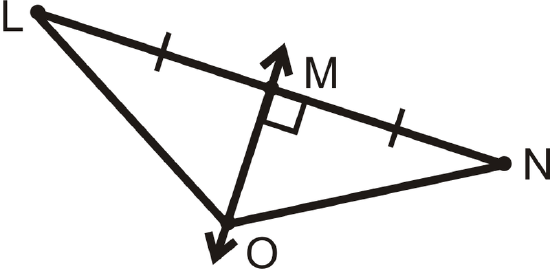
Example \(\PageIndex{3}\)
If \(\overleftrightarrow{MO}\)− is the perpendicular bisector of \(\overline{LN}\) and \(LO=8\), what is \(ON\)?
Solution
By the Perpendicular Bisector Theorem, \(LO=ON\). So, \(ON=8\).
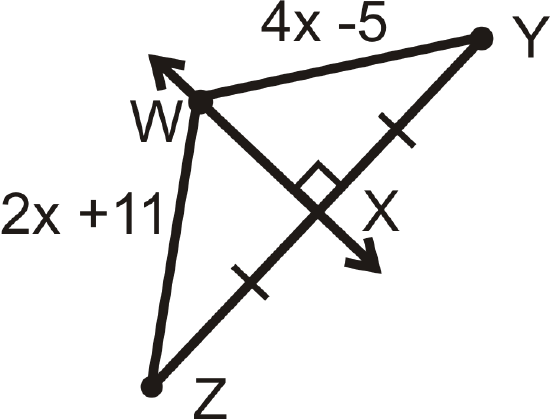
Example \(\PageIndex{4}\)
Find \(x\) and the length of each segment.
Solution
\(\overleftrightarrow{WX}\)− is the perpendicular bisector of \(\overline{XZ}\) and from the Perpendicular Bisector Theorem \(WZ=WY\).
\(\begin{align*} 2x+11&=4x−5 \\ 16&=2x \\ 8&=x \end{align*}\)
\(WZ=WY=2(8)+11=16+11=27\).
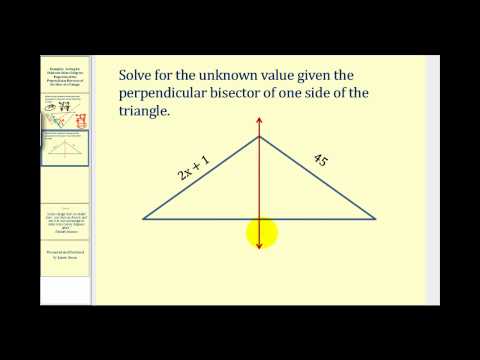
Example \(\PageIndex{5}\)
Find the value of \(x\). \(m\) is the perpendicular bisector of \(AB\).
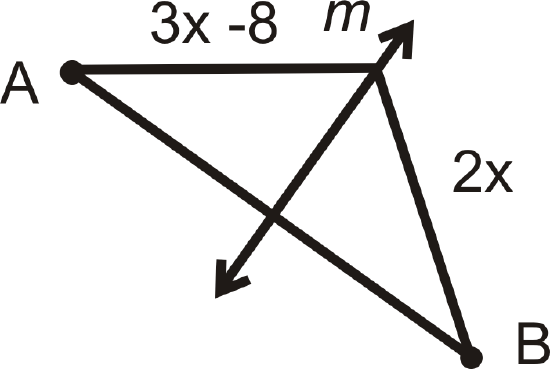
Solution
By the Perpendicular Bisector Theorem, both segments are equal. Set up and solve an equation.
\(\begin{align*}3x−8&=2x \\ x&=8 \end{align*} \)
Review
For questions 1-4, find the value of \(x\). m\) is the perpendicular bisector of\( AB\).
-
Figure \(\PageIndex{9}\) -
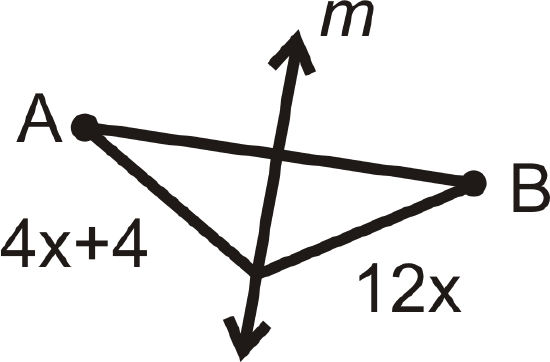
Figure \(\PageIndex{10}\) -
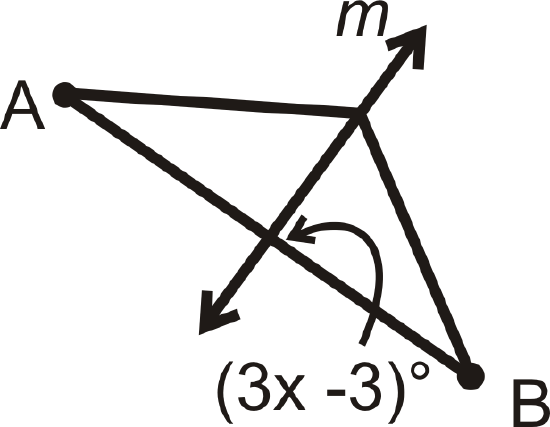
Figure \(\PageIndex{11}\) -
Figure \(\PageIndex{12}\)
m is the perpendicular bisector of \(\overline{AB}\).
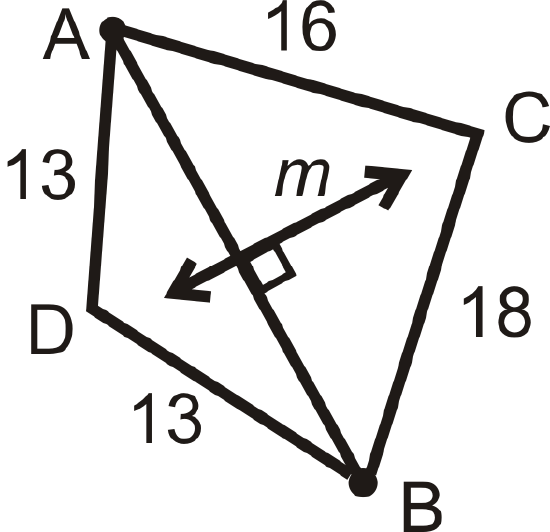
- List all the congruent segments.
- Is \(C\) on \(m\)? Why or why not?
- Is \(D\) on \(m\)? Why or why not?
For Question 8, determine if \(\overleftrightarrow{ST}\) is the perpendicular bisector of \overline{XY}\). Explain why or why not.
-
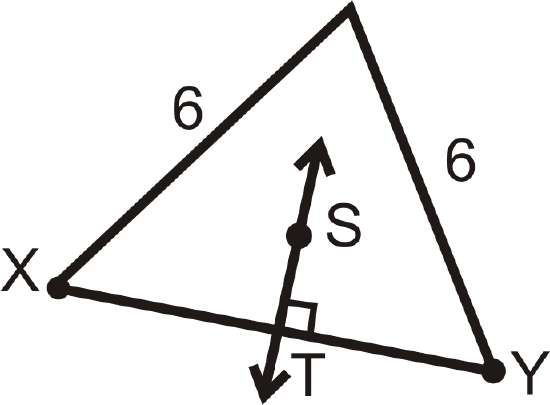Figure \(\PageIndex{14}\) - In what type of triangle will all perpendicular bisectors pass through vertices of the triangle?
- Fill in the blanks of the proof of the Perpendicular Bisector Theorem.
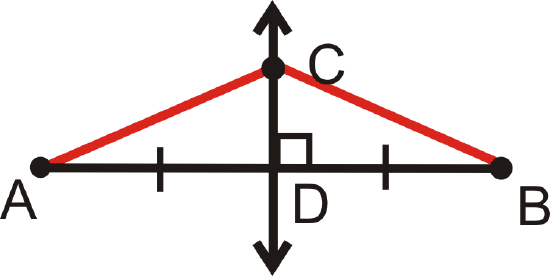
Figure \(\PageIndex{15}\)
Given: \(\overleftrightarrow{CD}\) is the perpendicular bisector of \(\overline{AB}\)
Prove: \(\overline{AC}\cong \overline{CB}\)
| Statement | Reason |
|---|---|
| 1. | 1. |
| 2. \(D\) is the midpoint of \(\overline{AB}\) | 2. |
| 3. | 3. Definition of a midpoint |
| 4. \(\angle CDA\) and \(\angle CDB\) are right angles | 4. |
| 5. \(\angle CDA\cong \angle CDB\) | 5. |
| 6. | 6. Reflexive PoC |
| 7. \(\Delta CDA\cong \Delta CDB\) | 7. |
| 8. \(\overline{AC}\cong \overline{CB}\) | 8. |
Review (Answers)
To see the Review answers, open this PDF file and look for section 5.2.
Vocabulary
| Term | Definition |
|---|---|
| circumcenter | The circumcenter is the point of intersection of the perpendicular bisectors of the sides in a triangle. |
| perpendicular bisector | A perpendicular bisector of a line segment passes through the midpoint of the line segment and intersects the line segment at \(90^{\circ}\). |
| Perpendicular Bisector Theorem Converse | If a point is equidistant from the endpoints of a segment, then the point is on the perpendicular bisector of the segment. |
Additional Resources
Interactive Element
Video: Perpendicular Bisectors Principles - Basic
Activites: Perpendicular Bisectors Discussion Questions
Study Aid: Bisectors, Medians, Altitudes Study Guide
Practice: Perpendicular Bisectors
Real World: Perpendicular Bisectors