5.3: Square and Rectangle Area and Perimeter
- Page ID
- 2155
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Compute edge and coverage measures of rectilinear quadrilaterals, given linear measures.
Area and Perimeter of Rectangles
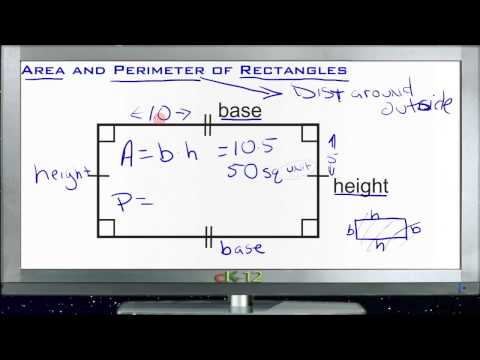
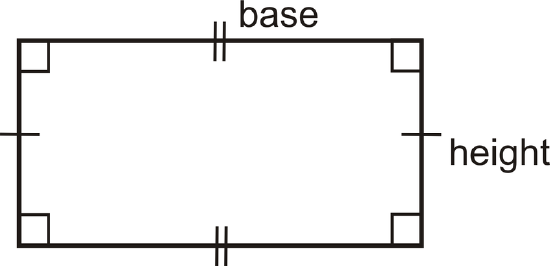
To find the area of a rectangle, calculate \(A=bh\), where \(b\) is the base (width) and \(h\) is the height (length). The perimeter of a rectangle will always be \(P=2b+2h\).
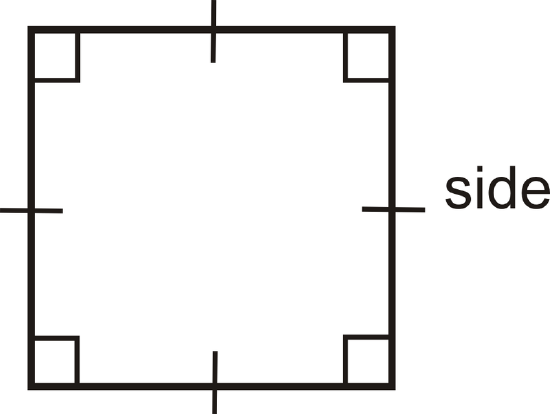
If a rectangle is a square, with sides of length s, then perimeter is \(P_{square}=2s+2s=4s\) and area is \(A_{sqaure}=s\cdot s=s^2\).
What if you were given a rectangle and the size of its base and height? How could you find the total distance around the rectangle and the amount of space it takes up?
Example \(\PageIndex{1}\)
The area of a square is \(75\text{ in}^2\). Find the perimeter.
Solution
To find the perimeter, we need to find the length of the sides.
\(\begin{aligned} A&=s^2=75\text{ in}^2 \\ s&=\sqrt{75}=5\sqrt{3}\text{ in } \end{aligned}\)
From this, \(P=4(5\sqrt{3})=20\sqrt{3}\text{ in }\)
Example \(\PageIndex{2}\)
Draw two different rectangles with an area of \(36\text{ cm^2 }\).
Solution
Think of all the different factors of 36. These can all be dimensions of the different rectangles.
Other possibilities could be \(6\times 6\), \(2\times 18\), and \(1\times 36\).
Example \(\PageIndex{3}\)
Find the area and perimeter of a rectangle with sides \(4\text{ cm }\) by \(9\text{ cm }\).
Solution
The perimeter is \(4+9+4+9=26\text{ cm }\). The area is \(A=9\cdot 4=36\text{ cm}^2\).
Example \(\PageIndex{4}\)
Find the area and perimeter of a square with side \(5\text{ in }\).
Solution
The perimeter is \(4(5)=20\:in\) and the area is \(5^2=25\text{ in}^2\).
Example \(\PageIndex{5}\)
Find the area and perimeter of a rectangle with sides \(13\text{ m }\) and \(12\text{ m}^2\).
Solution
The perimeter is \(2(13)+2(12)=50\text{ m }\). The area is \(13(12)=156\text{ m}^2\).
Review
- Find the area and perimeter of a square with sides of length \(12\text{ in }\).
- Find the area and perimeter of a rectangle with height of \(9\text{ cm }\) and base of \(16\text{ cm }\).
- Find the area and perimeter of a rectangle if the height is 8 and the base is 14.
- Find the area and perimeter of a square if the sides are \(18\text{ ft }\).
- If the area of a square is \(81\text{ ft}^2\), find the perimeter.
- If the perimeter of a square is \(24\text{ in }\), find the area.
- The perimeter of a rectangle is 32. Find two different dimensions that the rectangle could be.
- Draw two different rectangles that haven an area of \(90\text{ mm}^2\).
- True or false: For a rectangle, the bigger the perimeter, the bigger the area.
- Find the perimeter and area of a rectangle with sides \(17\text{ in }\) and \(21\text{ in }\).
Vocabulary
| Term | Definition |
|---|---|
| area | The amount of space inside a figure. Area is measured in square units. |
| perimeter | The distance around a shape. The perimeter of any figure must have a unit of measurement attached to it. If no specific units are given (feet, inches, centimeters, etc), write units. |
| Area of a Rectangle | To find the area '\(A\)' of a rectangle, calculate \(A = bh\), where \(b\) is the base (width) and h is the height (length). |
| Perimeter of a Rectangle | The perimeter '\(P\)' of a rectangle is equal to twice the base added to twice the height: \(P = 2b + 2h\). |
Additional Resources
Interactive Element
Video: Determine the Area of a Rectangle Involving Whole Numbers
Activities: Area and Perimeter of Rectangles Discussion Questions
Study Aids: Triangles and Quadrilaterals Study Guide
Practice: Square and Rectangle Area and Perimeter
Real World: Perimeter