2.1.1: Right Triangle Trigonometry
( \newcommand{\kernel}{\mathrm{null}\,}\)
Sine, cosine, tangent, and other ratios of sides of a right triangle.
Sine, Cosine, and Tangent
Trigonometry is the study of the relationships between the sides and angles of right triangles. The legs are called adjacent or opposite depending on which acute angle is being used.
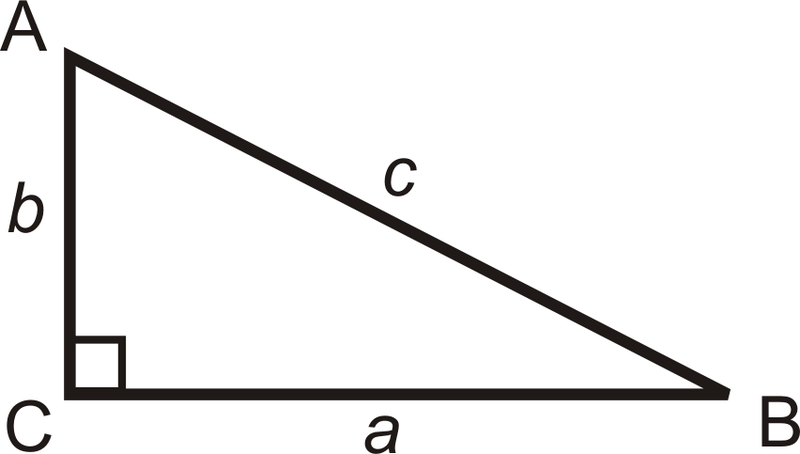
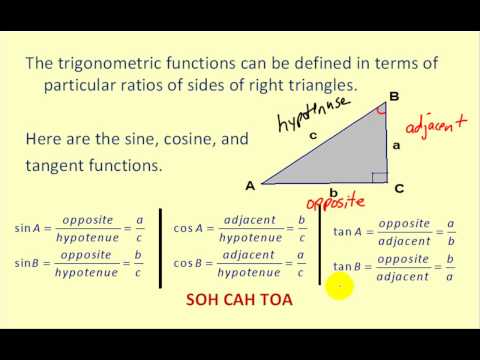
a is adjacent to ∠Ba is opposite ∠Ab is adjacent to ∠Ab is opposite ∠Bc is the hypotenuse
The three basic trigonometric ratios are called sine, cosine and tangent. For right triangle △ABC, we have:
sine Ratio: opposite leghypotenusesinA=ac or sinB=bc cosine Ratio: adjacent leghypotenusecosA=bc or cosB=ac Tangent Ratio: opposite legadjacent legtanA=ab or tanB=ba
An easy way to remember ratios is to use SOH-CAH-TOA.

A few important points:
- Always reduce ratios (fractions) when you can.
- Use the Pythagorean Theorem to find the missing side (if there is one).
- If there is a radical in the denominator, rationalize the denominator.
What if you were given a right triangle and told that its sides measure 3, 4, and 5 inches? How could you find the sine, cosine, and tangent of one of the triangle's non-right angles?
Find the sine, cosine and tangent ratios of ∠A.

Solution
First, we need to use the Pythagorean Theorem to find the length of the hypotenuse.
52+122=c213=c
sinA=leg opposite ∠Ahypotenuse=1213cosA=leg adjacent to ∠Ahypotenuse=513,tanA=leg opposite ∠Aleg adjacent to ∠A=125
Find the sine, cosine, and tangent of ∠B.
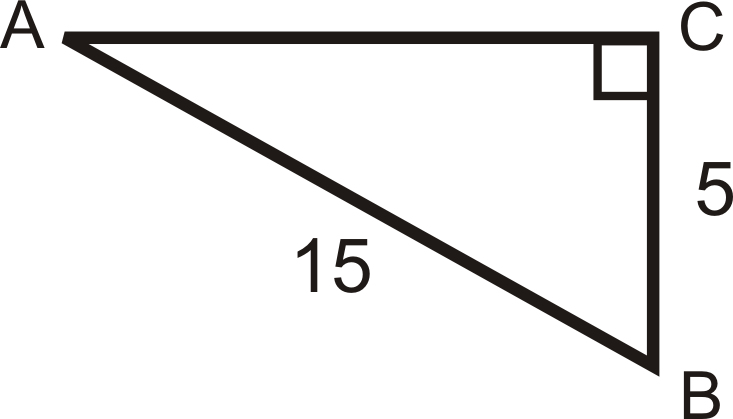
Find the length of the missing side.
Solution
AC2+52=152AC2=200AC=10√2
sinB=10√215=2√23cosB=515=13tanB=10√25=2√2
Find the sine, cosine and tangent of 30∘.
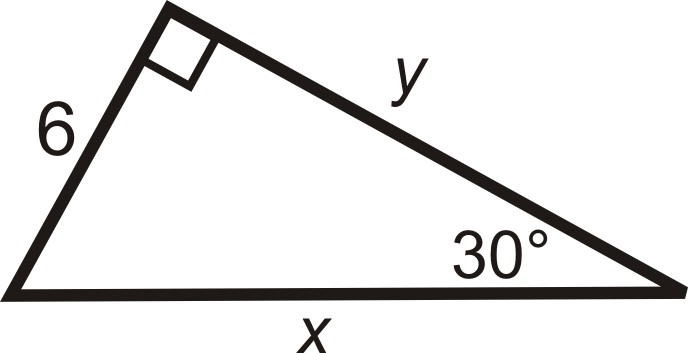
Solution
This is a 30-60-90 triangle. The short leg is 6, y=6√3 and x=12.
sin30∘=612=12cos30∘=6√312=√32tan30∘=66√3=1√3⋅√3√3=√33
Answer the questions about the following image. Reduce all fractions.
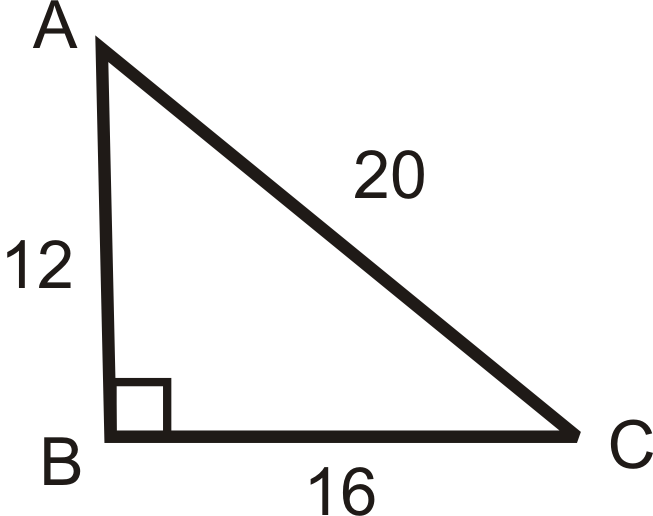
What is sinA, cosA, and tanA?
Solution
sinA=1620=45cosA=1220=35tanA=1612=43
Review
Use the diagram to fill in the blanks below.
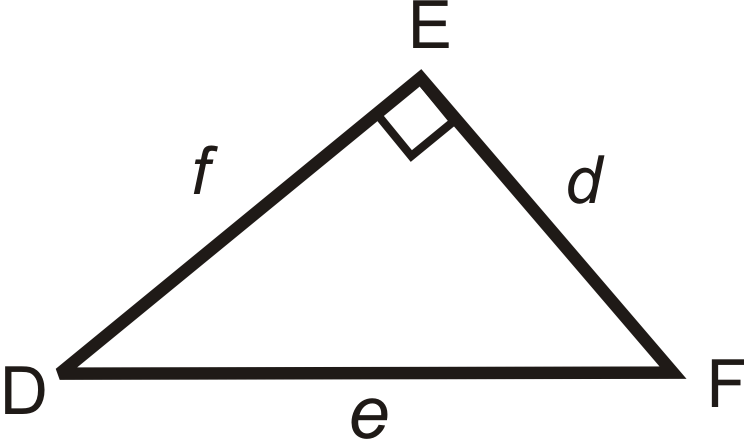
- tanD=??
- sinF=??
- tanF=??
- cosF=??
- sinD=??
- cosD=??
From questions 1-6, we can conclude the following. Fill in the blanks.
- cos_=sinF and sin_=cosF.
- tanD and tanF are _________ of each other.
Find the sine, cosine and tangent of ∠A. Reduce all fractions and radicals.
-
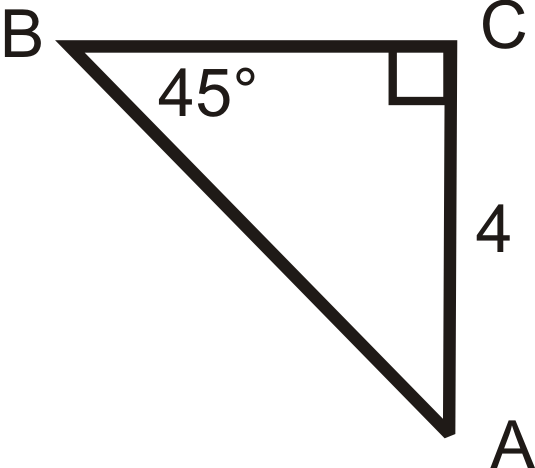
Figure 2.1.1.8 -
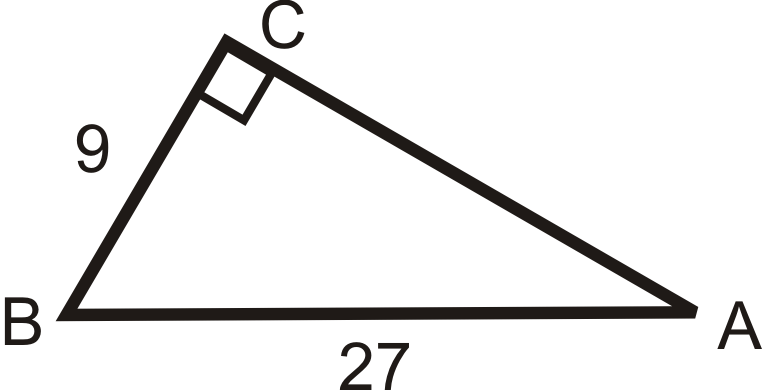
Figure 2.1.1.9 -
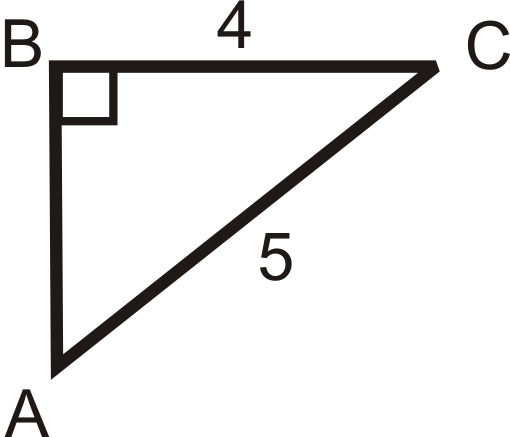
Figure 2.1.1.10 -
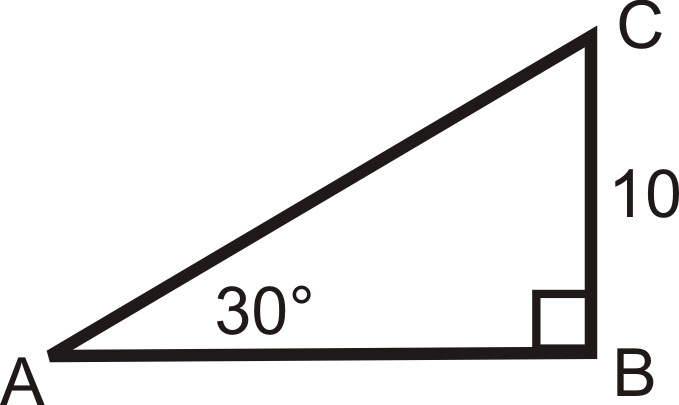
Figure 2.1.1.11 -
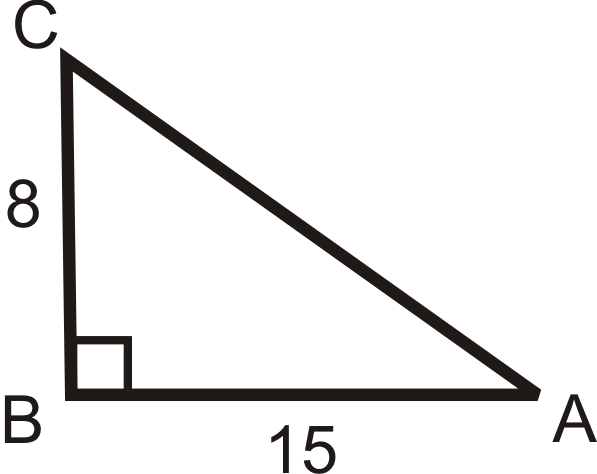 Figure \(\PageIndex{12}\)
Figure \(\PageIndex{12}\)
Review (Answers)
To see the Review answers, open this PDF file and look for section 8.7.
Resources
Vocabulary
| Term | Definition |
|---|---|
| Acute Angle | An acute angle is an angle with a measure of less than 90 degrees. |
| Adjacent Angles | Two angles are adjacent if they share a side and vertex. The word 'adjacent' means 'beside' or 'next-to'. |
| Hypotenuse | The hypotenuse of a right triangle is the longest side of the right triangle. It is across from the right angle. |
| Legs of a Right Triangle | The legs of a right triangle are the two shorter sides of the right triangle. Legs are adjacent to the right angle. |
| opposite | The opposite of a number x is −x. A number and its opposite always sum to zero. |
| Pythagorean Theorem | The Pythagorean Theorem is a mathematical relationship between the sides of a right triangle, given by a2+b2=c2, where a and b are legs of the triangle and c is the hypotenuse of the triangle. |
| Radical | The \boldsymbol{\sqrt}, or square root, sign. |
| sine | The sine of an angle in a right triangle is a value found by dividing the length of the side opposite the given angle by the length of the hypotenuse. |
| Trigonometric Ratios | Ratios that help us to unders\tan d the relationships between sides and angles of right triangles. |
Additional Resources
Video: Introduction to Trigonometric Functions U\sin g Triangles
Activities: Sine, cosine, Tangent Discussion Questions
Study Aids: Trigonometric Ratios Study Guide
Practice: Right Triangle Trigonometry
Real World: Sine cosine Tangent