Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

2.8: Truth Tables

Last updated

Dec 13, 2020
Save as PDF
- 2.7: Deductive Reasoning
- 2.9: And and Or Statements

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

So far we know these symbols for logic:

$\sim$ not (negation)
$\rightarrow$ if-then
$\therefore$ therefore

Two more symbols are:

$\wedge$ and
$\lor$ or

We would write “ $p$ and $q$ ” as $p\wedge q$ and “ $p$ or $q$ ” as $p\lor q$ .

Truth tables use these symbols and are another way to analyze logic. First, let’s relate p and \sim p. To make it easier, set p as: An even number. Therefore, \sim p is An odd number. Make a truth table to find out if they are both true. Begin with all the “truths” of p, true (T) or false (F).

p
T
F

Next we write the corresponding truth values for $\sim p$ . $\sim p$ has the opposite truth values of $p$ . So, if $p$ is true, then $\sim p$ is false and vise versa.

p	\sim p
T	F
F	T

To Recap:

Start truth tables with all the possible combinations of truths. For 2 variables there are 4 combinations for 3 variables there are 8. You always start a truth table this way.
Do any negations on the any of the variables.
Do any combinations in parenthesis.
Finish with completing what the problem was asking for.

Drawing a Truth Table

1. Draw a truth table for $p$ , $q$ and $p \wedge q$ .

First, make columns for p and q. Fill the columns with all the possible true and false combinations for the two.

p	q
T	T
T	F
F	T
F	F

Notice all the combinations of p and q. Anytime we have truth tables with two variables, this is always how we fill out the first two columns.

Next, we need to figure out when $p\wedge q$ is true, based upon the first two columns. p \wedge q can only be true if BOTH p and q are true. So, the completed table looks like this:

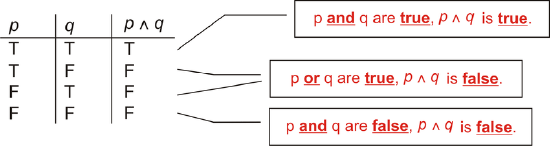f-d_b2b66563e17a9e7be0769a81e23555c42f9447ac8b6afba5c59a0c06+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{1}$

This is how a truth table with two variables and their “and” column is always filled out.

2. Draw a truth table for $p$ , $q$ and $p \lor q$ .

First, make columns for $p \lor q$ and $q$ , just like Example A.

p	q
T	T
T	F
F	T
F	F

Next, we need to figure out when $p \lor q$ is true, based upon the first two columns. $p \lor q$ is true if $p$ OR $q$ are true, or both are true. So, the completed table looks like this:

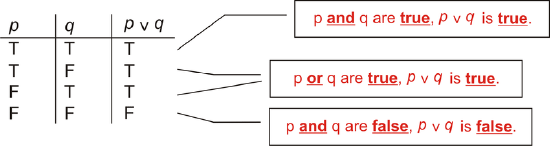f-d_f8b2daef3a483387836765fa06a98a5efcfc397b752608ef4f947790+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{2}$

The difference between $p \wedge q$ and $p \lor q$ is the second and third rows. For “and” both $p$ and $q$ have to be true, but for “or” only one has to be true.

Determining the Truths of Variables

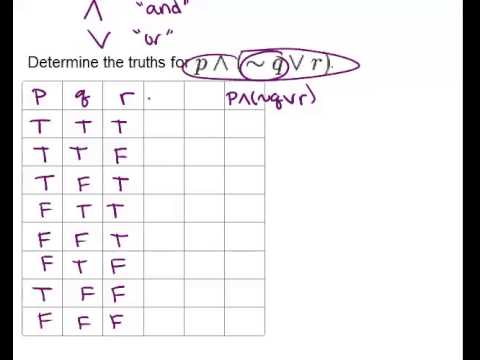
Determine the truths for $p \wedge(\sim q \lor r)$ .

First, there are three variables, so we are going to need all the combinations of their truths. For three variables, there are always 8 possible combinations.

$p$	$q$	$r$
T	T	T
T	T	F
T	F	T
T	F	F
F	T	T
F	T	F
F	F	T
F	F	F

Next, address the $\sim q$ . It will just be the opposites of the $q$ column.

$p$	$q$	$r$	$\sim q$
T	T	T	F
T	T	F	F
T	F	T	T
T	F	F	T
F	T	T	F
F	T	F	F
F	F	T	T
F	F	F	T

Now, let’s do what’s in the parenthesis, $\sim q\lor r$ . Remember, for “or” only $\sim q$ OR $r$ has to be true. Only use the $\sim q$ and $r$ columns to determine the values in this column.

$p$	$q$	$r$	$\sim q$	$\sim q\lor r$
T	T	T	F	T
T	T	F	F	F
T	F	T	T	T
T	F	F	T	T
F	T	T	F	T
F	T	F	F	F
F	F	T	T	T
F	F	F	T	T

Finally, we can address the entire problem, $p \wedge(\sim q \lor r)$ . Use the $p$ and $\sim q\lor r$ to determine the values. Remember, for “and” both $p$ and $\sim q\lor r$ must be true.

$p$	$q$	$r$	$\sim q$	$\sim q\lor r$	$p \wedge(\sim q \lor r)$
T	T	T	F	T	T
T	T	F	F	F	F
T	F	T	T	T	T
T	F	F	T	T	T
F	T	T	F	T	F
F	T	F	F	F	F
F	F	T	T	T	F
F	F	F	T	T	F

Write a truth table for the following variables.

Example $\PageIndex{1}$

$p \wedge \sim p$

Solution

First, make columns for $p$ , then add in $\sim p$ and finally, evaluate $p \wedge \sim p$ .

$p$	$\sim p$	$p \wedge \sim p$
T	F	F
F	T	F

Example $\PageIndex{2}$

$\sim p \lor \sim q$

Solution

First, make columns for $p$ and $q$ , then add in $\sim p$ and $\sim q$ . Finally, evaluate $\sim p \lor \sim q$ .

$p$	$q$	$\sim p$	$\sim q$	$\sim p \lor \sim q$
$p \lor \sim q$	T	F	F	F
T	F	F	T	T
F	T	T	F	T
F	F	T	T	T

Example $\PageIndex{3}$

$p \wedge (q\lor \sim q)$

Solution

First, make columns for p and q, then add in $\sim q$ and $q\lor \sim q$ . Finally, evaluate $p\wedge (q\lor \sim q)$ .

$p$	$q$	$\sim q$	$q\lor \sim q$	$p\wedge (q\lor \sim q)$
T	T	F	T	T
T	F	T	T	T
F	T	F	T	F
F	F	T	T	F

Review

Write a truth table for the following variables.

$(p \wedge q)\lor \sim r$
$p \lor ( \sim q \lor r)$
$p \wedge (q \lor \sim r)$
The only difference between #1 and #3 is the placement of the parenthesis. How do the truth tables differ?
When is $p \lor q \lor r$ true?
$p \lor q \lor r$
$(p \lor q) \lor \sim r$
$( \sim p \wedge \sim q) \wedge r$
$( \sim p \lor \sim q) \wedge r$

Is the following a valid argument? If so, what law is being used? HINT: Statements could be out of order.

$p \rightarrow q$

$r \rightarrow p$

$\therefore r \rightarrow q$

$p \rightarrow q$

$r \rightarrow q$

$\therefore p \rightarrow r$

$p \rightarrow \sim r$

$r$

$\therefore \sim p$

$\sim q \rightarrow r$

$q$

$\therefore \sim r$

$p \rightarrow (r \rightarrow s)$

$p$

$\therefore r \rightarrow s$

$r \rightarrow q$

$r \rightarrow s$

$\therefore q \rightarrow s$

Additional Resources

Video: Truth Tables Principles

Practice: Truth Tables

Drawing a Truth Table

Determining the Truths of Variables

Review

Additional Resources

Support Center

How can we help?