Home
Bookshelves
Mathematics
Geometry
3: Lines
3.10: Perpendicular Lines in the Coordinate Plane

3.10: Perpendicular Lines in the Coordinate Plane

Last updated
Save as PDF

Page ID: 4772

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Lines with opposite sign, reciprocal slopes that intersect at right angles.

Here you'll learn that the slopes of perpendicular lines are negative reciprocals of each other. You'll then apply this fact to determine if two lines are perpendicular and to find what their equations are.

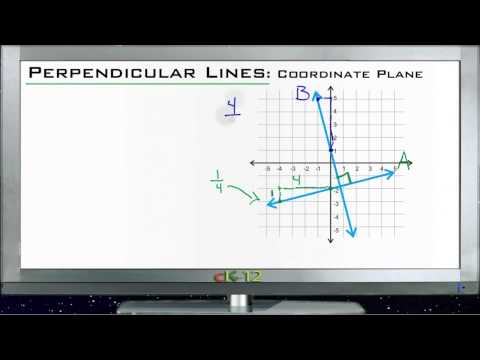
Perpendicular lines are two lines that intersect at a , or right, angle. In the coordinate plane, that would look like this:

f-d_b58b089b8f6b842ed94d2b6810e4f81e02986697681440a6ed7ef6de+IMAGE_TINY+IMAGE_TINY.png — Figure \(\PageIndex{1}\)

If we take a closer look at these two lines, the slope of one is -4 and the other is .

This can be generalized to any pair of perpendicular lines in the coordinate plane. The slopes of perpendicular lines are opposite reciprocals of each other.

What if you were given two perpendicular lines in the coordinate plane? What could you say about their slopes?

Vocabulary

Term	Definition
perpendicular	Two lines that intersect at a \(90^{\circ}\), or right, angle. The slopes of perpendicular lines are opposite reciprocals of each other.

Additional Resource

Interactive Element

Video: Equations of Parallel and Perpendicular Lines

Activities: Perpendicular Lines in the Coordinate Plane Discussion Questions

Study Aids: Lines in the Coordinate Plane

Practice: Perpendicular Lines in the Coordinate Plane

Real World: Perpendicular Lines In The Coordiante Plane