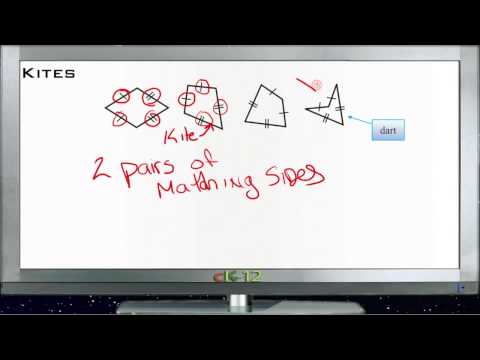
5.16: Kites

Quadrilaterals with two distinct sets of adjacent, congruent sides.

A kite is a quadrilateral with two distinct sets of adjacent congruent sides. It looks like a kite that flies in the air.

Figure $\PageIndex{1}$

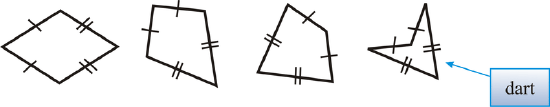
From the definition, a kite could be concave. If a kite is concave, it is called a dart. The word distinct in the definition means that the two pairs of congruent sides have to be different. This means that a square or a rhombus is not a kite.

The angles between the congruent sides are called vertex angles. The other angles are called non-vertex angles. If we draw the diagonal through the vertex angles, we would have two congruent triangles.

Figure $\PageIndex{2}$

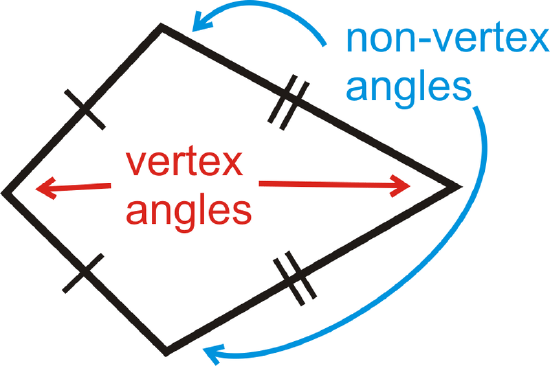
Facts about Kites

1. The non-vertex angles of a kite are congruent.

Figure $\PageIndex{3}$

If $KITE$ is a kite, then $\angle K\cong \angle T$.

2. The diagonal through the vertex angles is the angle bisector for both angles.

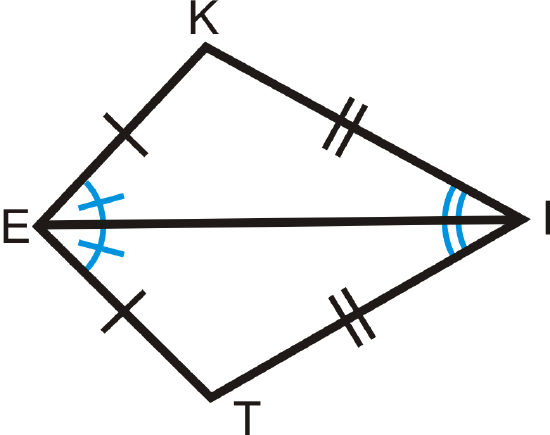Figure \(\PageIndex{4}\)

If $KITE$ is a kite, then $\angle KEI\cong \angle IET$ and $\angle KIE\cong \angle EIT$.

3. Kite Diagonals Theorem: The diagonals of a kite are perpendicular.

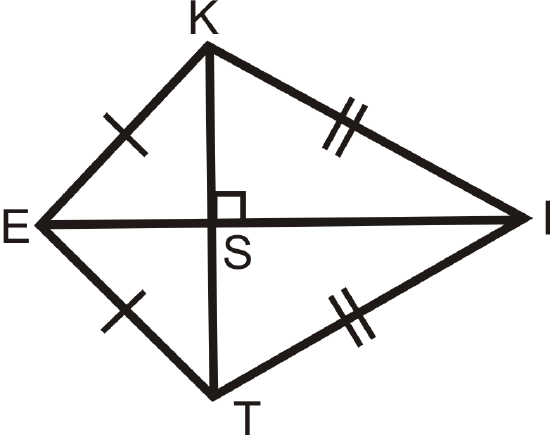Figure $\PageIndex{5}$

$\Delta KET$ and $\Delta KIT$ are isosceles triangles, so $\overline{EI}$ is the perpendicular bisector of $\overline{KT}$ (Isosceles Triangle Theorem).

What if you were told that $WIND$ is a kite and you are given information about some of its angles or its diagonals? How would you find the measure of its other angles or its sides?

For Examples 1 and 2, use the following information:

$KITE$ is a kite.

Figure $\PageIndex{6}$

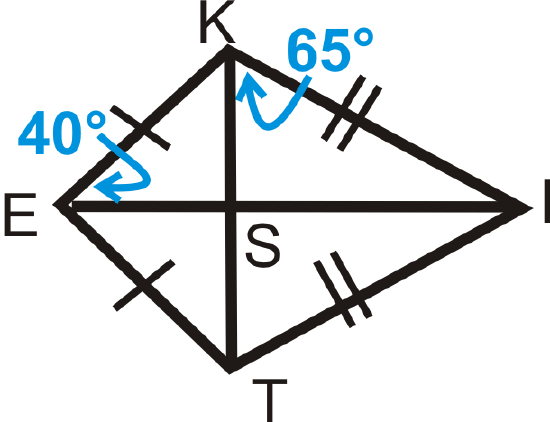
Example $\PageIndex{1}$

Find $m\angle KIS$.

Solution

$m\angle KIS=25^{\circ}$ by the Triangle Sum Theorem (remember that \angle KSI is a right angle because the diagonals are perpendicular.)

Example $\PageIndex{2}$

Find $m\angle IST$.

Solution

$m\angle IST=90^{\circ}$ because the diagonals are perpendicular.

Example $\PageIndex{3}$

Find the missing measures in the kites below.

1. 

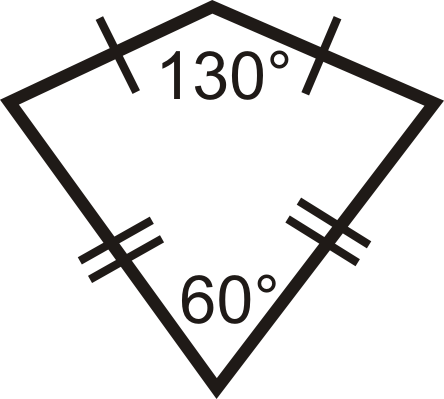
   Figure $\PageIndex{7}$

2. 

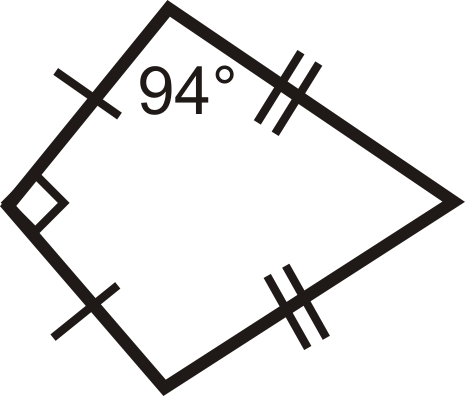
   Figure $\PageIndex{8}$

Solution

1. The two angles left are the non-vertex angles, which are congruent.

   $\begin{aligned} 130^{\circ} +60^{\circ} +x+x=360^{\circ} \\ 2x&=170^{\circ} \\ x&=85^{\circ} \qquad Both angles are 85^{\circ} \end{aligned}$

2. The other non-vertex angle is also $94^{\circ}$. To find the fourth angle, subtract the other three angles from $360^{\circ}$.

   $\begin{aligned} 90^{\circ} +94^{\circ} +94^{\circ} +x &=360^{\circ} \\ x&=82^{\circ} \end{aligned}$

Example $\PageIndex{4}$

Use the Pythagorean Theorem to find the lengths of the sides of the kite.

Figure $\PageIndex{9}$

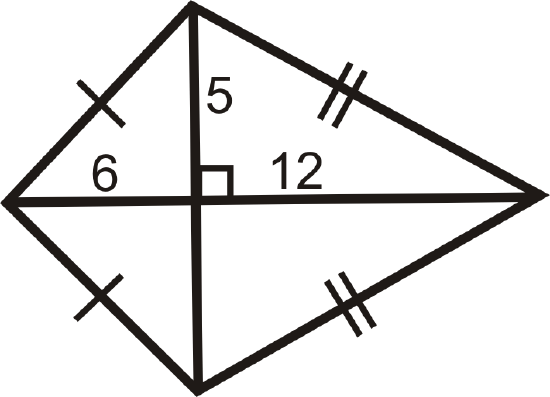
Solution

Recall that the Pythagorean Theorem says $a^2+b^2=c^2$, where $c$ is the hypotenuse. In this kite, the sides are the hypotenuses.

$\begin{array}{rr} 6^{2}+5^{2}=h^{2} & 12^{2}+5^{2}=j^{2} \\ 36+25=h^{2} & 144+25=j^{2} \\ 61=h^{2} & 169=j^{2} \\ \sqrt{61}=h & 13=j \end{array}$

Example $\PageIndex{5}$

Prove that the non-vertex angles of a kite are congruent.

Given: $KITE$ with $\overline{KE}\cong \overline{TE}$ and $\overline{KI}\cong \overline{TI}$

Prove: $\angle K\cong \angle T$

Figure $\PageIndex{10}$

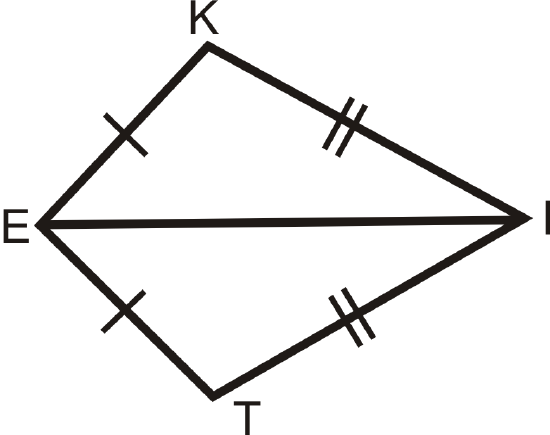
Solution

Statement	Reason
1. $\overline{KE}\cong \overline{TE}$ and $\overline{KI}\cong \overline{TI}$	1. Given
2.$\overline{EI}\cong \overline{EI}$	2. Reflexive PoC
3. $\Delta EKI\cong \Delta ETI$	3. SSS
4. $\angle K\cong \angle T$	4. CPCTC

Review

For questions 1-6, find the value of the missing variable(s). All figures are kites.

1. 

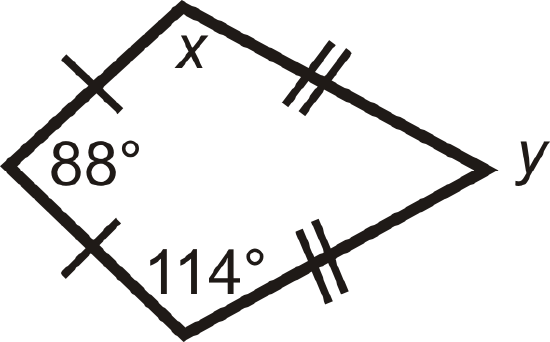
   Figure $\PageIndex{11}$

2. 

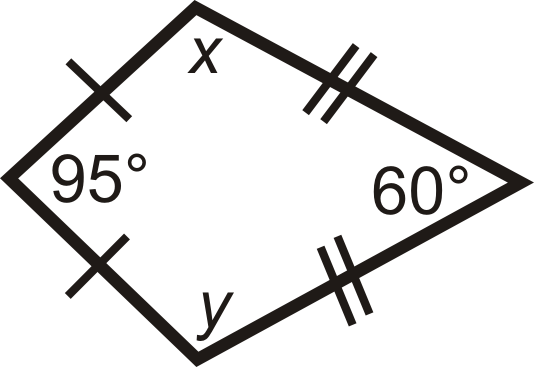
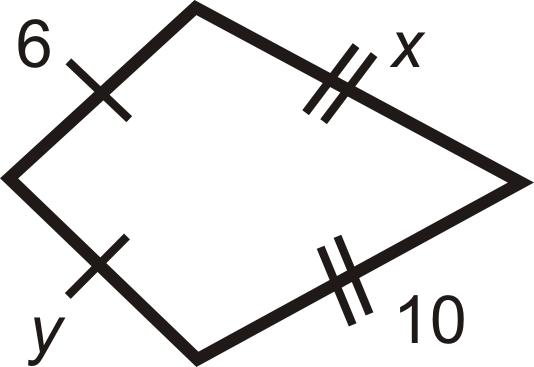
   Figure $\PageIndex{12}$

3. 

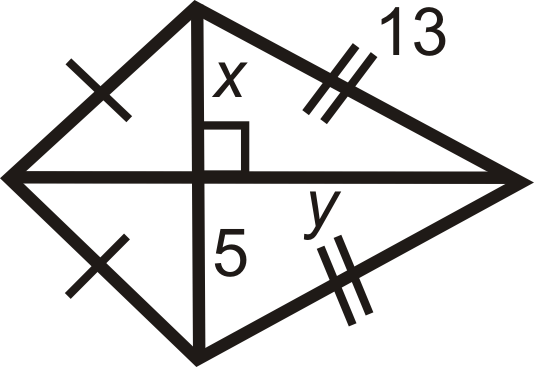
   Figure $\PageIndex{13}$

4. 

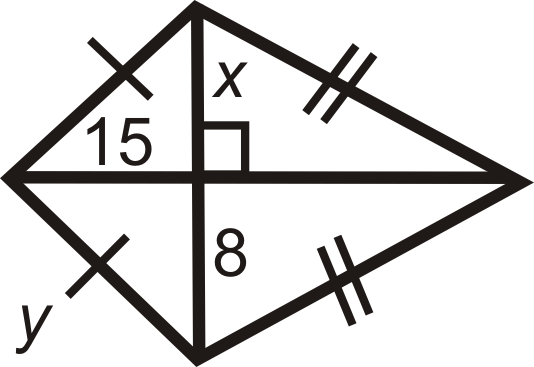   Figure $\PageIndex{14}$

5. Figure $\PageIndex{15}$

6. 

   Figure $\PageIndex{16}$

For questions 7-11, find the value of the missing variable(s).

7. 

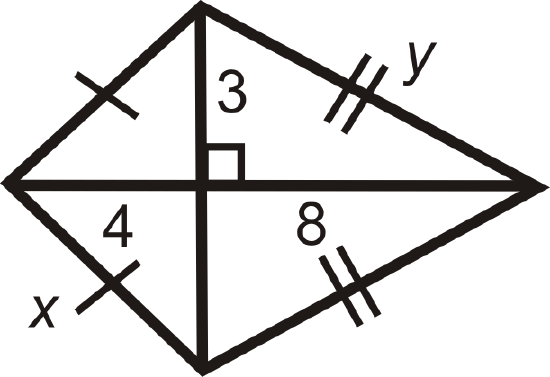
   Figure $\PageIndex{17}$

8. 

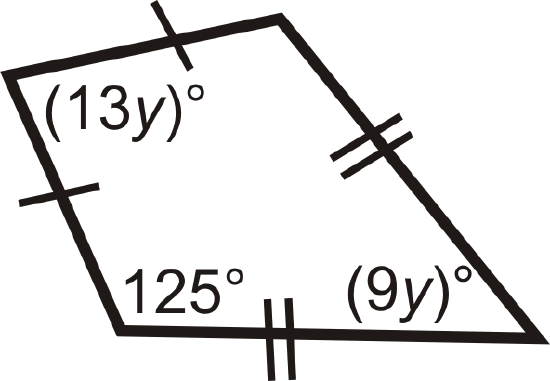
   Figure $\PageIndex{18}$

9. 

   Figure $\PageIndex{19}$

10. 

    Figure $\PageIndex{20}$

11. 

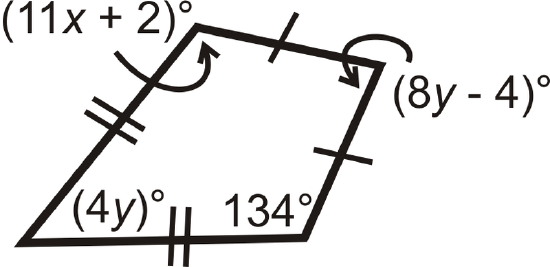
    Figure $\PageIndex{21}$

12. Fill in the blanks to the proof below.

Given: $\overline{KE}\cong \overline{TE}$ and $\overline{KI}\cong \overline{TI}$

Prove: $\overline{EI}$ is the angle bisector of $\angle KET$ and $\angle KIT$

Figure $\PageIndex{22}$

Statement	Reason
1.$\overline{KE}\cong \overline{TE} and \overline{KI}\cong \overline{TI}$	1.
2. $\overline{EI}\cong \overline{EI}$	2.
3. $\Delta EKI\cong \Delta ETI$	3.
4.	4. CPCTC
5. $\overline{EI} is the angle bisector of \angle KET$ and \angle KIT\)	5.

13. Fill in the blanks to the proof below.

Given: $\overline{EK}\cong \overline{ET},\: \overline{KI}\cong \overline{IT}$

Prove: $\overline{KT}\perp \overline{EI}$

Figure $\PageIndex{23}$

Statement	Reason
1. $\overline{KE}\cong \overline{TE}$ and $\overline{KI}\cong \overline{TI}$	1.
2.	2. Definition of isosceles triangles
3. $\overline{EI}$ is the angle bisector of $\angle KET$ and $\angle KIT$	3.
4.	4. Isosceles Triangle Theorem
5. $\overline{KT}\perp \overline{EI}$	5.

Review (Answers)

To see the Review answers, open this PDF file and look for section 6.7.

Vocabulary

Term	Definition
kite	A quadrilateral with distinct adjacent congruent sides.
Triangle Sum Theorem	The Triangle Sum Theorem states that the three interior angles of any triangle add up to 180 degrees.
Vertical Angles	Vertical angles are a pair of opposite angles created by intersecting lines.

Additional Resources

Interactive Element

Video: Kites Principles - Basic

Activities: Kites Discussion Questions

Study Aids: Trapezoids and Kites Study Guide

Practice: Kites

Real World: Go Fly a Kite