6.15: Inscribed Quadrilaterals in Circles
( \newcommand{\kernel}{\mathrm{null}\,}\)
Quadrilaterals with every vertex on a circle and opposite angles that are supplementary.
An inscribed polygon is a polygon where every vertex is on the circle, as shown below.
For inscribed quadrilaterals in particular, the opposite angles will always be supplementary.
Inscribed Quadrilateral Theorem: A quadrilateral can be inscribed in a circle if and only if the opposite angles are supplementary.
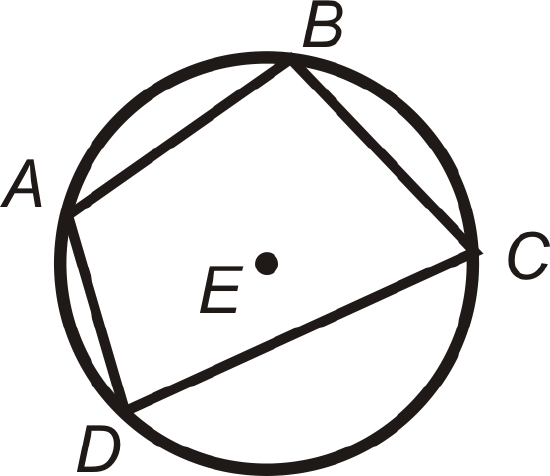
If ABCD is inscribed in ⨀E, then m∠A+m∠C=180∘ and m∠B+m∠D=180∘. Conversely, If m∠A+m∠C=180∘ and m∠B+m∠D=180∘, then ABCD is inscribed in ⨀E.
What if you were given a circle with a quadrilateral inscribed in it? How could you use information about the arcs formed by the quadrilateral and/or the quadrilateral's angle measures to find the measure of the unknown quadrilateral angles?
Example 6.15.1
-
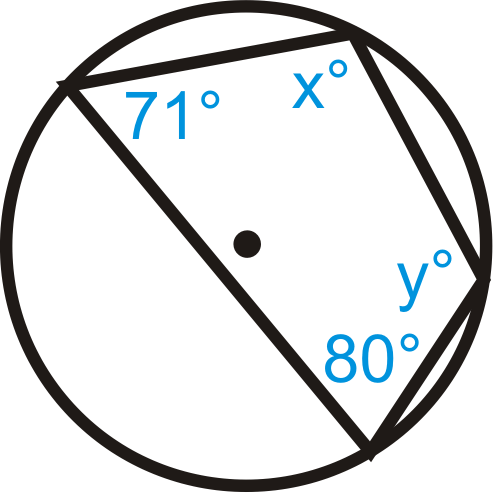
Figure 6.15.3 -
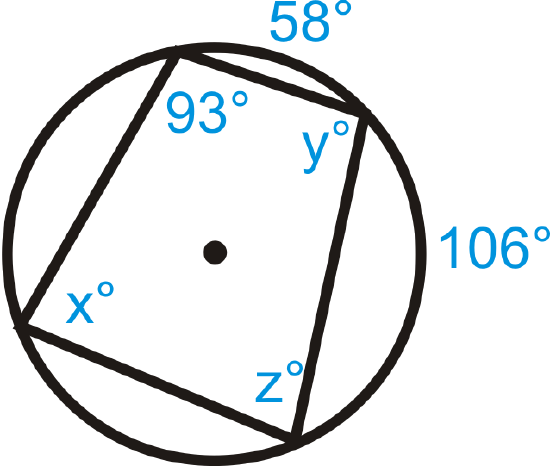
Figure 6.15.4
Solution
- x+80∘=180∘y+71∘=180∘x=100∘y=109∘
- z+93∘=180∘x=12(58∘+106∘)y+82∘=180∘z=87∘x=82∘y=98∘
Example 6.15.2
Find x and y in the picture below.
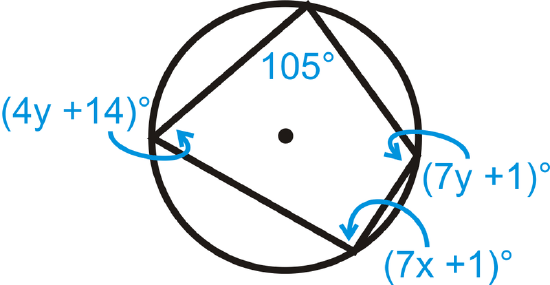
Solution
(7x+1)∘+105∘=180∘(4y+14)∘+(7y+1)∘=180∘7x+106∘=180∘11y+15∘=180∘7x=7411y=165x=10.57y=15
Example 6.15.3
Find the values of x and y in ⨀A.
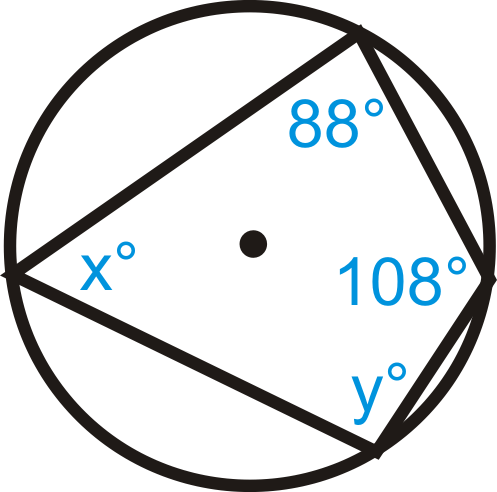
Solution
Use the Inscribed Quadrilateral Theorem. x∘+108∘=180∘ so x=72∘. Similarly, y∘+88∘=180∘ so y=92∘.
Example 6.15.4
Quadrilateral ABCD is inscribed in ⨀E. Find m∠A, m∠B, m∠C, and m∠D.
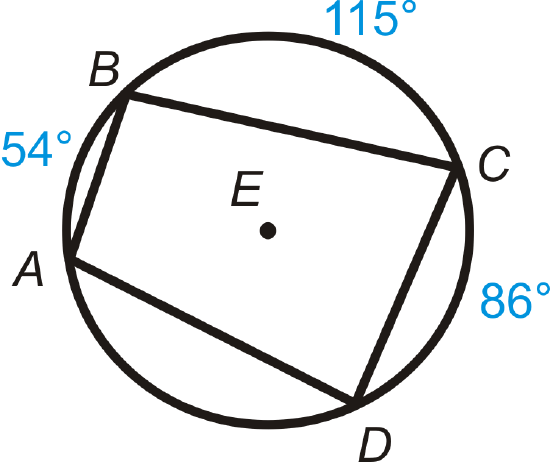
Solution
First, note that m^AD=105∘ because the complete circle must add up to 360∘.
m∠A=12m^BD=12(115+86)=100.5∘m∠B=12m^AC=12(86+105)=95.5∘m∠C=180∘−m∠A=180∘−100.5∘=79.5∘m∠D=180∘−m∠B=180∘−95.5∘=84.5∘
Review
Fill in the blanks.
- A(n) _______________ polygon has all its vertices on a circle.
- The _____________ angles of an inscribed quadrilateral are ________________.
Quadrilateral ABCD is inscribed in ⨀E. Find:
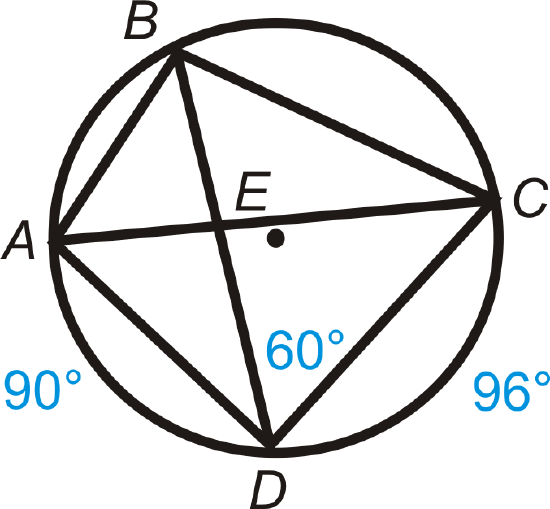
- m∠DBC
- m^BC
- m^AB
- m∠ACD
- m∠ADC
- m∠ACB
Find the value of x and/or y in ⨀A.
-
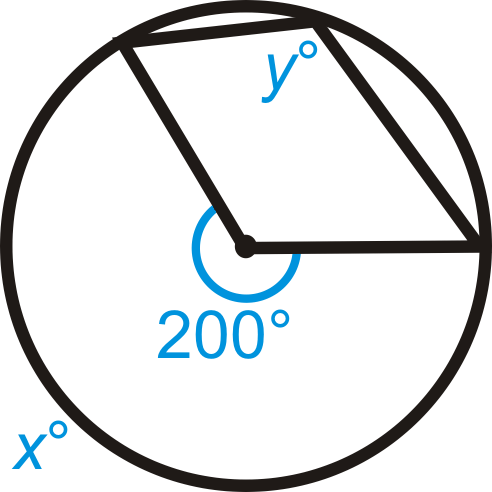
Figure 6.15.9 -
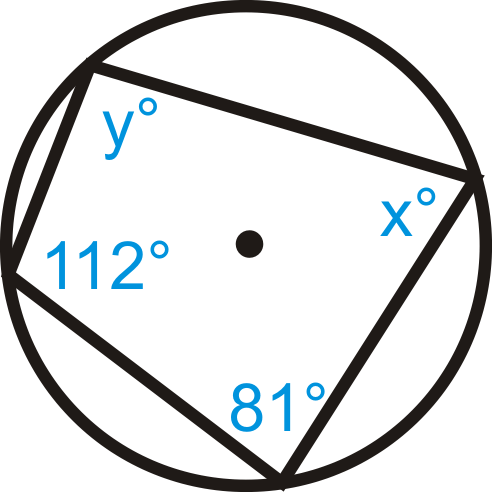
Figure 6.15.10 -
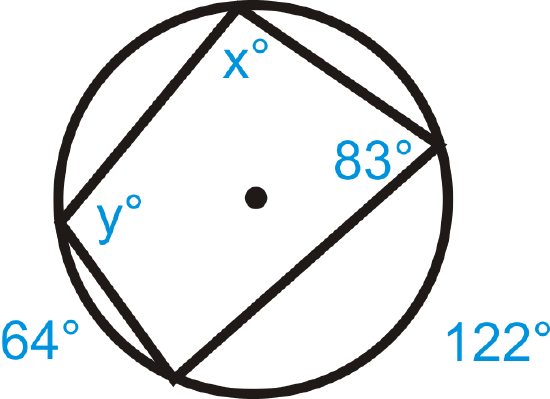
Figure 6.15.11
Solve for x.
-
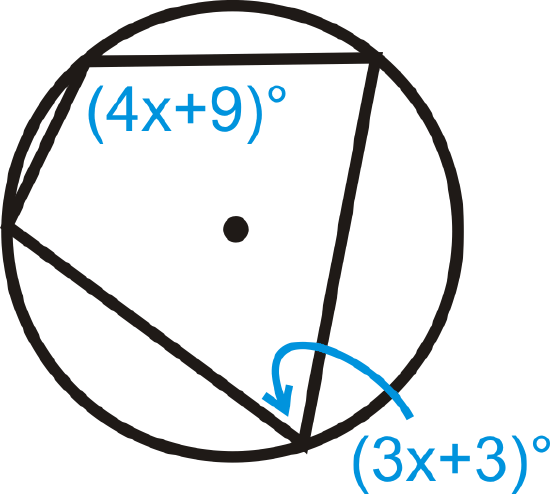
Figure 6.15.12 -

Figure 6.15.13
Vocabulary
| Term | Definition |
|---|---|
| central angle | An angle formed by two radii and whose vertex is at the center of the circle. |
| chord | A line segment whose endpoints are on a circle. |
| circle | The set of all points that are the same distance away from a specific point, called the center. |
| diameter | A chord that passes through the center of the circle. The length of a diameter is two times the length of a radius. |
| inscribed angle | An angle with its vertex on the circle and whose sides are chords. |
| intercepted arc | The arc that is inside an inscribed angle and whose endpoints are on the angle. |
| radius | The distance from the center to the outer rim of a circle. |
| Inscribed Polygon | An inscribed polygon is a polygon with every vertex on a given circle. |
| Inscribed Quadrilateral Theorem | The Inscribed Quadrilateral Theorem states that a quadrilateral can be inscribed in a circle if and only if the opposite angles of the quadrilateral are supplementary. |
| Cyclic Quadrilaterals | A cyclic quadrilateral is a quadrilateral that can be inscribed in a circle. |
Additional Resources
Interactive Element
Video: Inscribed Quadrilaterals in Circles Principles - Basic
Activities: Inscribed Quadrilaterals in Circles Discussion Questions
Study Aids: Inscribed in Circles Study Guide
Practice: Inscribed Quadrilaterals in Circles
Real World: Sunrise at Stonehenge


