Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

2.16: Parallelogram Proofs

Last updated

Nov 28, 2020
Save as PDF
- 2.15: Proofs- Angle Pairs and Segments
- 2.17: Proofs Involving Triangles

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Apply theorems to show if a quadrilateral has two pairs of parallel sides.

Quadrilaterals that are Parallelograms

Recall that a parallelogram is a quadrilateral with two pairs of parallel sides. Even if a quadrilateral is not marked with having two pairs of sides, it still might be a parallelogram. The following is a list of theorems that will help you decide if a quadrilateral is a parallelogram or not.

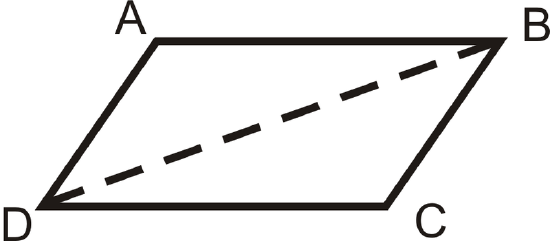
1. Opposite Sides Theorem Converse: If both pairs of opposite sides of a quadrilateral are congruent, then the figure is a parallelogram.

f-d_b44f3f753ae5d2c0f64fe05ea27747fece73e8409e7a42a40ebe4a1b+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{1}$

then

f-d_994d6497cbae0e45c57daa4d03e6067e99015df4ccb97c8bf5a14596+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{2}$

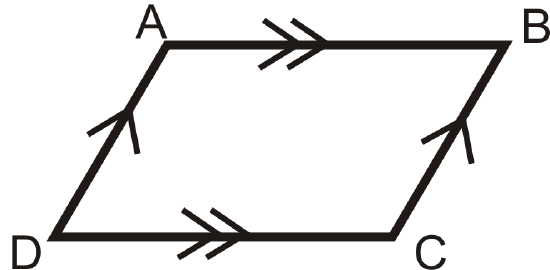
2. Opposite Angles Theorem Converse: If both pairs of opposite angles of a quadrilateral are congruent, then the figure is a parallelogram.

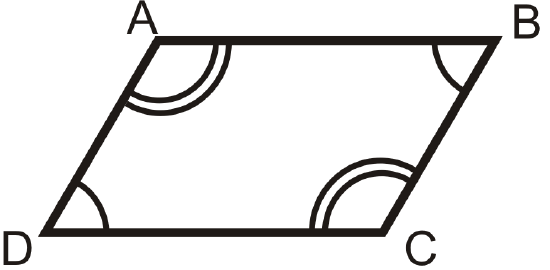
f-d_2118168440f8fc1a13c1238ef26d6fa54c1173fb4e7e4e2a20c7199c+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{3}$

then

f-d_528830801800a01007e868c589b2ebf40ab26919f4557be5e605481a+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{4}$

3. Parallelogram Diagonals Theorem Converse: If the diagonals of a quadrilateral bisect each other, then the figure is a parallelogram.

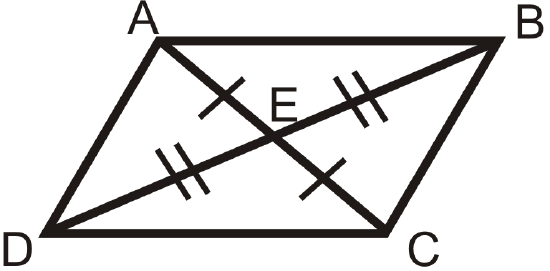
f-d_46c004c44a75296d2eac72bd737291bae01ab65e1820b5204f3586ca+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{5}$

then

f-d_c6443e1c10cf2e9c4d75ba5b555b815d0a95690ca23ea976a0b40e5f+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{6}$

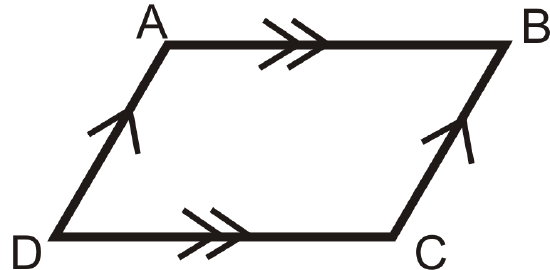
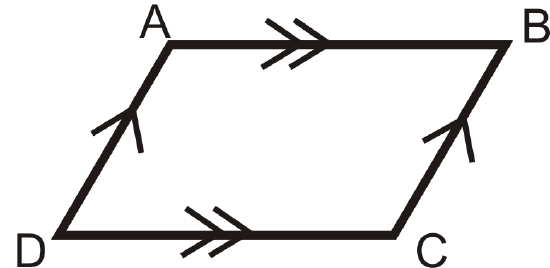
4. Parallel Congruent Sides Theorem: If a quadrilateral has one set of parallel lines that are also congruent, then it is a parallelogram.

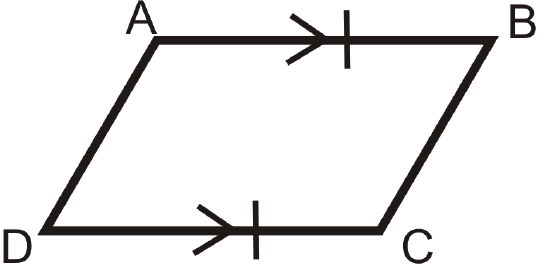
f-d_e46203f33a4881541307b95407d5031345edcb82ae45185ea0c3aa76+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{7}$

then

f-d_7d8fcf2fea2284750352c44fdde20db6255d6298f2cf47be907ff997+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{8}$

You can use any of the above theorems to help show that a quadrilateral is a parallelogram. If you are working in the x−y plane, you might need to know the formulas shown below to help you use the theorems.

The Slope Formula, $\dfrac{y_2−y_1}{x_2−x_1}$ . (Remember that if slopes are the same then lines are parallel).
The Distance Formula, $\sqrt{(x_2−x_1)^2+(y_2−y_1)^2}$ . (This will help you to show that two sides are congruent).
The Midpoint Formula, $( \dfrac{x_1+x_2}{2}, \dfrac{y_1+y_2}{2} )$ . (If the midpoints of the diagonals are the same then the diagonals bisect each other).

What if you were given four pairs of coordinates that form a quadrilateral? How could you determine if that quadrilateral is a parallelogram?

Example $\PageIndex{1}$

Prove the Parallel Congruent Sides Theorem.

f-d_481247021bd2921618edf2f5e05543ff7668412a6a797825b7b69f29+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{9}$

Given: $\overline{AB}\parallel\overline{DC}$ , and $\overline{AB}\cong \overline{DC}$

Prove: $ABCD$ is a parallelogram

Solution

*Statement*	*Reason*
1. $\overline{AB}\parallel\overline{DC}$ , and $\overline{AB}\cong \overline{DC}$	1. Given
2. $\angle ABD\cong \angle BDC$	2. Alternate Interior Angles
3. $\overline{DB}\cong \overline{DB}$	3. Reflexive $PoC$
4. $\Delta ABD\cong \Delta CDB$	4. SAS
5. $\overline{AD}\cong \overline{BC}$ \)	5. CPCTC
6. $ABCD$ is a parallelogram	6. Opposite Sides Converse

Example $\PageIndex{2}$

What value of $x$ would make $ABCD$ a parallelogram?

f-d_57be9d320081b376883ec8cad8136dedf5e1644f34d20438c1632327+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{10}$

Solution

$\overline{AB}\parallel\overline{DC}$ . By the Parallel Congruent Sides Theorem, $ABCD$ would be a parallelogram if $AB=DC$ .

$\begin{align*} 5x−8 &=2x+13 \\ 3x &=21 \\ x &=7 \end{align*}$

Example $\PageIndex{3}$

Prove the Opposite Sides Theorem Converse.

Given: $\overline{AB}\cong \overline{DC}$ , $\overline{AD}\cong \overline{BC}$

Prove: $ABCD$ is a parallelogram

Solution

*Statement*	*Reason*
1. $\overline{AB}\cong \overline{DC}$ , $\overline{AD}\cong \overline{BC}$	1.Given
2. $\overline{DB}\cong \overline{DB}$	2. Reflexive $PoC$
3. $\Delta ABD\cong \Delta CDB$	3. SSS
4. $\angle ABD\cong \angle BDC$ , $\angle ADB\cong \angle DBC$	4. $CPCTC$
5. $\overline{AB}\parallel\overline{DC}$ , $\overline{AD}\parallel\overline{BC}$	5. Alternate Interior Angles Converse
6. $ABCD$ is a parallelogram	6. Definition of a parallelogram

Example $\PageIndex{4}$

Is quadrilateral $EFGH$ a parallelogram? How do you know?

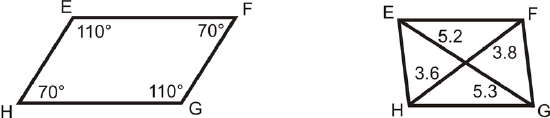
f-d_b1740b63357c5d41fd536f61ac4336ce081028133139b46445b5a7af+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{12}$

Solution

By the Opposite Angles Theorem Converse, $EFGH$ is a parallelogram.

$EFGH$ is not a parallelogram because the diagonals do not bisect each other.

Example $\PageIndex{5}$

Is the quadrilateral $ABCD$ a parallelogram?

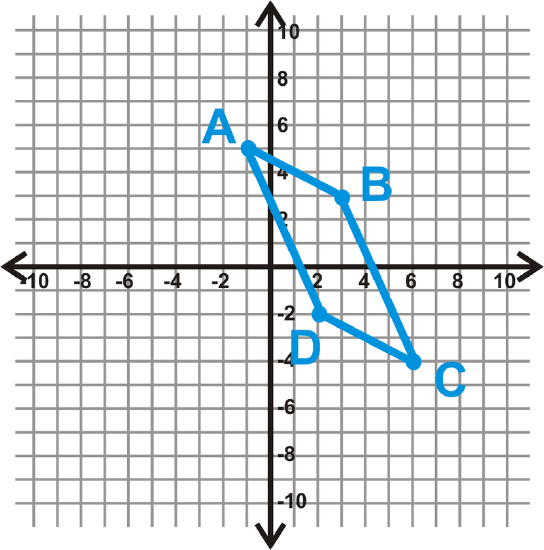
f-d_ad6c14bc1b86d3eeeaa76acbda23277a9070ebd2c788dea374e53bbc+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{13}$

Solution

Let’s use the Parallel Congruent Sides Theorem to see if $ABCD$ is a parallelogram. First, find the length of AB and CD using the distance formula.

$\begin{align*} AB &=\sqrt{(−1−3)^2+(5−3)^2} & CD &=\sqrt{(2−6)^2+(−2+4)^2} \\ &=\sqrt{(−4)^{2}+2^2} & &=\sqrt{(−4)^2+2^2} \\ &=\sqrt{16+4}=\sqrt{20} & & =\sqrt{16+4}=\sqrt{20} \end{align*}$

Next find the slopes to check if the lines are parallel.

$\begin{align*} Slope \: AB =\dfrac{5−3}{−1−3} =\dfrac{2}{−4} &=−\dfrac{1}{2} &Slope \: CD=\dfrac{−2+4}{2−6}=\dfrac{2}{−4}=−\dfrac{1}{2} \end{align*}$

$AB=CD$ and the slopes are the same (implying that the lines are parallel), so $ABCD$ is a parallelogram.

Review

For questions 1-12, determine if the quadrilaterals are parallelograms.

Figure $\PageIndex{14}$
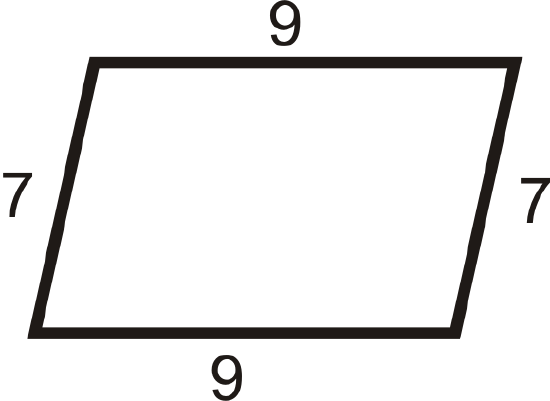
Figure $\PageIndex{15}$
Figure $\PageIndex{16}$
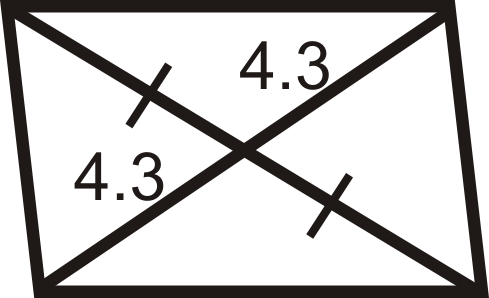
Figure $\PageIndex{17}$
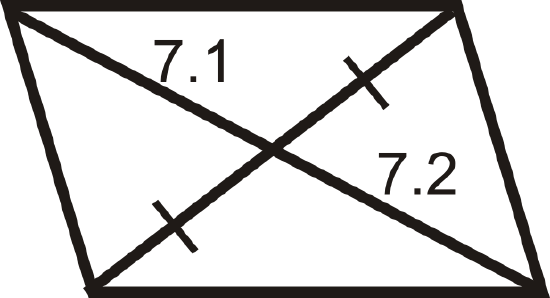
Figure $\PageIndex{18}$
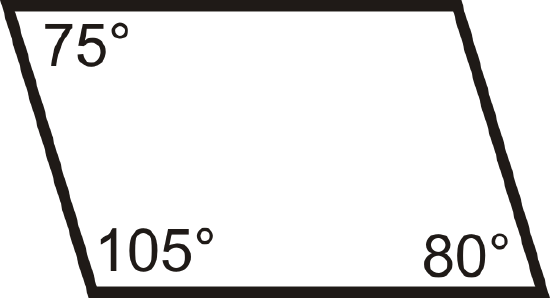
Figure $\PageIndex{19}$
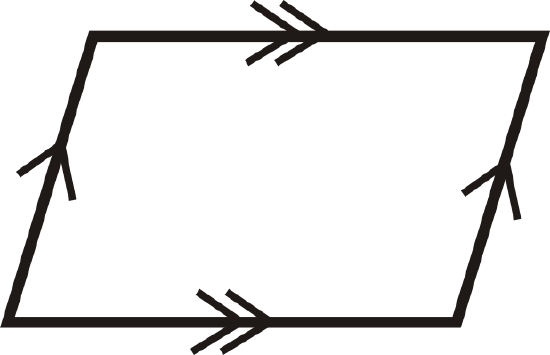
Figure $\PageIndex{20}$
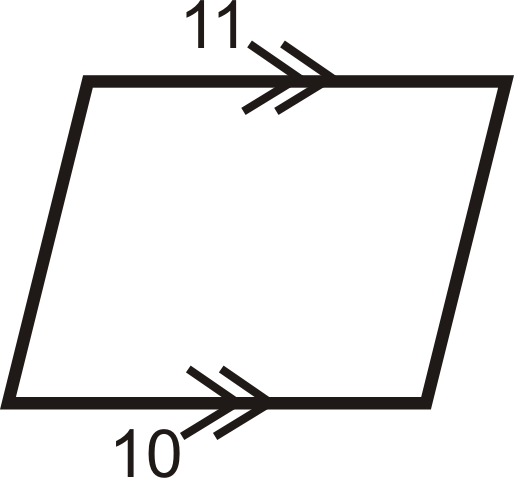
Figure $\PageIndex{21}$
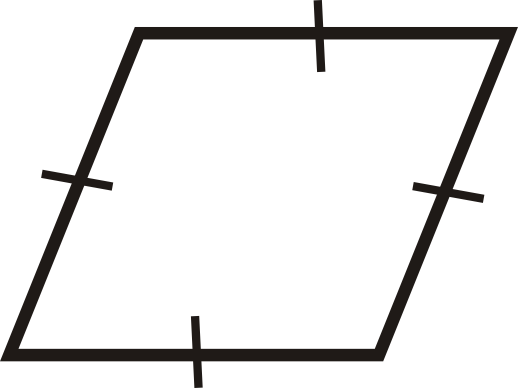
Figure $\PageIndex{22}$
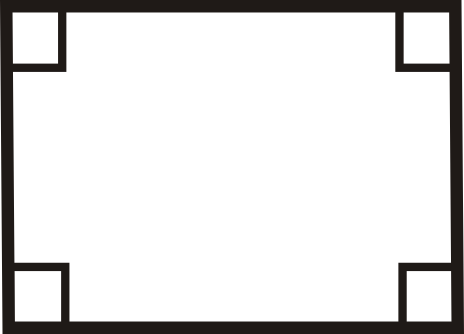
Figure $\PageIndex{23}$
Figure $\PageIndex{24}$
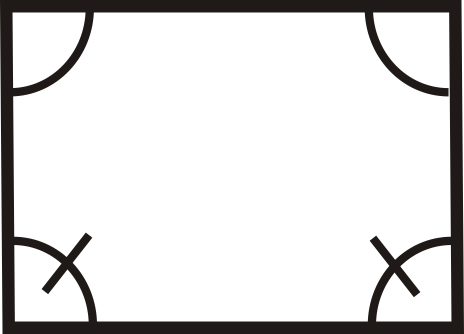
Figure $\PageIndex{25}$

For questions 13-18, determine the value of $x$ and $y$ that would make the quadrilateral a parallelogram.

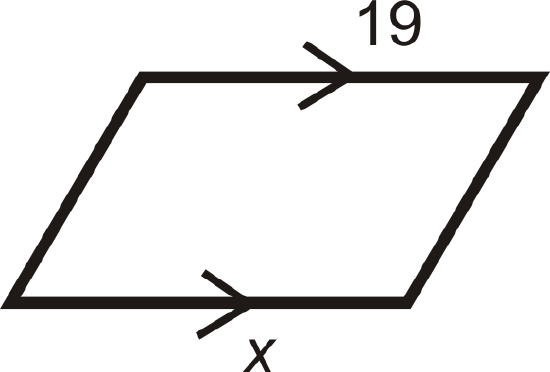
Figure $\PageIndex{26}$
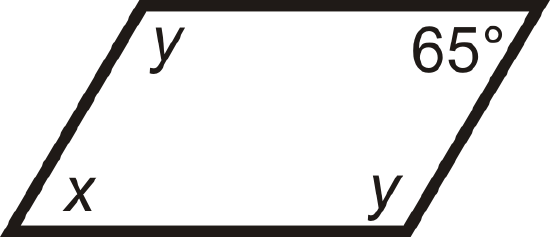
Figure $\PageIndex{27}$
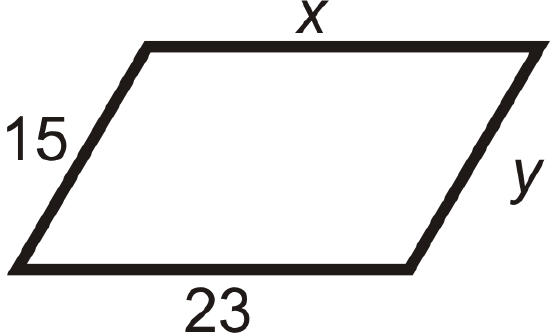
Figure $\PageIndex{28}$
Figure $\PageIndex{29}$
Figure $\PageIndex{30}$
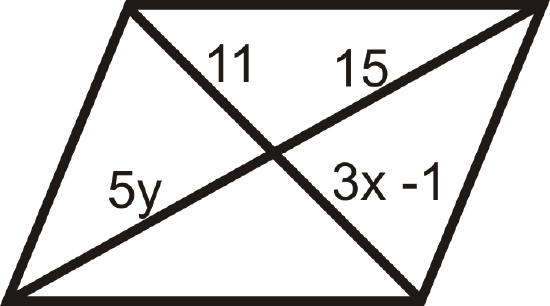
Figure $\PageIndex{31}$

For questions 19-22, determine if $ABCD$ is a parallelogram.

$A(8,−1)$ , $B(6,5)$ , $C(−7,2)$ , $D(−5,−4)$
$A(−5,8)$ , $B(−2,9)$ , $C(3,4)$ , $D(0,3)$
$A(−2,6)$ , $B(4,−4)$ , $C(13,−7)$ , $D(4,−10)$
$A(−9,−1)$ , $B(−7,5)$ , $C(3,8)$ , $D(1,2)$

Fill in the blanks in the proofs below.

Opposite Angles Theorem Converse

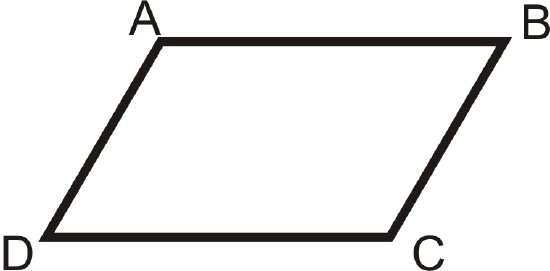
f-d_14c5c7af431574174f61e564b4ce1c17a9f6dafef030e565b85454c6+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{32}$

Given: $\angle A\cong \angle C$ , $\angle D\cong \angle B$

Prove: $ABCD$ is a parallelogram

*Statement*	*Reason*
1.	1.
2. $m\angle A=m\angle C$ , $m\angle D=m\angle B$	2.
3.	3. Definition of a quadrilateral
4. $m\angle A+m\angle A+m\angle B+m\angle B=360^{\circ}$	4.
5.	5. Combine Like Terms
6.	6. Division $PoE$
7. $\angle A$ and $\angle B$ are supplementary $\angle A$ and $\angle D$ are supplementary	7.
8.	8. Consecutive Interior Angles Converse
9. $ABCD$ is a parallelogram	9.

Parallelogram Diagonals Theorem Converse

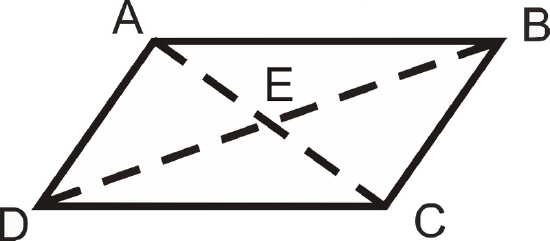
f-d_4c50cdd807989540195ffb2226df7dc09513ff33267308d9f8203ca8+IMAGE_TINY+IMAGE_TINY.png — Figure $\PageIndex{33}$

Given: $\overline{AE}\cong \overline{EC}$ , $\overline{DE}\cong \overline{EB}$

Prove: $ABCD$ is a parallelogram

*Statement*	*Reason*
1.	1.
2.	2. Vertical Angles Theorem
3. $\Delta AED \cong \Delta CEB$ $\Delta AEB\cong \Delta CED$	3.
4.	4.
5. $ABCD$ is a parallelogram	5.

Given: $\angle ADB\cong \angle CBD$ , $\overline{AD}\cong \overline{BC}$

Prove: $ABCD$ is a parallelogram

*Statement*	*Reason*
1.	1.
2. $\overline{AD}\parallel\overline{BC}$	2.
3. $ABCD$ is a parallelogram	3.

Review (Answers)

To see the Review answers, open this PDF file and look for section 6.4.

Additional Resources

Interactive Element

Video: Proving a Quadrilateral is a Parallelogram Principles - Basic

Activities: Quadrilaterals that are Parallelograms Discussion Questions

Study Aids: Parallelograms Study Guide

Practice: Parallelogram Proofs

Real World: Quadrilaterals That Are Parallelograms

Quadrilaterals that are Parallelograms

Review

Review (Answers)

Additional Resources

Support Center

How can we help?