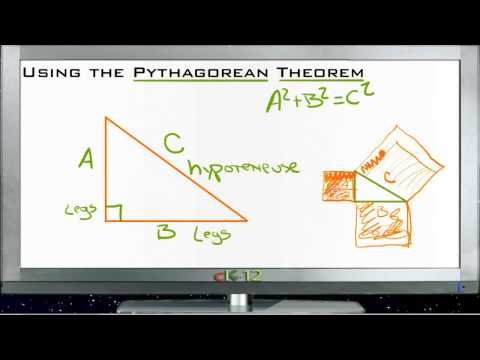
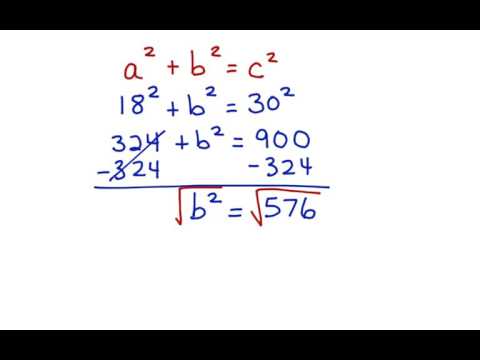4.33: Pythagorean Theorem and its Converse
( \newcommand{\kernel}{\mathrm{null}\,}\)
Use a-squared + b-squared = c-squared to prove triangles are right triangles.
Suppose you're pitching a tent, and an 8-foot pole makes a right angle with the ground. If a rope goes from the top of the pole to a point on the ground 12 feet away from the base of the pole, how long is the rope? How would you calculate its length?
Pythagorean Theorem and its Converse
The Pythagorean Theorem
One of the most important theorems in mathematics and science is Pythagorean’s Theorem. Simply put, it states, “The sum of the square of each leg of a right triangle is equal to the square of the hypotenuse.”

A right triangle is a triangle with a right angle. Let’s review basic right triangle anatomy.
The two segments forming the right angle (90∘) are called the legs of the right triangle. The segment opposite the right angle is called the hypotenuse.
The Pythagorean Theorem states, (leg1)2+(leg2)2=(hypotenuse)2:
a2+b2=c2
Or, to find the hypotenuse, c=√a2+b2.
Notice this relationship is only true for right triangles. In later courses, you will learn how to determine relationships with non-right triangles.
Although we usually refer to the Pythagorean Theorem when determining side lengths of a right triangle, the theorem originally made a statement about areas. If we build squares on each side of a right triangle, the Pythagorean Theorem says that the area of the square whose side is the hypotenuse is equal to the sum of the areas of the squares formed by the legs of the triangle.
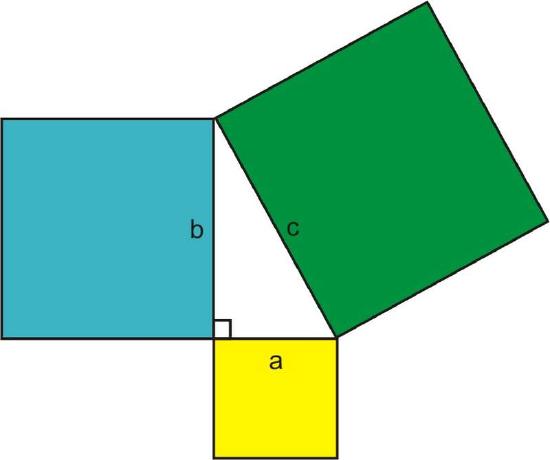
The Converse of the Pythagorean Theorem
The Converse of the Pythagorean Theorem is also true. That is, if the lengths of three sides of a triangle make the equation a2+b2=c2 true, then they represent the sides of a right triangle.
With this converse, you can use the Pythagorean Theorem to prove that a triangle is a right triangle, even if you do not know any of the triangle’s angle measurements.
Let's complete the following problems involving right triangles and the Pythagorean Theorem:
- Does the triangle below contain a right angle?
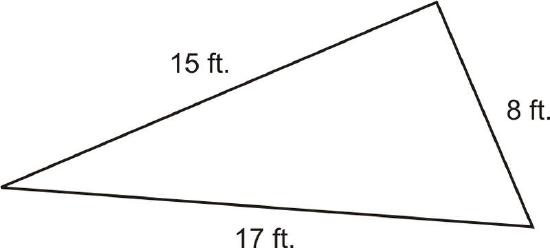
This triangle does not have any right angle marks or measured angles, so you cannot assume you know whether the triangle is acute, right, or obtuse just by looking at it. Take a moment to analyze the side lengths and see how they are related. Two of the sides, 15 and 17, are relatively close in length. The third side, 8, is about half the length of the two longer sides.
To see if the triangle might be right, try substituting the side lengths into the Pythagorean Theorem to see if they make the equation true. The hypotenuse is always the longest side, so 17 should be substituted for c. The other two values can represent a and b and the order is not important.
a2+b2=c282+152=17264+225=289289=289
Since both sides of the equation are equal, these values satisfy the Pythagorean Theorem. Therefore, the triangle described in the problem is a right triangle.
- One leg of a right triangle is 5 more than the other leg. The hypotenuse is one more than twice the size of the short leg. Find the dimensions of the triangle.
Let x= length of the short leg. Then, x+5=lengthofthelongleg and 2x+1=lengthofthehypotenuse.
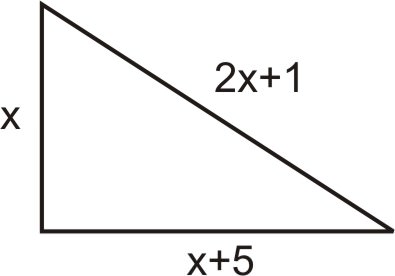
The sides of the triangle must satisfy the Pythagorean Theorem.
x2+(x+5)2=(2x+1)Eliminatetheparentheses.x2+x2+10x+25=4x2+4x+1Movealltermstotherighthandsideoftheequation.0=2x2−6x−24Dividealltermsby2.0=x2−3x−12Solveusingthequadraticformula.x=3±√9+482=3±√572x≠5.27orx≠−2.27
The negative solution does not make sense in the context of this problem. So, use x=5.27 and we get
short−leg=5.27,long−leg=10.27 and
hypotenuse=11.54.
- Find the area of the shaded region in the following diagram.
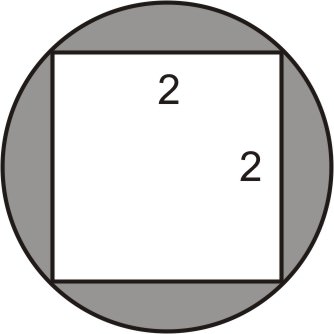
Draw the diagonal of the square on the figure.
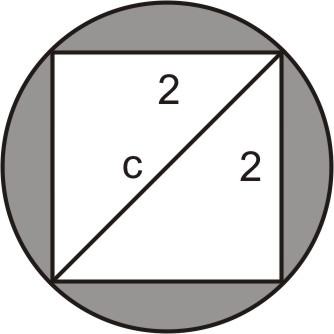
Notice that the diagonal of the square is also the diameter of the circle.
Define variables. Let c=diameterofthecircle.
22+22=c24+4=c2Writetheformulaandsolve.c2=8⇒c=√8⇒c=2√2
The diameter of the circle is 2√2. Therefore, the radius is r=√2.
The area of a circle is A=πr2=π(√2)2=2π.
The area of the shaded region is, therefore, 2\pi −4\neq 2.28\).
Example 4.33.1
Earlier, you were asked to find the length of a rope you used to pitch a tent. You know that the rope goes from the top of an 8 foot pole to a point on the ground 12 feet away from the base.
Solution
This situation can be represented by a right triangle.
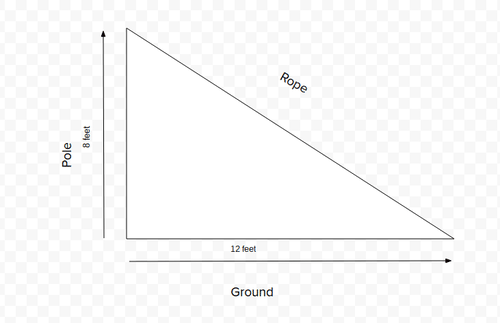
Assuming that the angle between the ground and the pole is 90 degrees, we can use the Pythagorean Theorem to solve for the length of the rope.
Let a=8feet(lengthofthepole),\(b=12feet (the distance from the pole to the rope on the ground), and c=thelengthoftherope.
a2+b2=c282+122=c2208=c2c=√208c=√16√13c=4√13feet
The rope is 4√13 feet long.
Example 4.33.2
Determine whether or not a triangle with sides of lengths 5, 6 and 8 forms a right triangle.
Solution
Use the Converse of the Pythagorean Theorem:
StartwiththePythagoreanequation.a2+b2=c2Substituteinthevaluesofthesides.52+62?=82Simplify.25+36?=64Check.61≠64
Since these lengths of sides do not satisfy the equation of the Pythagorean Theorem, the triangle is not a right triangle.
Review
Verify that each triangle is a right triangle.
- a=12,b=9,c=15
- a=6,b=6,c=6√2
- a=8,b=8√3,c=16
Find the missing length of each right triangle.
- a=12,b=16,c=?
- a=?,b=20,c=30
- a=4,b=?,c=11
-

Figure 4.33.8 -
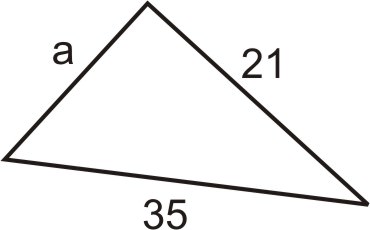
Figure 4.33.9 -

Figure 4.33.10 - One leg of a right triangle is 4 feet less than the hypotenuse. The other leg is 12 feet. Find the lengths of the three sides of the triangle.
- One leg of a right triangle is 3 more than twice the length of the other. The hypotenuse is 3 times the length of the short leg. Find the lengths of the three legs of the triangle.
- A regulation baseball diamond is a square with 90 feet between bases. How far is second base from home plate?
- Emanuel has a cardboard box that measures 20cm×10cm×8cm(length×width×height). What is the length of the diagonal from a bottom corner to the opposite top corner?
- Samuel places a ladder against his house. The base of the ladder is 6 feet from the house and the ladder is 10 feet long. How high above the ground does the ladder touch the wall of the house?
- Find the area of the triangle by using the formula A=12base×height.
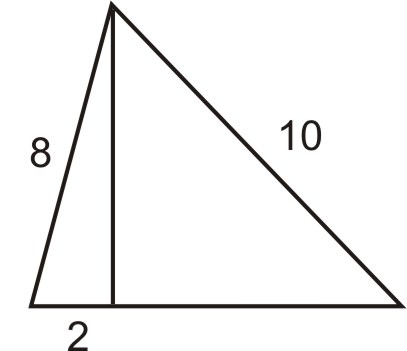
Figure 4.33.11 - Instead of walking along the two sides of a rectangular field, Mario decided to cut across the diagonal. He saves a distance that is half of the long side of the field. Find the length of the long side of the field given that the short side is 123 feet.
- Marcus sails due north and Sandra sails due east from the same starting point. In two hours, Marcus’s boat is 35 miles from the starting point and Sandra’s boat is 28 miles from the starting point. How far are the boats from each other?
- Determine the area of the circle.

Figure 4.33.12 - In a right triangle, one leg is twice as long as the other and the perimeter is 28. What are the measures of the sides of the triangle?
- Maria has a rectangular cookie sheet that measures 10inches×14inches. Find the length of the diagonal of the cookie sheet.
- Mike is loading a moving van by walking up a ramp. The ramp is 10 feet long and the bed of the van is 2.5 feet above the ground. How far does the ramp extend past the back of the van?
Quick Quiz
- Identify the origin of h(x)=√x−2+5, and then graph the function.
- Simplify 63√2 by rationalizing the denominator.
- Simplify: 4√−32. If the answer is not possible, explain why.
- What is an extraneous solution? In what situations do such solutions occur?
- Can sides with lengths of 3, 4, and 6 form a right triangle?
- Solve 5=y+3√y+6.
Vocabulary
| Term | Definition |
|---|---|
| converse of the Pythagorean Theorem | If the lengths of the three sides of a triangle satisfy the equation a2+b2=c2, then the triangle is a right triangle. |
| converse | If a conditional statement is p→q (if p, then q), then the converse is q→p (if q, then p. Note that the converse of a statement is not true just because the original statement is true. |
| Hypotenuse | The hypotenuse of a right triangle is the longest side of the right triangle. It is across from the right angle. |
| Legs of a Right Triangle | The legs of a right triangle are the two shorter sides of the right triangle. Legs are adjacent to the right angle. |
| Pythagorean Theorem | The Pythagorean Theorem is a mathematical relationship between the sides of a right triangle, given by a2+b2=c2, where a and b are legs of the triangle and c is the hypotenuse of the triangle. |
Additional Resources
Interactive Element
Video: Pythagorean Theorem - Overview
Activities: Pythagorean Theorem and its Converse Discussion Questions
Practice: Pythagorean Theorem and its Converse
Real World: SF Map - PythThm