5.10: Area of a Parallelogram
( \newcommand{\kernel}{\mathrm{null}\,}\)
Use A=bh to find area.
A parallelogram is a quadrilateral whose opposite sides are parallel.

To find the area of a parallelogram, make it into a rectangle.
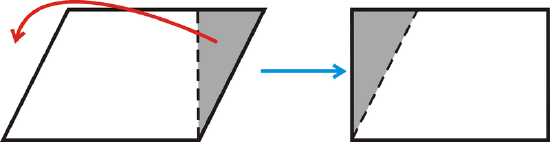
From this, we see that the area of a parallelogram is the same as the area of a rectangle. The area of a parallelogram is A=bh. The height of a parallelogram is always perpendicular to the base. This means that the sides are not the height.
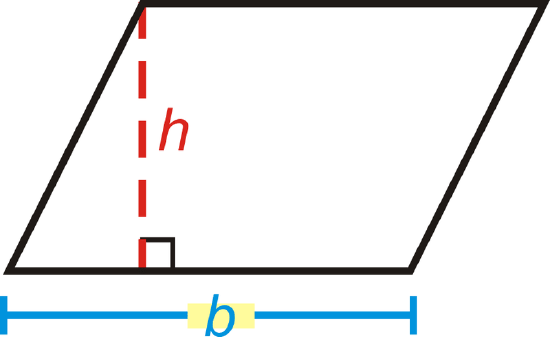
What if you were given a parallelogram and the size of its base and height? How could you find the amount of space the parallelogram takes up?
Example 5.10.1
Find the area of the parallelogram.
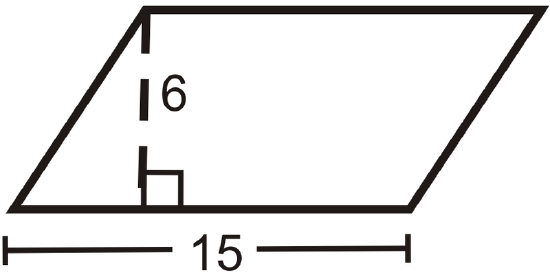
Solution
Area is 15(6)=90 un2.
Example 5.10.2
Find the area of the parallelogram with a base of 10 m and a height of 12 m.
Solution
Area is 10(12)=120\text{ m}^2\).
Example 5.10.3
Find the area of the parallelogram.
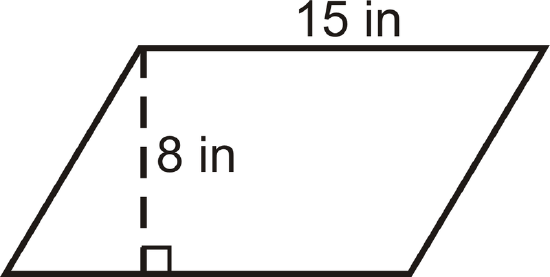
Solution
A=15⋅8=120 in2
Example 5.10.4
If the area of a parallelogram is 56 units 2 and the base is 4 units, what is the height?
Solution
Solve for the height in A=bh.
56 units =4h
14 units =h
Example 5.10.5
If the height of a parallelogram is 12 m and the area is 60m2, how wide is the base?
Solution
Solve for the base in A=bh.
60 units =12b
5 units =b
Review
- Find the area of a parallelogram with height of 20 m and base of 18 m.
- Find the area of a parallelogram with height of 12 m and base of 15 m.
- Find the area of a parallelogram with height of 40 m and base of 33 m.
- Find the area of a parallelogram with height of 32 m and base of 21 m.
- Find the area of a parallelogram with height of 25 m and base of 10 m.
Find the area of the parallelogram.
-
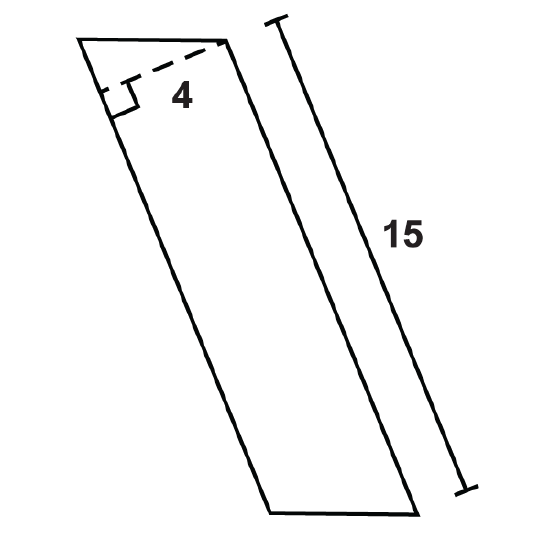
Figure 5.10.6 -
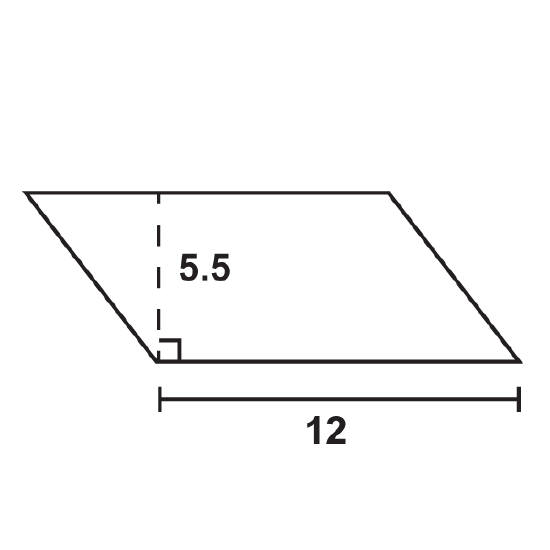
Figure 5.10.7 -
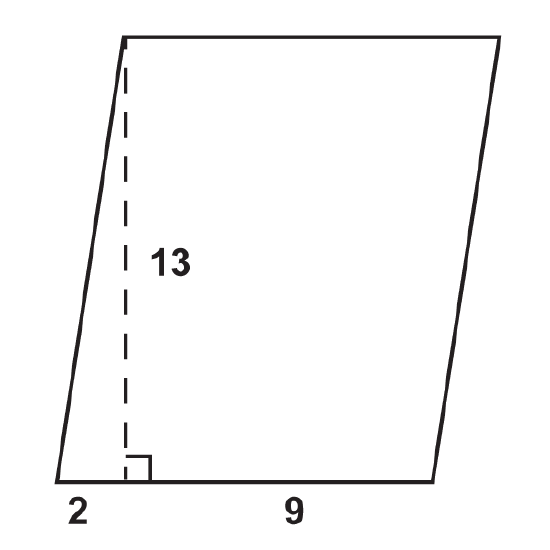
Figure 5.10.8 -

Figure 5.10.9 -

Figure 5.10.10 -

Figure 5.10.11 -
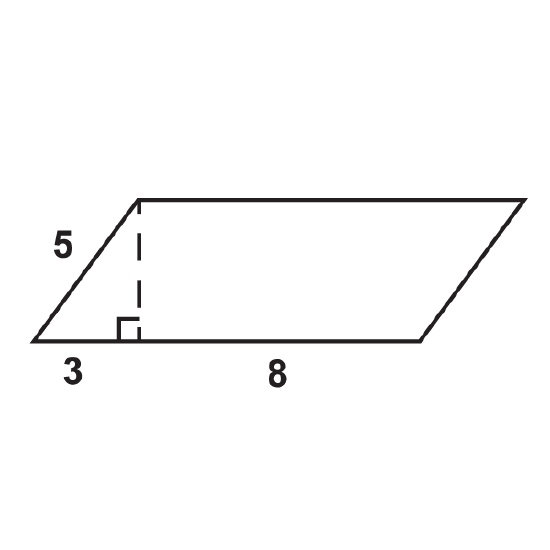
Figure 5.10.12 - If the area of a parallelogram is 42 units 2 and the base is 6 units , what is the height?
- If the area of a parallelogram is 48 units 2 and the height is 6 units , what is the base?
- If the base of a parallelogram is 9 units and the area is 108 units 2, what is the height?
- If the height of a parallelogram is 11 units and the area is 27.5 units 2, what is the base?
Vocabulary
| Term | Definition |
|---|---|
| area | The amount of space inside a figure. Area is measured in square units. |
| perimeter | The distance around a shape. The perimeter of any figure must have a unit of measurement attached to it. If no specific units are given (feet, inches, centimeters, etc), write units. |
| Parallelogram | A parallelogram is a quadrilateral with two pairs of parallel sides. |
| Area of a Parallelogram | The area of a parallelogram is equal to the base multiplied by the height: A=bh. The height of a parallelogram is always perpendicular to the base (the sides are not the height). |
Additional Resources
Interactive Element
Video: Area of a Parallelogram (Whole Numbers)
Activities: Area of a Parallelogram Discussion Questions
Study Aids: Triangles and Quadrilaterals Study Guide
Practice: Area of a Parallelogram
Real World: Perimeter