5.13: Trapezoids
( \newcommand{\kernel}{\mathrm{null}\,}\)
Determine unknown angle measurements of quadrilaterals with exactly one pair of parallel sides.
A trapezoid is a quadrilateral with exactly one pair of parallel sides.
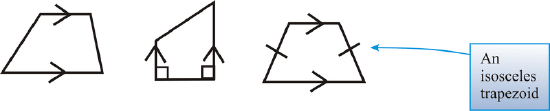
An isosceles trapezoid is a trapezoid where the non-parallel sides are congruent.
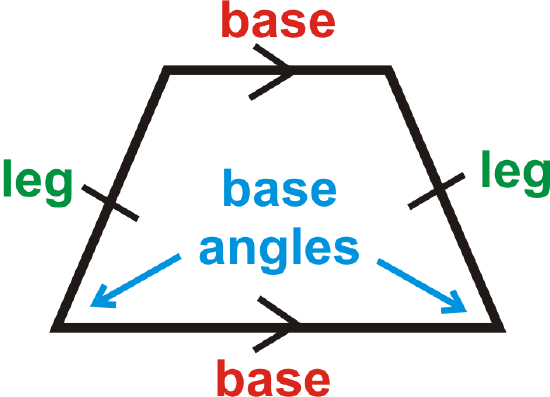
The base angles of an isosceles trapezoid are congruent. If \(ABCD\) is an isosceles trapezoid, then ∠A≅∠B and ∠C≅∠D.
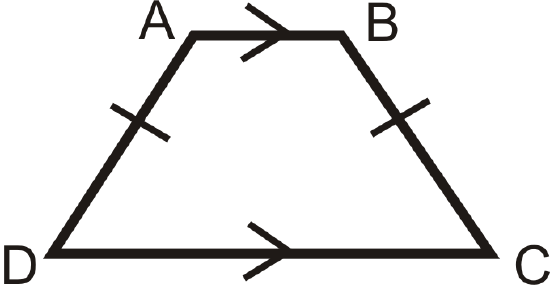
The converse is also true. If a trapezoid has congruent base angles, then it is an isosceles trapezoid. The diagonals of an isosceles trapezoid are also congruent. The midsegment (of a trapezoid) is a line segment that connects the midpoints of the non-parallel sides:
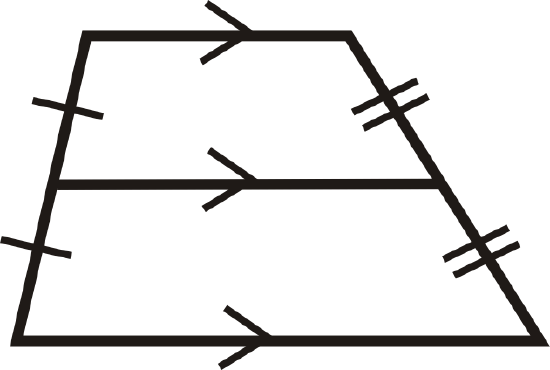
There is only one midsegment in a trapezoid. It will be parallel to the bases because it is located halfway between them.
Midsegment Theorem: The length of the midsegment of a trapezoid is the average of the lengths of the bases.
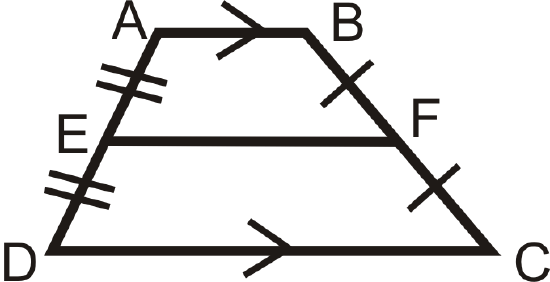
If ¯EF is the midsegment, then EF=AB+CD2.
What if you were told that the polygon ABCD is an isosceles trapezoid and that one of its base angles measures 38∘? What can you conclude about its other base angle?
For Examples 1 and 2, use the following information:
\(TRAP\) is an isosceles trapezoid.

Example 5.13.1
Find m∠TPA.
Solution
∠TPZ≅∠RAZ so m∠TPA=20∘+35∘=55∘.
Example 5.13.2
Find m∠ZRA.
Solution
Since m∠PZA=110∘, m∠RZA=70∘ because they form a linear pair. By the Triangle Sum Theorem, m∠ZRA=90∘.
Example 5.13.3
Look at trapezoid TRAP below. What is m∠A?
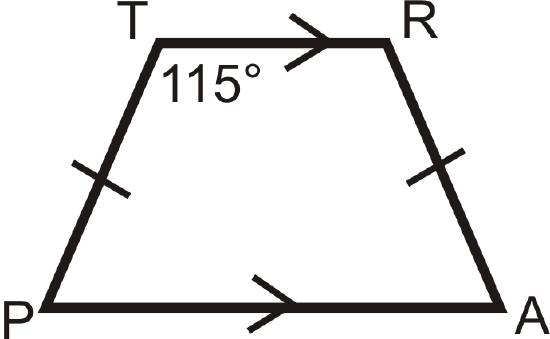
Solution
TRAP is an isosceles trapezoid. m∠R=115∘ also.
To find \(m\angle , set up an equation.
115∘+115∘+m∠A+m∠P=360∘230∘+2m∠A=360∘→m∠A=m∠P2m∠A=130∘m∠A=65∘
Notice that m∠R+m∠A=115∘+65∘=180∘. These angles will always be supplementary because of the Consecutive Interior Angles Theorem.
Example 5.13.4
Is ZOID an isosceles trapezoid? How do you know?
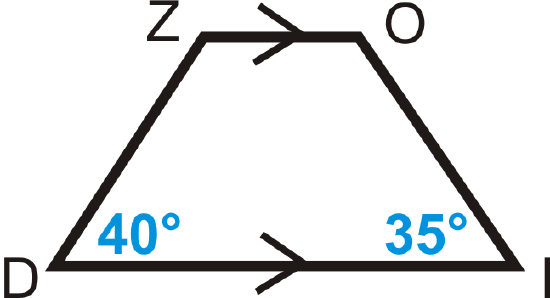
Solution
40∘≠35∘, ZOID is not an isosceles trapezoid.
Example 5.13.5
Find x. All figures are trapezoids with the midsegment marked as indicated.
-
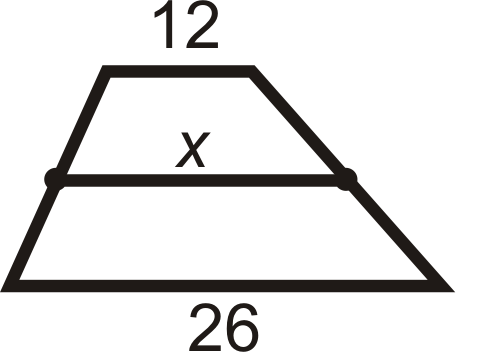
Figure 5.13.10
-
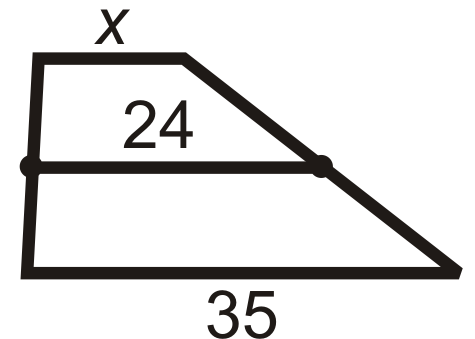
Figure 5.13.10 -
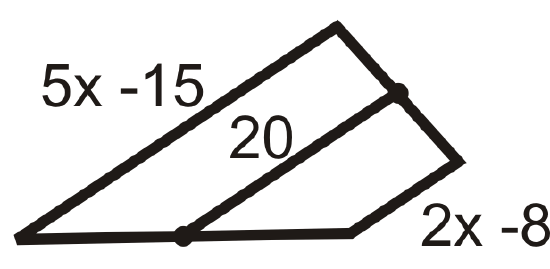
Figure 5.13.11
Solution
- x is the average of 12 and 26. 12+262=382=19
- 24 is the average of x and 35.
x+352=24x+35=48x=13
- 20 is the average of 5x−15 and 2x−8.
5x−15+2x−82=207x−23=407x=63x=9
Review
1. Can the parallel sides of a trapezoid be congruent? Why or why not?
For questions 2-8, find the length of the midsegment or missing side.
-
Figure 5.13.12 -
Figure 5.13.13 -
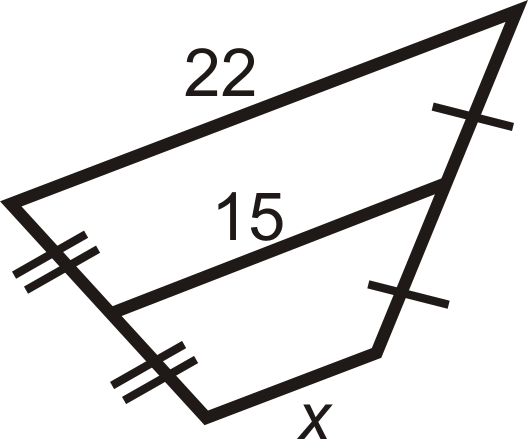
Figure 5.13.14 -
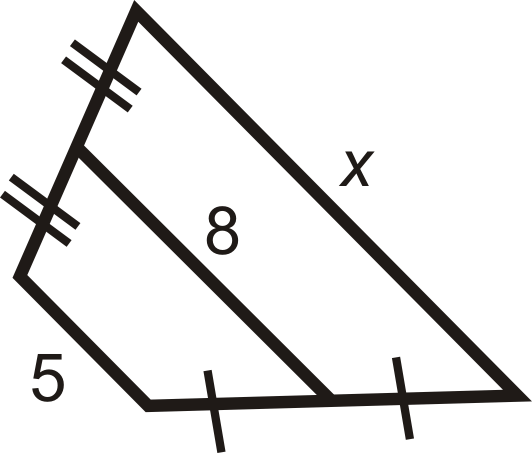
Figure 5.13.15 -
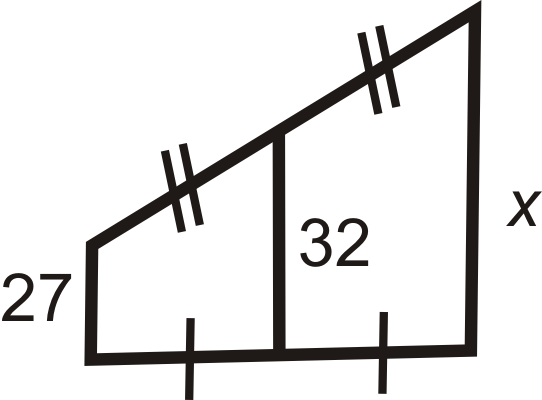
Figure 5.13.16 -
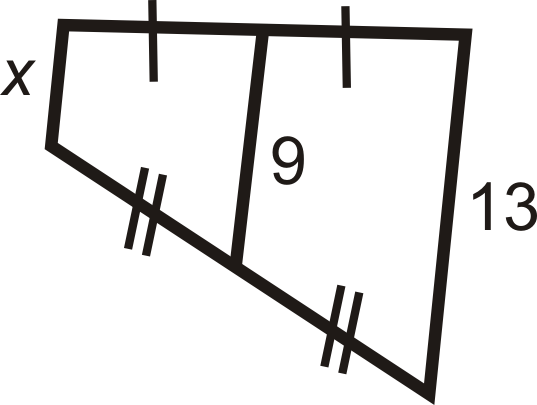
Figure 5.13.17
Find the value of the missing variable(s).
-
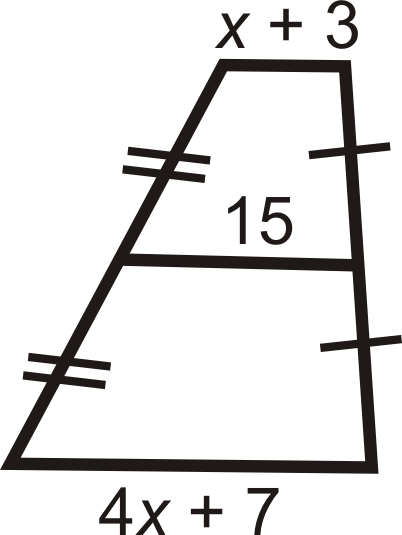
Figure 5.13.18
Find the lengths of the diagonals of the trapezoids below to determine if it is isosceles.
- A(−3,2), B(1,3), C(3,−1), D(−4,−2)
- A(−3,3), B(2,−2), C(−6,−6), D(−7,1)
Review (Answers)
To see the Review answers, open this PDF file and look for section 6.6.
Vocabulary
| Term | Definition |
|---|---|
| isosceles trapezoid | An isosceles trapezoid is a trapezoid where the non-parallel sides are congruent. |
| midsegment (of a trapezoid) | A line segment that connects the midpoints of the non-parallel sides. |
| trapezoid | A quadrilateral with exactly one pair of parallel sides. |
| Diagonal | A diagonal is a line segment in a polygon that connects nonconsecutive vertices |
| midsegment | A midsegment connects the midpoints of two sides of a triangle or the non-parallel sides of a trapezoid. |
Additional Resources
Interactive Element
Video: Trapezoids Examples - Basic
Activities: Trapezoids Discussion Questions
Study Aids: Trapezoids and Kites Study Guide
Practice: Trapezoids
Real World: Trapezoids in Timbuktu


