Skip to main content
K12 LibreTexts

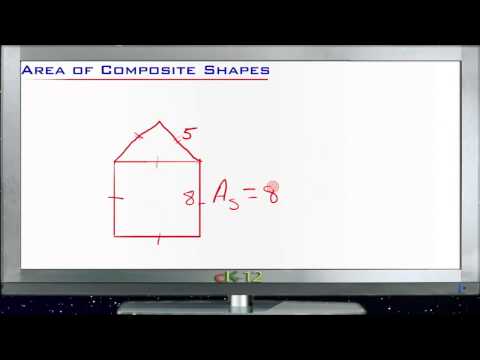
5.18: Area and Perimeter of Composite Shapes

  1. Last updated
  2. Save as PDF
  • Page ID
    5002

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    Find area and perimeter of figures made up of two or more common shapes.

    Area of Composite Shapes

    Perimeter is the distance around a shape. The perimeter of any figure must have a unit of measurement attached to it. If no specific units are given (feet, inches, centimeters, etc.), write “units.”

    Area is the amount of space inside a figure. If two figures are congruent, they have the same area (Congruent Areas Postulate).

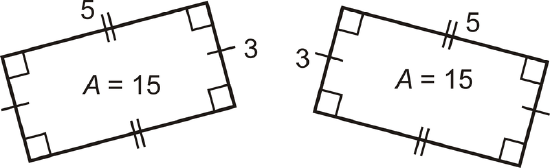
    f-d_4103fe1784f847c617fdd3ca111b42aa884f5923ad74e8cfdf60cc88+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{1}\)

    A composite shape is a shape made up of other shapes. To find the area of such a shape, simply find the area of each part and add them up.

    Area Addition Postulate: If a figure is composed of two or more parts that do not overlap each other, then the area of the figure is the sum of the areas of the parts.

    Consider a basic house drawn as a triangle on top of a square. How could you find the area of this composite shape?

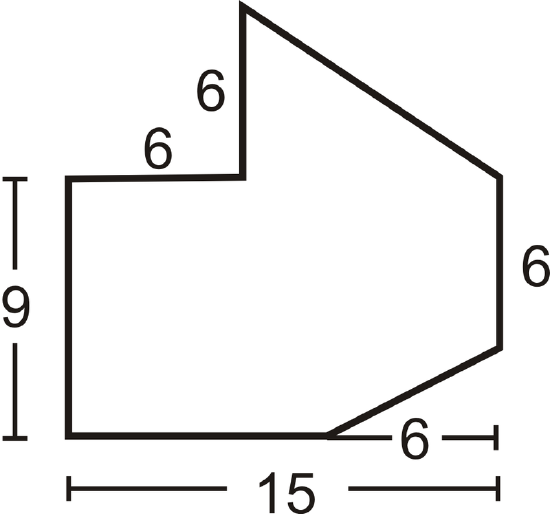
    Example \(\PageIndex{1}\)

    Find the area of the figure below. All angles that look like right angles are right angles.

    f-d_1f1795886afba0951483579c32edf05f837afeabd587ab546cb1cb3b+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{2}\)

    Solution

    Divide the figure into a triangle and a rectangle with a small rectangle cut out of the lower right-hand corner.

    f-d_3bcfb82613d3ef4d5b567dae818e27adc4263f3408571d9b769e1b70+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{3}\)

    \(A=A_{\text{top triangle}}+A_{\text{rectangle}}−A_{\text{small triangle}} \\ A=(\dfrac{1}{2} \cdot 6\cdot 9)+(9\cdot 15))−(\dfrac{1}{2}\cdot 3\cdot 6) \\ A&=27+135−9 \\ A&=153\text{ units}^2\)

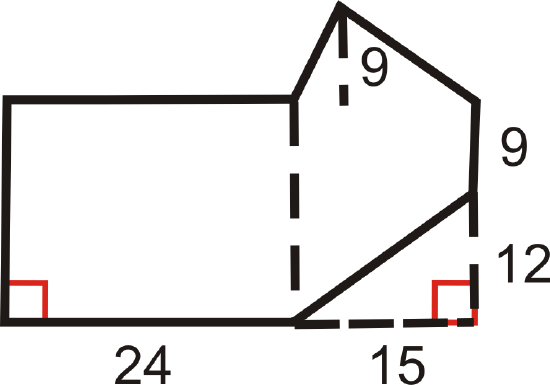
    Example \(\PageIndex{2}\)

    Divide the shape into two rectangles and one triangle. Find the area of the two rectangles and triangle. All angles that look like right angles are right angles.

    f-d_a79d378075956d908990bf9fb43237cc3e2fbfbaae24c3c315606846+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{4}\)

    Solution

    Rectangle #1: \(\text{Area} =24(9+12)=504\text{ units}^2\)

    Rectangle #2: \(\text{Area}=15(9+12)=315\text{ units}^2\)

    Triangle: \(\text{Area} =15(9)2=67.5\text{ units}^2\)

    Example \(\PageIndex{3}\)

    Find the area of the entire shape from Example 2 (you will need to subtract the area of the small triangle in the lower right-hand corner).

    Solution

    \(\text{Total Area} =504+315+67.5−\dfrac{15(12)}{2}=796.5 \text{ units}^2\)

    Example \(\PageIndex{4}\)

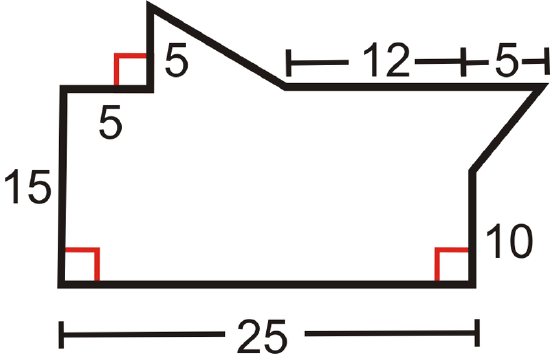
    f-d_5fcc9e2bf4aaa5a3c0d21db97bfcfac3b0f000b1cab94134f3f4d4d8+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{5}\)

    All angles that look like right angles are right angles.

    1. Divide the shape into two triangles and one rectangle.
    2. Find the area of the two triangles and rectangle.
    3. Find the area of the entire shape.

    Solution

    1. One triangle on the top and one on the right, the rest of the shape is a rectangle.
    2. The area of the triangle on top is \(\dfrac{8(5)}{2}=20\text{ units}^2\). The area of the triangle on the right is \(\dfrac{5(5)}{2}=12.5\text{ units}^2\). The area of the rectangle is \(375\text{ units}^2\).
    3. The total area is \(407.5\text{ units}^2\).

    Review

    Use the picture below for questions 1-4. The composite shape is formed of a square within a square.

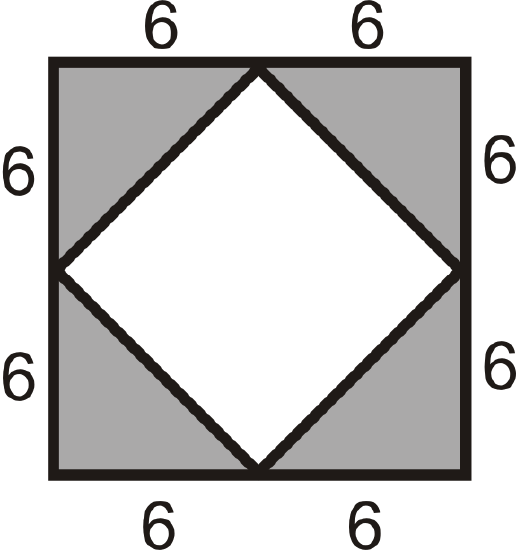
    f-d_9593292257f4fa65b53ae9cdf1261fbd00bfb3be1d7dd7b32c597979+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{6}\)
    1. Find the area of the outer square.
    2. Find the area of one grey triangle.
    3. Find the area of all four grey triangles.
    4. Find the area of the inner square.

    Find the areas of the figures below. You may assume all sides are perpendicular.

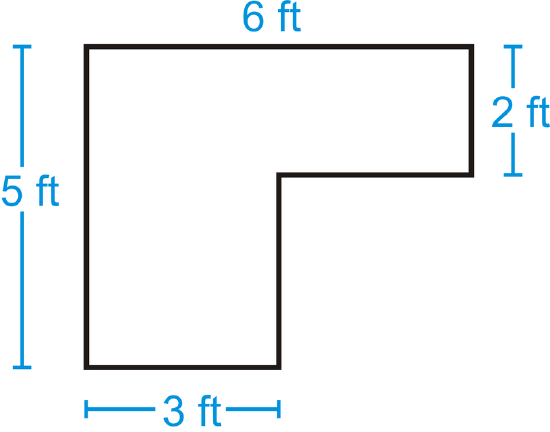
    1. f-d_1f85269bdbc32d34e48721c78ba8166ab24c2a13b1d938a73bc3453c+IMAGE_TINY+IMAGE_TINY.png
      Figure \(\PageIndex{7}\)
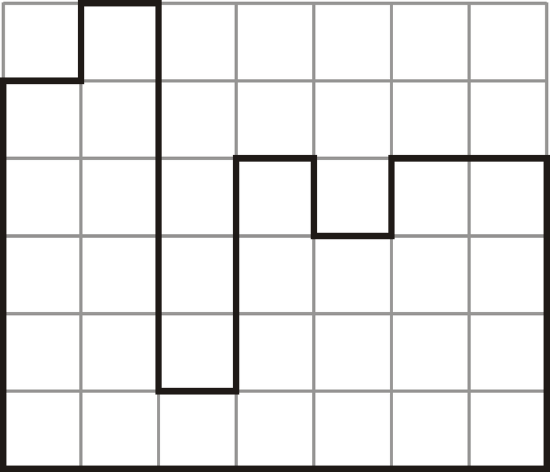
    2. f-d_712e4faec92b44f0a0157977ec74e34490315a27ba16279d19807c6c+IMAGE_TINY+IMAGE_TINY.png
      Figure \(\PageIndex{8}\)

    Find the areas of the composite figures.

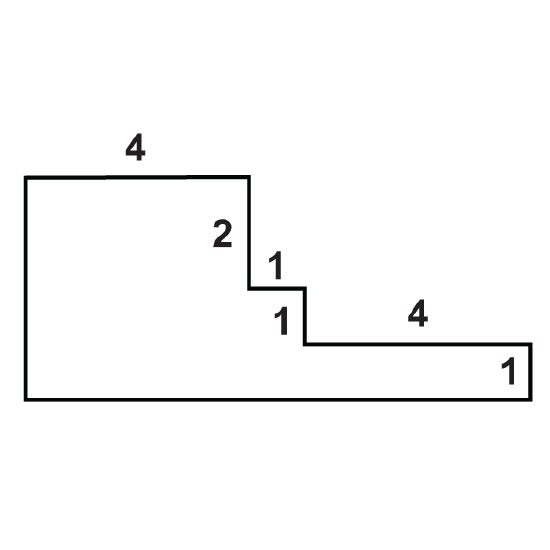
    1. f-d_71857e1e9a2c3464d7e0d122ccfd8eae7dedeaa4cfb9912ed3791321+IMAGE_TINY+IMAGE_TINY.png
      Figure \(\PageIndex{9}\)
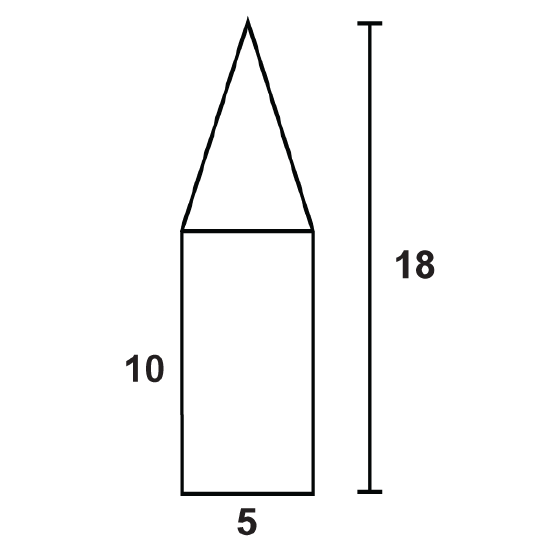
    2. f-d_723adbdd8763dc3b0035a822f4ce043ebdeb5db522b587c45d8a6176+IMAGE_TINY+IMAGE_TINY.png
      Figure \(\PageIndex{10}\)
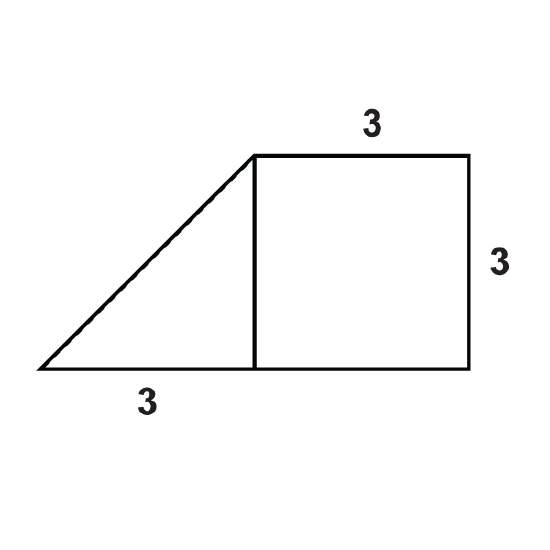
    3. f-d_eb96e49c999a24ee9bb5f536d7daf7d750116c8d85bf93b6380d0e01+IMAGE_TINY+IMAGE_TINY.png
      Figure \(\PageIndex{11}\)
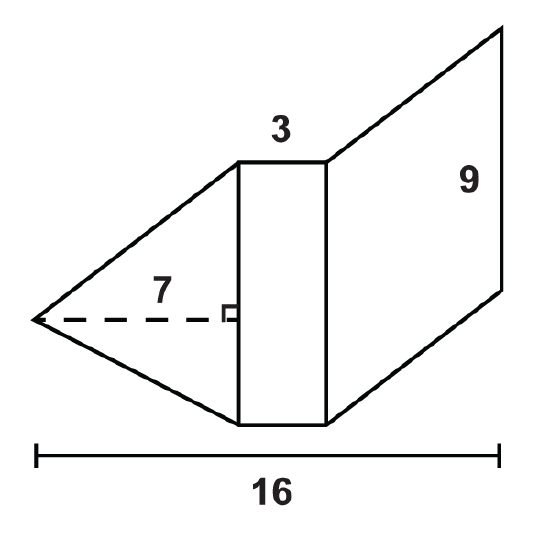
    4. f-d_ebaa4db79f353dbe7138f591000b9257241437b3b38616f65fb56358+IMAGE_TINY+IMAGE_TINY.png
      Figure \(\PageIndex{12}\)
    5. f-d_91a4addc1cabf13ea8e143be6f8fda889969908418c7a4ce6b2d8fbc+IMAGE_TINY+IMAGE_TINY.png
      Figure \(\PageIndex{13}\)
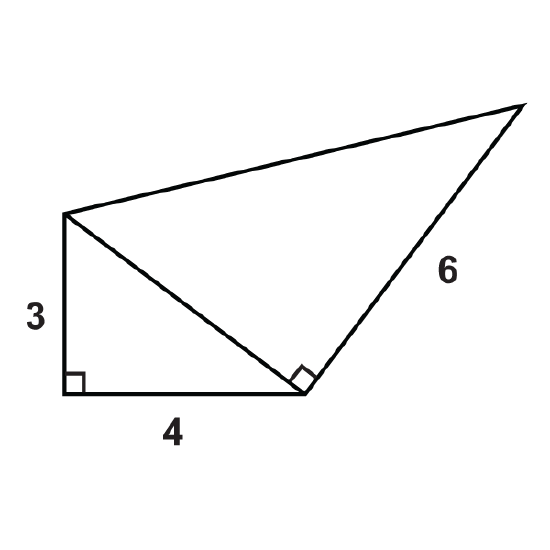
    6. f-d_a9cdf3b23c37f87c98221b7fba8a8545f1f281191d55d9a9d272da72+IMAGE_TINY+IMAGE_TINY.png
      Figure \(\PageIndex{14}\)

    Use the figure to answer the questions.

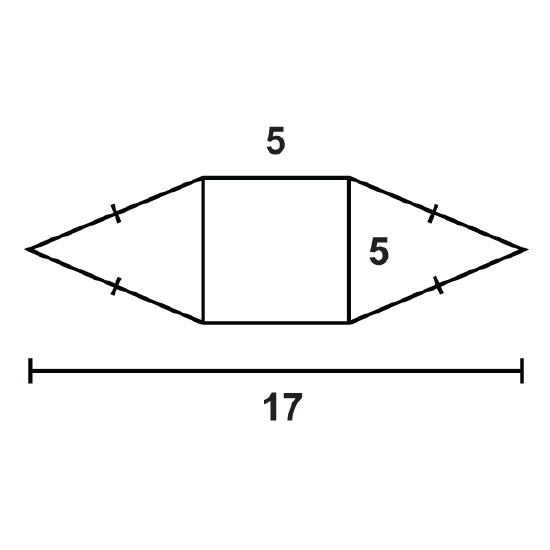
    f-d_dfbf75487b2e31fe2a63bc3ce8fde39eecd21f622c4b5d058522fae4+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{15}\)
    1. What is the area of the square?
    2. What is the area of the triangle on the left?
    3. What is the area of the composite figure?

    Review (Answers)

    To see the Review answers, open this PDF file and look for section 10.6.

    Vocabulary

    Term Definition
    area The amount of space inside a figure. Area is measured in square units.
    composite shape A shape made up of other shapes.
    perimeter The distance around a shape. The perimeter of any figure must have a unit of measurement attached to it. If no specific units are given (feet, inches, centimeters, etc), write units.
    Composite A number that has more than two factors.

    Additional Resources

    Interactive Element

    Video: Area of a Triangle (Whole Numbers)

    Activites: Area of Composite Shapes Discussion Questions

    Study Aids: Perimeter and Area Study Guide

    Practice: Area and Perimeter of Composite Shapes

    This page titled 5.18: Area and Perimeter of Composite Shapes is shared under a CK-12 license and was authored, remixed, and/or curated by CK-12 Foundation via source content that was edited to the style and standards of the LibreTexts platform; a detailed edit history is available upon request.

    CK-12 Foundation
    LICENSED UNDER
    CK-12 Foundation is licensed under CK-12 Curriculum Materials License