7.14: Theorems Involving Similarity
( \newcommand{\kernel}{\mathrm{null}\,}\)
Prove and use the Triangle Proportionality, Triangle Angle Bisector, and Pythagorean Theorems.
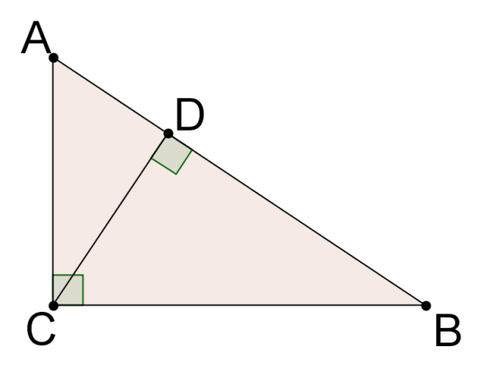
Can you find any similar triangles in the picture below?
Theorems on Similar Triangles
If two triangles are similar, then their corresponding angles are congruent and their corresponding sides are proportional. There are many theorems about triangles that you can prove using similar triangles.
- Triangle Proportionality Theorem: A line parallel to one side of a triangle divides the other two sides of the triangle proportionally. This theorem and its converse will be explored and proved in #1 and #2, and the Review exercises.
- Triangle Angle Bisector Theorem: The angle bisector of one angle of a triangle divides the opposite side of the triangle into segments proportional to the lengths of the other two sides of the triangle. This theorem will be explored and proved in #3.
- Pythagorean Theorem: For a right triangle with legs a and b and hypotenuse c, a2+b2=c2. This theorem will be explored and proved in the Examples problems.
Let's take a look at some problems about proving triangle similarity.
1. Prove that ΔADE∼ΔABC.
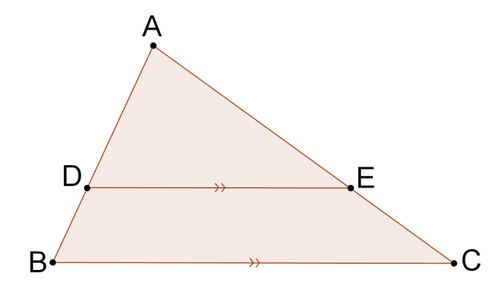
The two triangles share ∠A. Because ¯DE∥¯BC, corresponding angles are congruent. Therefore, ∠ADE≅∠ABC. The two triangles have two pairs of congruent angles. Therefore, ΔADE∼ΔABC by AA\sim\).
2. Use your result from #1 to prove that ABAD=ACAE. Then, use algebra to show that DBAD=ECAE.
\(\Delta ADE\sim \Delta ABC\) which means that corresponding sides are proportional. Therefore, ABAD=ACAE. Now, you can use algebra to show that the second proportion must be true. Remember that AB=AD+DB and AC=AE+EC.
ABAD=ACAE→AD+DBAD=AE+ECAE→1+DBAD=1+ECAE→DBAD=ECAE
You have now proved the triangle proportionality theorem: a line parallel to one side of a triangle divides the other two sides of the triangle proportionally.
3. Consider ΔABC with ¯AE the angle bisector of ∠BAC and point D constructed so that ¯DC∥¯AE. Prove that EBBA=ECCA.

By the triangle proportionality theorem, EBEC=BAAD. Multiply both sides of this proportion by ECBA.
(ECBA)⋅EBEC→EBBA=BAAD⋅(ECBA)=ECAD
Now all you need to show is that AD=CA in order to prove the desired result.
- Because ¯AE is the angle bisector of ∠BAC, ∠BAE≅∠EAC.
- Because ¯DC∥¯AE, ∠BAE≅∠BDC (corresponding angles).
- Because ¯DC∥¯AE, ∠EAC≅∠DCA (alternate interior angles).
- Thus, ∠BDC≅∠DCA by the transitive property.
Therefore, ΔADC is isosceles because its base angles are congruent and it must be true that ¯AD≅¯CA. This means that AD=CA. Therefore:
EBBA=ECCA
This proves the triangle angle bisector theorem: the angle bisector of one angle of a triangle divides the opposite side of the triangle into segments proportional to the lengths of the other two sides of the triangle.
Example 7.14.1
Earlier, you were asked can you find any similar triangles in the picture below.

Solution
There are three triangles in this picture: ΔBAC, ΔBCD, ΔCAD. All three triangles are right triangles so they have one set of congruent angles (the right angle). ΔBAC and ΔBCD share ∠B, so ΔBAC∼ΔBCDby\(AA∼. Similarly, ΔBAC and ΔCAD share ∠C, so ΔBAC∼ΔCAD by AA∼. By the transitive property, all three triangles must be similar to one another.
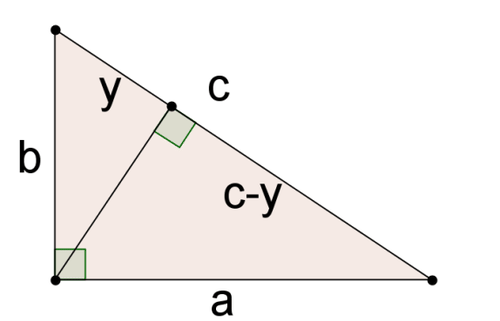
The large triangle above has sides a, b, and c. Side c has been divided into two parts: y and c−y. In the Concept Problem Revisited you showed that the three triangles in this picture are similar.
Example 7.14.2
Explain why ac=c−ya.
Solution
When triangles are similar, corresponding sides are proportional. Carefully match corresponding sides and you see that ac=c−ya.
Example 7.14.3
Explain why bc=yb.
Solution
When triangles are similar, corresponding sides are proportional. Carefully match corresponding sides and you see that bc=yb.
Example 7.14.4
Use the results from #2 and #3 to show that a2+b2=c2.
Solution
Cross multiply to rewrite each equation. Then, add the two equations together.
ac=c−ya→a2=c2−cybc=yb→b2=cy→a2+b2=c2−cy+cy→a2+b2=c2
You have just proved the Pythagorean Theorem using similar triangles.
Review
Solve for x in each problem.
-
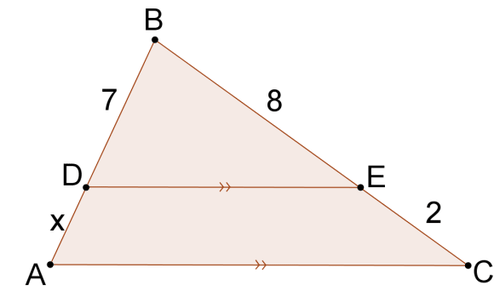
Figure 7.14.6 -
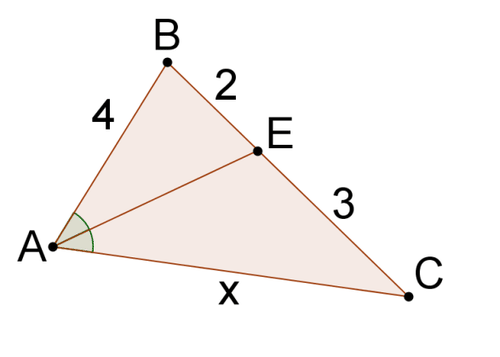
Figure 7.14.7 -
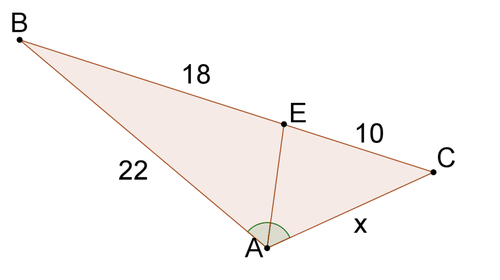
Figure 7.14.8 -
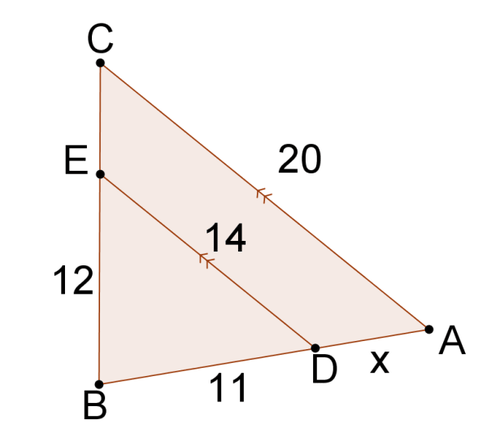
Figure 7.14.9 -

Figure 7.14.10 -

Figure 7.14.11 -
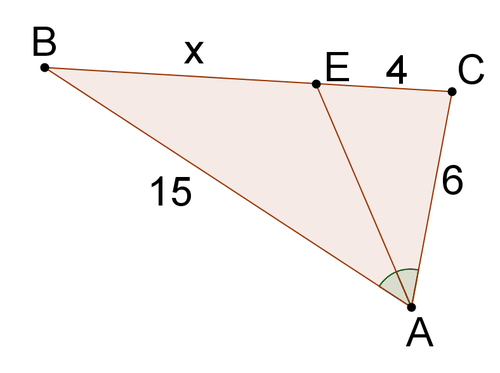
Figure 7.14.12
Use the picture below for #8-#10.
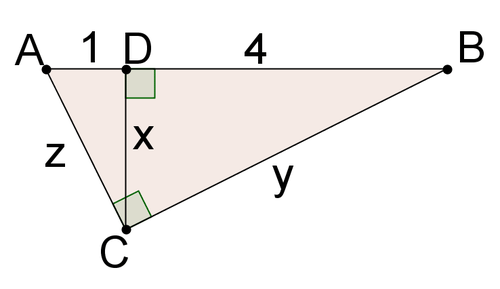
8. Solve for x.
9. Solve for z.
10. Solve for y.
Use the picture below for #11-#13.
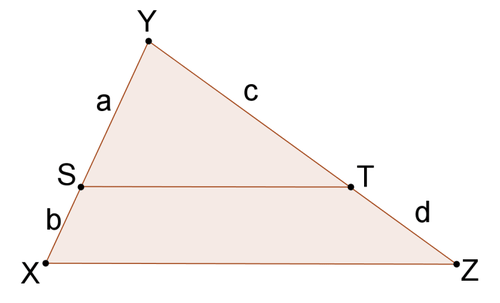
11. Assume that ba=dc. Use algebra to show that b+aa=d+cc.
12. Prove that ΔYST∼ΔYXZ
13. Prove that ¯ST∥¯XZ
14. Prove that a segment that connects the midpoints of two sides of a triangle will be parallel to the third side of the triangle.
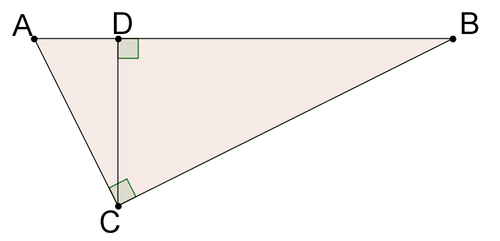
15. Prove the Pythagorean Theorem using the picture below.
Review (Answers)
To see the Review answers, open this PDF file and look for section 6.6.
Vocabulary
| Term | Definition |
|---|---|
| Pythagorean Theorem | The Pythagorean Theorem is a mathematical relationship between the sides of a right triangle, given by a2+b2=c2, where a and b are legs of the triangle and c is the hypotenuse of the triangle. |
| Triangle Proportionality Theorem | The Triangle Proportionality Theorem states that if a line is parallel to one side of a triangle and it intersects the other two sides, then it divides those sides proportionally. |
| Triangle Angle Bisector Theorem | The angle bisector of one angle of a triangle divides the opposite side of the triangle into segments proportional to the lengths of the other two sides of the triangle. |


