4.40: Applications of the Distance Formula
- Page ID
- 4978
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)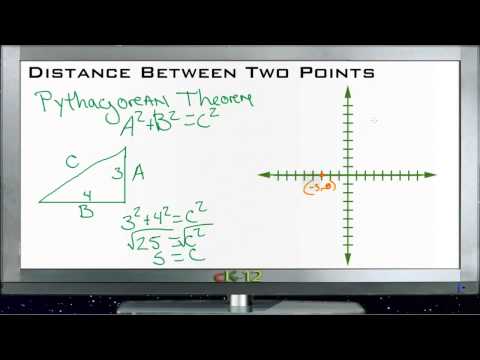Length between two points based on a right triangle.
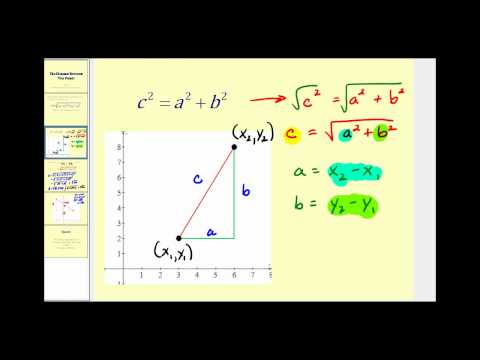
Distance Formula in the Coordinate Plane
The distance between two points \((x_1, y_1)\) and \((x_2,y_2)\) can be defined as \(d=\sqrt{(x_2−x_1)^2+(y_2−y_1)^2}\). This is called the distance formula. Remember that distances are always positive!
What if you were given the coordinates of two points? How could you find how far apart these two points are?
Example \(\PageIndex{1}\)
Find the distance between \((-2, -3)\) and \((3, 9)\).
Solution
Use the distance formula, plug in the points, and simplify.
\(\begin{align*}d&=\sqrt{(3−(−2))^2+(9−(−3))^2} \\ &=\sqrt{(5)^2+(12)^2} \\ &= \sqrt{25+144} \\ &= \sqrt{169}=13\text{ units }\end{align*}\)
Example \(\PageIndex{2}\)
Find the distance between \((12, 26)\) and \((8, 7)\).
Solution
Use the distance formula, plug in the points, and simplify.
\(\begin{align*}d&=\sqrt{(8−12)^2+(7−26)^2} \\ &= \sqrt{(−4)^2+(−19)^2} \\ &= \sqrt{16+361} \\ &= \sqrt{377}\approx 19.42\text{ units }\end{align*}\)
Example \(\PageIndex{3}\)
Find the distance between \((4, -2)\) and \((-10, 3)\).
Solution
Plug in \((4, -2)\) for \((x_1, y_1)\) and \((-10, 3)\) for \((x_2,y_2)\) and simplify.
\(\begin{align*}d&=\sqrt{(−10−4)^2+(3+2)^2} \\ &= \sqrt{(−14)^2+(5)^2} \\ &= \sqrt{196+25} \\ &= \sqrt{221}\approx 14.87\text{ units }\end{align*}\)
Example \(\PageIndex{4}\)
Find the distance between \((3, 4)\) and \((-1, 3)\).
Solution
Plug in (3, 4)\) for \((x_1, y_1)\) and \((-1, 3)\) for \((x_2,y_2)\) and simplify.
\(\begin{align*}d &=\sqrt{(−1−3)^2+(3−4)^2} \\ &= \sqrt{(−4)^2+(−1)^2} \\ &= \sqrt{16+1} \\ &= \sqrt{17} \approx 4.12\text{ units }\end{align*}\)
Example \(\PageIndex{5}\)
Find the distance between \((4, 23)\) and \((8, 14)\).
Solution
Plug in \((4, 23)\) for \((x_1, y_1)\) and \((8, 14)\) for \((x_2,y_2)\) and simplify.
\(\begin{align*} d&=\sqrt{(8−4)^2+(14−23)^2} \\ &=\sqrt{(4)^2+(−9)^2} \\ &=\sqrt{16+81} \\ & =\sqrt{97} \approx 9.85\text{ units }\end{align*} \)
Review
Find the distance between each pair of points. Round your answer to the nearest hundredth.
- \((4, 15)\) and \((-2, -1)\)
- \((-6, 1)\) and \((9, -11)\)
- \((0, 12)\) and \((-3, 8)\)
- \((-8, 19)\) and \((3, 5)\)
- \((3, -25)\) and \((-10, -7)\)
- \((-1, 2)\) and\((8, -9)\)
- \((5, -2)\) and \((1, 3)\)
- \((-30, 6)\) and \((-23, 0)\)
- \((2, -2)\) and \((2, 5)\)
- \((-9, -4)\) and \((1, -1) \)
Review (Answers)
To see the Review answers, open this PDF file and look for section 3.10.
Resource
Vocabulary
| Term | Definition |
|---|---|
| Distance Formula | The distance between two points \((x_1, y_1)\) and \((x_2,y_2)\) can be defined as \(d=\sqrt{(x_2−x_1)^2+(y_2−y_1)^2}\). |
| Pythagorean Theorem | The Pythagorean Theorem is a mathematical relationship between the sides of a right triangle, given by \(a^2+b^2=c^2\), where \(a\) and \(b\) are legs of the triangle and c is the hypotenuse of the triangle. |
Additional Resources
Interactive Element
Video: The Distance Formula
Activities: Distance Formula in the Coordinate Plane Discussion Questions
Study Aids: Segments Study Guide
Practice: Applications of the Distance Formula
Real World: Distance Formula in the Coordinate Plane