8.9: Translation Notation
( \newcommand{\kernel}{\mathrm{null}\,}\)
Graphical introduction to image translations. Learn to use notation to describe mapping rules,and graph images given preimage and translation
Rules for Translations
Jack describes a translation as point moving from J(−2,6) to J′(4,9). Write the mapping rule to describe this translation for Jack.
In geometry, a transformation is an operation that moves, flips, or changes a shape (called the preimage) to create a new shape (called the image). A translation is a type of transformation that moves each point in a figure the same distance in the same direction. Translations are often referred to as slides. You can describe a translation using words like "moved up 3 and over 5 to the left" or with notation. There are two types of notation to know.
- One notation looks like T(3,5). This notation tells you to add 3 to the x values and add 5 to the y values.
- The second notation is a mapping rule of the form (x,y)→(x−7,y+5). This notation tells you that the x and y coordinates are translated to x−7 and y+5.
The mapping rule notation is the most common.
Interactive Element
Let's write a mapping rule for each of the following translations:
- Sarah describes a translation as point P moving from P(−2,2) to P′(1,−1).
In general, P(x,y)→P′(x+a,y+b).
In this case, P(−2,2)→P′(−2+a,2+b) or P(−2,2)→P′(1,−1)
Therefore:
−2+a=1 and 2+b=−1a=3b=−3
The rule is: (x,y)→(x+3,y−3)
- Mikah describes a translation as point D in a diagram moving from D(1,−5) to D′(−3,1).
In general, P(x,y)→P′(x+a,y+b).
In this case, D(1,−5)→D′(1+a,−5+b) or D(1,−5)→D′(−3,1)
Therefore:
1+a=−3 and −5+b=1a=−4b=6
The rule is: (x,y)→(x−4,y+6)
- The translation of the preimage A to the translated image J in the diagram below:
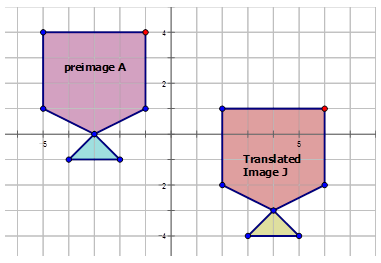
First, pick a point in the diagram to use to see how it is translated.
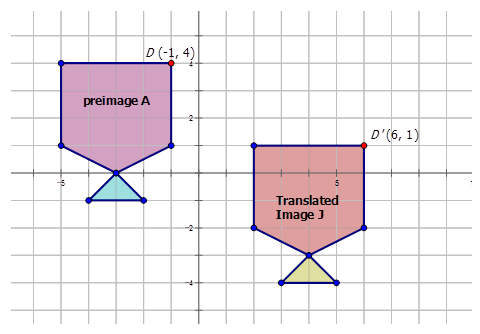
D:(−1,4)D′:(6,1)
D(x,y)→D′(x+a,y+b)
So: D(−1,4)→D′(−1+a,4+b) or D(−1,4)→D′(6,1)
Therefore:
−1+a=6 and 4+b=1a=7b=−3
The rule is: (x,y)→(x+7,y−3)
Example 8.9.1
Earlier, you were told that Jack described a translation as point J moving from J(−2,6) to J′(4,9). What is the mapping rule that describes this translation?
Solution
(x,y)→(x+6,y+3)
Example 8.9.2
Write the mapping rule that represents the translation of the red triangle to the translated green triangle in the diagram below.
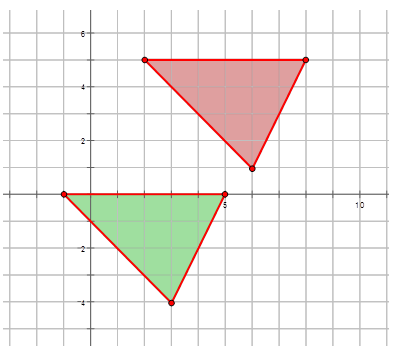
Solution
(x,y)→(x−3,y−5)
Example 8.9.3
The following pattern is part of wallpaper found in a hotel lobby. Write the mapping rule that represents the translation of one blue trapezoid to a translated blue trapezoid shown in the diagram below.

Solution
If you look closely at the diagram below, there two pairs of trapezoids that are translations of each other. Therefore you can choose one blue trapezoid that is a translation of the other and pick a point to find out how much the shape has moved to get to the translated position.
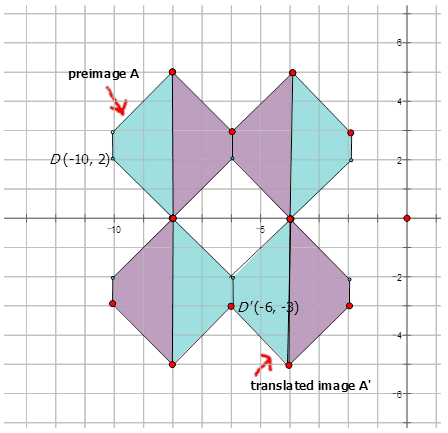
For those two trapezoids: (x,y)→(x+4,y−5)
Review
Write the mapping rule to describe the movement of the points in each of the translations below.
- S(1,5)→S′(2,7)
- W(−5,−1)→W′(−3,1)
- Q(2,−5)→Q′(−6,3)
- M(4,3)→M′(−2,9)
- B(−4,−2)→B′(2,−2)
- A(2,4)→A′(2,6)
- C(−5,−3)→C′(−3,4)
- D(4,−1)→D′(−4,2)
- Z(7,2)→Z′(−3,6)
- L(−3,−2)→L′(4,−1)
Write the mapping rule that represents the translation of the preimage to the image for each diagram below.
-
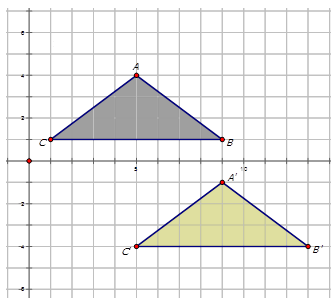
Figure 8.9.6 -
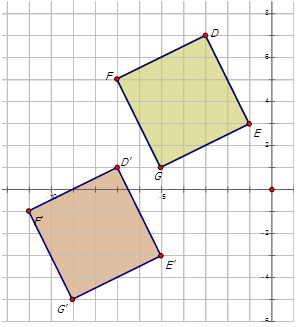
Figure 8.9.7 -
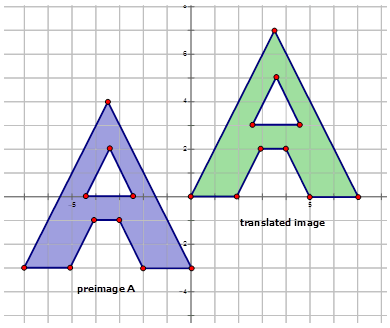
Figure 8.9.8 -

Figure 8.9.9 -
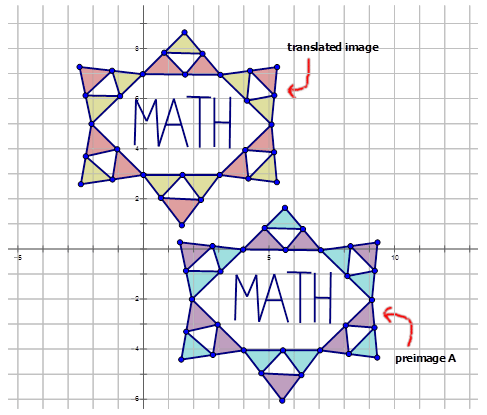
Figure 8.9.10
Review (Answers)
To see the Review answers, open this PDF file and look for section 10.3.
Additional Resources
Interactive Element
Video: Quadrants of Coordinate Plane
Practice: Translation Notation

