Skip to main content
K12 LibreTexts

8.12: Geometry Software and Graphing Rotations

  1. Last updated
  2. Save as PDF
  • Page ID
    6056

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    Graph rotated images given preimage and number of degrees. Perform rotations using Geogebra.

    Quadrilateral \(WXYZ\) has coordinates \(W(−5,−5)\), \(X(−2,0), \(Y(2,3)\) and \(Z(−1,3)\). Draw the quadrilateral on the Cartesian plane. Rotate the image \(110^{\circ}\) counterclockwise about the point \(X\). Show the resulting image.

    Graphs of Rotations

    In geometry, a transformation is an operation that moves, flips, or changes a shape to create a new shape. A rotation is an example of a transformation where a figure is rotated about a specific point (called the center of rotation), a certain number of degrees.

    For now, in order to graph a rotation in general you will use geometry software. This will allow you to rotate any figure any number of degrees about any point. There are a few common rotations that are good to know how to do without geometry software, shown in the table below.

    Center of Rotation Angle of Rotation Preimage (Point \(P\)) Rotated Image (Point \(P′\))
    \((0, 0)\) \(90^{\circ}\) (or \(−270^{\circ} ) \((x,y)\) \((−y,x)\)
    \((0, 0)\) \(180^{\circ}\) (or \(−180^{\circ}\) ) \((x,y)\) \((−x,−y)\)
    \((0, 0)\) \(270^{\circ}\) (or \(−90^{\circ}\) ) \((x,y)\) \((y,−x)\)

    Let's draw the preimage and image and properly label each for the following transformation:

    Line \(\overline{AB}\) drawn from \((-4, 2)\) to \((3, 2)\) has been rotated about the origin at an angle of \(90^{\circ}\) CW.

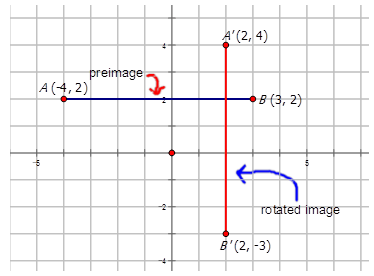
    f-d_4e8293b23f1108eb46b23b117bec739624ac7421dbdc195594564a7e+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{1}\)

    Now, let's draw and label the rotated image for the following rotations:

    1. The diamond \(ABCD\) is rotated \(145^{\circ}\) CCW about the origin to form the image \(A′B′C′D′\).
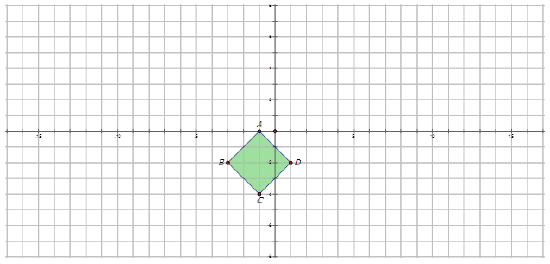
    f-d_867d3e90e4b46c1dec081c2f1d13ec0ad1ebf473ee1eb5b37f3acc87+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{2}\)
    f-d_15917e414351bed4facebc51b0fc65c528909378997202e4fdad295e+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{3}\)

    Notice the direction is counter-clockwise.

    1. The following figure is rotated about the origin \(200^{\circ}\) CW to make a rotated image.
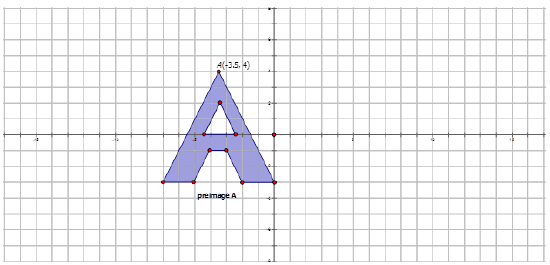
    f-d_6489c7a10df04071dccadd8d756f2c77bea669f2716b1ace4e0745a5+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{4}\)
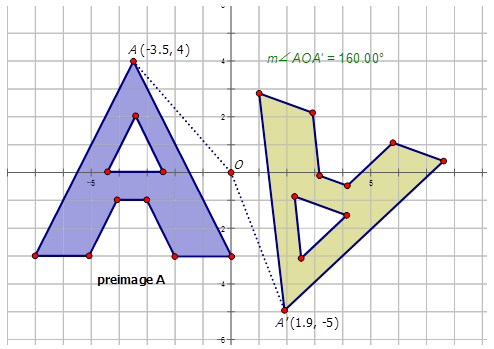
    f-d_b1eb7fcd75f30bc2b8523d839684fe764a62ffebe2d35428a7cb3af8+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{5}\)

    Notice the direction of the rotation is counter-clockwise, therefore the angle of rotation is \(160^{\circ}\).

    Example \(\PageIndex{1}\)

    Earlier, you were asked about the quadrilateral \(WXYZ\) has coordinates \(W(−5,−5)\), \(X(−2,0)\), Y(2,3)\) and \(Z(−1,3)\). Draw the quadrilateral on the Cartesian plane. Rotate the image \(110^{\circ}\) counterclockwise about the point X\). Show the resulting image.

    Solution

    f-d_3ff27bec70435e89df832cb2242ab8fab65df5fa515f1b579891d4f9+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{6}\)

    Example \(\PageIndex{2}\)

    Line \(\overline{ST}\) drawn from \((-3, 4)\) to \((-3, 8)\) has been rotated \(60^{\circ}\) CW about the point \(S\). Draw the preimage and image and properly label each.

    f-d_9a247e66473af0d0e6b8cbca69dee2ac7587b495f2623199b2d49e8c+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{7}\)

    Solution

    Notice the direction of the angle is clockwise, therefore the angle measure is \(60^{\circ}\) CW or \(−60^{\circ}\).

    Example \(\PageIndex{3}\)

    The polygon below has been rotated \(155^{\circ}\) CCW about the origin. Draw the rotated image and properly label each.

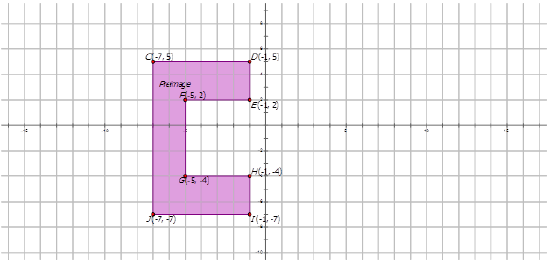
    f-d_880a2f3c197e88037d4aff3df22000108b3429f09f91023a185cfbc2+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{8}\)

    Solution

    f-d_63d6f804cf604a611bec938a0f61983a0efa7e00f1944ba3c6ecf663+IMAGE_THUMB_POSTCARD_TINY+IMAGE_THUMB_POSTCARD_TINY.png
    Figure \(\PageIndex{9}\)

    Notice the direction of the angle is counter-clockwise, therefore the angle measure is \(155^{\circ}\) CCW or \(155^{\circ}\).

    Example \(\PageIndex{4}\)

    The purple pentagon is rotated about the point A \(225^{\circ}\). Find the coordinates of the purple pentagon. On the diagram, draw and label the rotated pentagon.

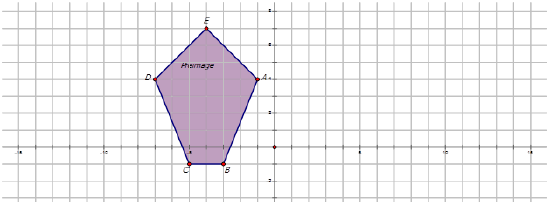
    f-d_d9f2c73ee75e6361c623ee4128c2fa68a44e0b7b743e5a2c6362a72e+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{10}\)

    Solution

    f-d_181564900f7f7aa9c2cf034dabec27754bc89bc96171f7435398dfbb+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{11}\)

    The measure of \(\angle BAB′=m\angle BAE′+m\angle E′AB′\). Therefore \(\angle BAB′=111.80^{\circ} +113.20^{\circ}\) or \(225^{\circ}\). Notice the direction of the angle is counter-clockwise, therefore the angle measure is \(225^{\circ}\) CCW or \(225^{\circ}\).

    Review

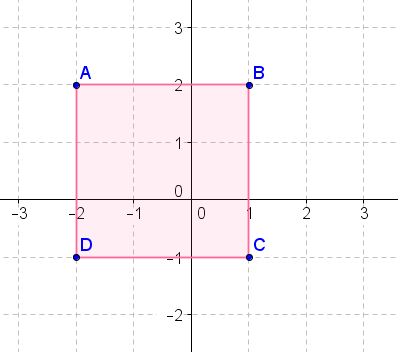
    f-d_234afdf1317423c6f313c6e1127ac511da750b753685f77fa1caf1c1+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{12}\)
    1. Rotate the above figure \(90^{\circ}\) clockwise about the origin.
    2. Rotate the above figure \(270^{\circ}\) clockwise about the origin.
    3. Rotate the above figure \(180^{\circ}\) about the origin.
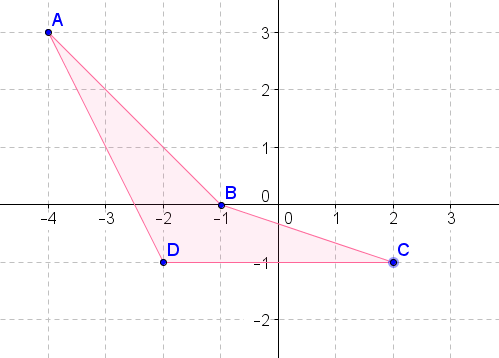
    f-d_4f6233f7ca960b07c4aa34d7be4bc94232c3bb7afad60c012c860d61+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{13}\)
    1. Rotate the above figure \(90^{\circ}\) counterclockwise about the origin.
    2. Rotate the above figure \(270^{\circ}\) counterclockwise about the origin.
    3. Rotate the above figure \(180^{\circ}\) about the origin.
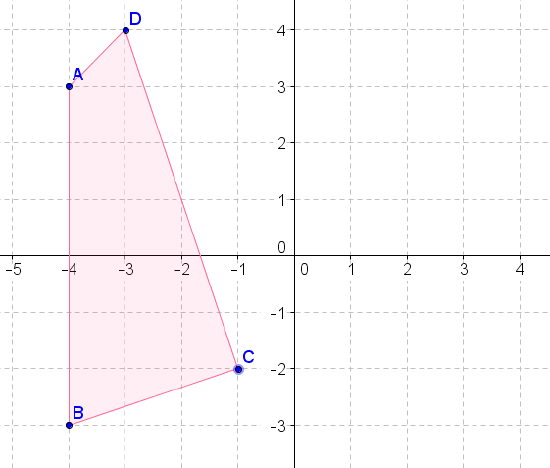
    f-d_00db51f117c77256de686a27748cdfa88c7f0ed0e633a59a3f89da32+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{14}\)
    1. Rotate the above figure \(90^{\circ}\) clockwise about the origin.
    2. Rotate the above figure \(270^{\circ}\) clockwise about the origin.
    3. Rotate the above figure \(180^{\circ}\) about the origin.
    f-d_7a0999108d4a8ddde2d12e9dbc80c751d5d6fdcec076d52a0c96c0dc+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{15}\)
    1. Rotate the above figure \(90^{\circ}\) counterclockwise about the origin.
    2. Rotate the above figure \(270^{\circ}\) counterclockwise about the origin.
    3. Rotate the above figure \(180^{\circ}\) about the origin.
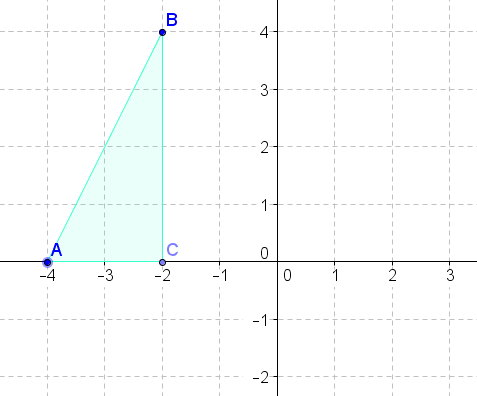
    f-d_a0bd1ee4fe9000d0b093449e2ca6308318278563f9c95bda50d72a99+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{16}\)
    1. Rotate the above figure \(90^{\circ}\) clockwise about the origin.
    2. Rotate the above figure \(270^{\circ}\) clockwise about the origin.
    3. Rotate the above figure \(180^{\circ}\) about the origin.
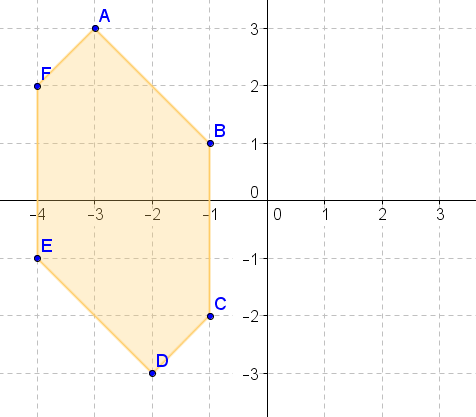
    f-d_ca485b559bb107f944db21c19cb24bdd30b0eee9ee1f9fadc2416114+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{17}\)
    1. Rotate the above figure \(90^{\circ}\) counterclockwise about the origin.
    2. Rotate the above figure \(270^{\circ}\) counterclockwise about the origin.
    3. Rotate the above figure \(180^{\circ}\) about the origin.
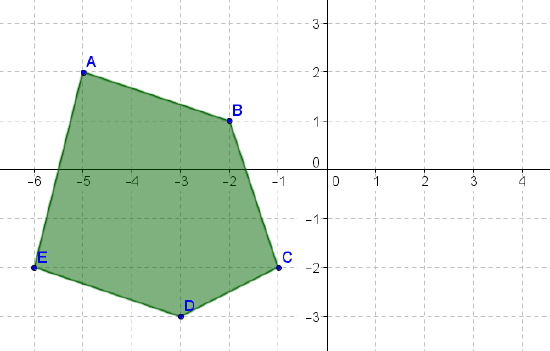
    f-d_ab7c6c52e913f82cdc35f16bafc69b8c2b49fbcbc9082c37fd4469b2+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{18}\)
    1. Rotate the above figure \(90^{\circ}\) clockwise about the origin.
    2. Rotate the above figure \(270^{\circ}\) clockwise about the origin.
    3. Rotate the above figure \(180^{\circ}\) about the origin.
    f-d_b9dcf9e5f525629af5fa89480a949f75b148f600e1b7a4405d326c4e+IMAGE_TINY+IMAGE_TINY.png
    Figure \(\PageIndex{19}\)
    1. Rotate the above figure \(90^{\circ}\) counterclockwise about the origin.
    2. Rotate the above figure \(270^{\circ}\) counterclockwise about the origin.
    3. Rotate the above figure \(180^{\circ}\) about the origin.

    Review (Answers)

    To see the Review answers, open this PDF file and look for section 10.8.

    Vocabulary

    Term Definition
    Rotation A rotation is a transformation that turns a figure on the coordinate plane a certain number of degrees about a given point without changing the shape or size of the figure.

    Additional Resources

    Practice: Geometry Software and Graphing Rotations

    This page titled 8.12: Geometry Software and Graphing Rotations is shared under a CK-12 license and was authored, remixed, and/or curated by CK-12 Foundation via source content that was edited to the style and standards of the LibreTexts platform; a detailed edit history is available upon request.

    CK-12 Foundation
    LICENSED UNDER
    CK-12 Foundation is licensed under CK-12 Curriculum Materials License
    1. Back to top
    • Was this article helpful?

    Recommended articles

    1. Article type
      Section or Page
      Author
      CK12
      License
      CK-12
      OER program or Publisher
      CK-12
      Show TOC
      no
    2. Tags
      1. source@https://www.ck12.org/c/geometry