8.18: Notation for Composite Transformations
( \newcommand{\kernel}{\mathrm{null}\,}\)
Interpret and use notation for combined transformations
The figure below shows a composite transformation of a trapezoid. Write the mapping rule for the composite transformation.

In geometry, a transformation is an operation that moves, flips, or changes a shape to create a new shape. A composite transformation is when two or more transformations are performed on a figure (called the preimage) to produce a new figure (called the image). The order of transformations performed in a composite transformation matters.
To describe a composite transformation using notation, state each of the transformations that make up the composite transformation and link them with the symbol ∘. The transformations are performed in order from right to left. Recall the following notation for translations, reflections, and rotations:
- Translation: Ta,b:(x,y)→(x+a,y+b) is a translation of a units to the right and b units up.
- Reflection: ry−axis(x,y)→(−x,y).
- Rotation: R90∘(x,y)=(−y,x)
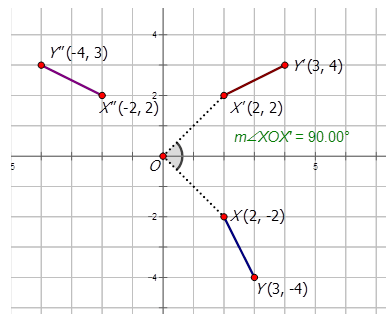
Let's graph the line described below and the composite image defined by ry−axis∘R90∘:
The first translation is a 90∘ CCW turn about the origin to produce X′Y′. The second translation is a reflection about the y-axis to produce X′′Y′′.
Now, let's graph the composite images described in the following problems:
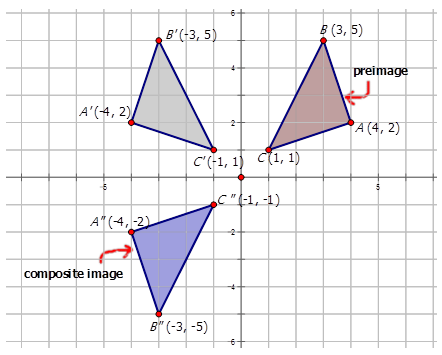
- Image A with vertices A(3,5), B(4,2) and C(1,1) undergoes a composite transformation with mapping rule rx−axis∘ry−axis.
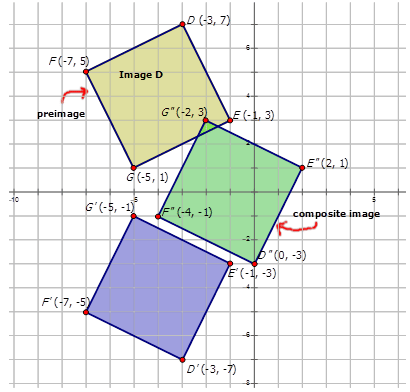
- Image D with vertices D(−3,7), E(−1,3), F(−7,5) and G(−5,1) undergoes a composite transformation with mapping rule T3,4∘rx−axis.
Example 8.18.1
Earlier, you were asked to write the mapping rule for the following composite transformation:

Solution
The transformation from Image A to Image B is a reflection across the y-axis. The notation for this is ry−axis. The transformation for image B to form image C is a rotation about the origin of 90∘CW. The notation for this transformation is R270∘. Therefore, the notation to describe the transformation of Image A to Image C is R270∘∘ry−axis
Example 8.18.2
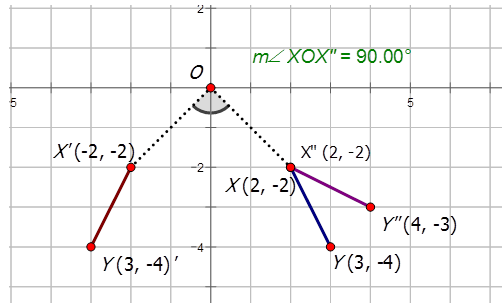
Graph the line XY given that X(2,−2) and Y(3,−4). Also graph the composite image that satisfies the rule R90∘∘ry−axis
Solution
The first transformation is a reflection about the y-axis to produce X′Y′. The second transformation is a 90∘CCW turn about the origin to produce X′′Y′′.
Example 8.18.3
Describe the composite transformations in the diagram below and write the notation to represent the transformation of figure ABCD to A′′B′′C′′D′′.

Solution
There are two transformations shown in the diagram. The first transformation is a reflection about the line X=2\) to produce A′B′C′D′. The second transformation is a 90∘CW (or 270∘CCW) rotation about the point (2,0) to produce the figure A′′B′′C′′D′′. Notation for this composite transformation is:
R270∘∘rx=2
Example 8.18.4
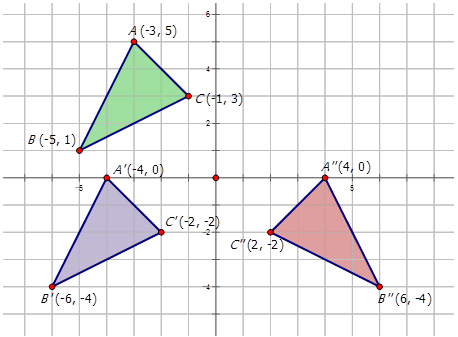
Describe the composite transformations in the diagram below and write the notation to represent the transformation of figure ABC to A′′B′′C′′.
Solution
There are two transformations shown in the diagram. The first transformation is a translation of 1 unit to the left and 5 units down to produce A′B′C′. The second reflection in the y-axis to produce the figure A′′B′′C′′. Notation for this composite transformation is:
ry−axis∘T−1,−5
Review
Complete the following table:
| Starting Point | T3,−4∘R90∘ | rx−axis∘ry−axis | T1,6∘rx−axis | ry−axis∘R180∘ |
|---|---|---|---|---|
| 1. (1,4) | ||||
| 2. (4,2) | ||||
| 3. (2,0) | ||||
| 4. (−1,2) | ||||
| 5. (−2,−3) | ||||
| 6. (4,−1) | ||||
| 7. (3,−2) | ||||
| 8. (5,4) | ||||
| 9. (−3,7) | ||||
| 10. (0,0) |
Write the notation that represents the composite transformation of the preimage A to the composite images in the diagrams below.
-
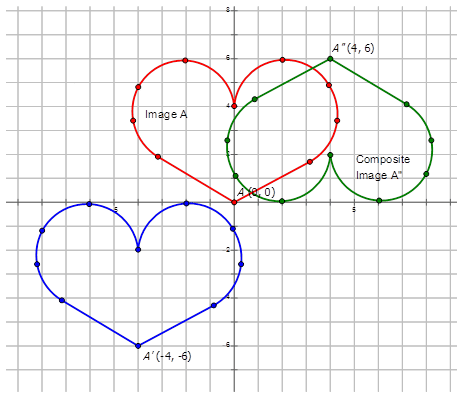
Figure 8.18.9 -
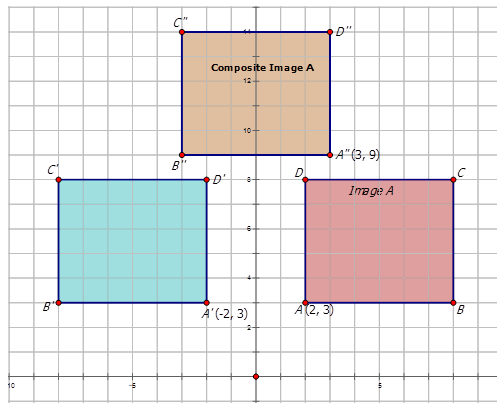
Figure 8.18.10 -

Figure 8.18.11 -
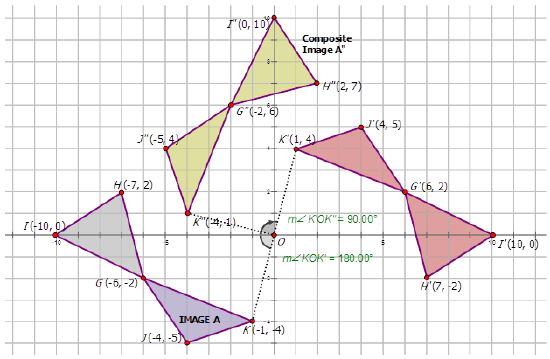
Figure 8.18.12 -
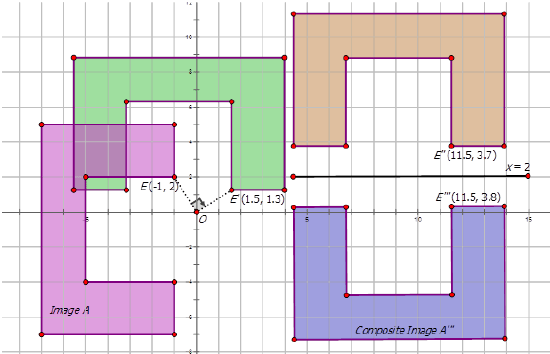
Figure 8.18.13
Review (Answers)
To see the Review answers, open this PDF file and look for section 10.15.
Vocabulary
| Term | Definition |
|---|---|
| Reflections | Reflections are transformations that result in a "mirror image" of a parent function. They are caused by differing signs between parent and child functions. |
| Rotation | A rotation is a transformation that turns a figure on the coordinate plane a certain number of degrees about a given point without changing the shape or size of the figure. |
| Transformation | A transformation moves a figure in some way on the coordinate plane. |
Additional Resources
Practice: Notation for Composite Transformations

