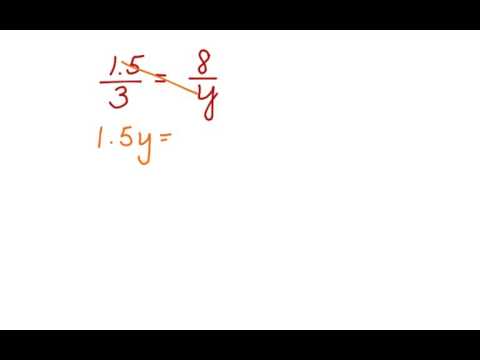9.8: Area and Volume of Similar Solids
- Page ID
- 6194
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Solve problems using ratios between similar solids.
Understand Scale Relationships
Tim has a cube with a side length of 4 inches. He has a similar cube with dimensions that are twice the first cube. How does the volume of the larger cube compare to the volume of the smaller cube?
In this concept, you will learn to understand scale relationships of area and volume.
Scale Relationships
You can compare the scale relationships of distance, area and volume when looking at three–dimensional figures. Some examples of three–dimensional figures include a prism or a pyramid. When you compare different measurements, you will see the proportional relationships between them.
Let’s look at a situation involving volume.
Brooke has a scale model of a warehouse. A storage unit is shaped like a rectangular prism and has the dimensions 4 in. by 3 in. by 6 in. If the scale of the model is \(0.5 \text{ in}=2\text{ ft}\), what are the actual dimensions of the storage unit? What is the volume?
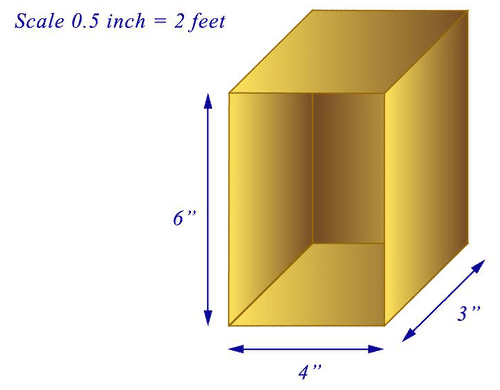
First, notice that there are two parts to this problem. The first part is figuring out the actual dimensions given that Brooke has a scale model. The second part is figuring out the volume. Start by using the scale to write a proportion for the length, width, and height.
\(\begin{aligned}
&\text { length } \quad \text { width } \quad \text { height }\\
&\frac{0.5 \mathrm{in}}{2 \mathrm{ft}}=\frac{4 \mathrm{in}}{x \mathrm{ft}} \quad \frac{0.5 \mathrm{in}}{2 \mathrm{ft}}=\frac{3 \mathrm{in}}{x \mathrm{ft}} \quad \frac{0.5 \mathrm{in}}{2 \mathrm{ft}}=\frac{6 \mathrm{in}}{x \mathrm{ft}}
\end{aligned}\)
Next, cross multiply for each dimension.
\(\begin{array}{ccc}
\text { length } & \text { width } & \text { height } \\
\frac{0.5}{2}=\frac{4}{x} & \frac{0.5}{2}=\frac{3}{x} & \frac{0.5}{2}=\frac{6}{x} \\
0.5 x=2 \times 4 & 0.5 x=2 \times 3 & 0.5 x=2 \times 6 \\
0.5 x=8 & 0.5 x=6 & 0.5 x=12
\end{array}\)
Then, divide both sides by 0.5 to solve for x.
\(\begin{array}{ccc}
\text { length } & \text { width } & \text { height } \\
0.5 x=8 & 0.5 x=6 & 0.5 x=12 \\
\frac{0.5 x}{0.5}=\frac{8}{0.5} & \frac{0.5 x}{0.5}=\frac{6}{0.5} & \frac{0.5 x}{0.5}=\frac{12}{0.5} \\
x=16 & x=12 & x=24
\end{array}\)
The answers are 16, 12, and 24.
The length of the storage unit is 16 ft, the width is 12 ft, and the height is 24 ft.
Then, you need to calculate the volume of the storage unit.
\(\begin{aligned} V&=l\times w\times h \\ V&=16\times 12\times 24 \\ V&=4608\end{aligned}\)
The answer is 4608.
The volume of the storage unit is \(4608\: ft^{3}\) or 4608 cubic feet.
There is a relationship between the area of the base of the prism and the volume of the prism. Let’s take a look at how the area of the base of the prism relates to the volume of the prism using the storage unit problem.
\(\begin{aligned} A&=l\times w \\ A&=16\times 12 \\ A&=192\end{aligned}\)
The area of the prism is 192 ft^{2} or 192 square feet.
Now, to see the relationship between the volume of a prism and the area of a prism, divide the volume by the area.
\(\dfrac{V}{A}=4608192\)
\(\dfrac{V}{A}=24\)
The answer is 24.
Notice that this is the height of the prism.
Example \(\PageIndex{1}\)
Earlier, you were given a problem about Tim’s cubes.
Tim has two cubes where the larger one is twice the size of the smaller one. This means that there is a scale factor of 2.
Solution
First, find the dimensions of the larger cube.
The smaller cube has a side measure of 4 inches. Since this a cube, the length=width = height =4 inches.
The larger cube will have a side measure of 4 in\times 2=8 in. Therefore the length=width = height =8 inches.
Next find the volume of both cubes and compare.
\(\begin{aligned}
&\text { Volume of smaller cube } \quad \text { Volume of larger cube }\\
&\begin{array}{ll}
V=l \times w \times h & V=l \times w \times h \\
V=4 \mathrm{in} \times 4 \mathrm{in} \times 4 \mathrm{in} & V=8 \mathrm{in} \times 8 \mathrm{in} \times 8 \mathrm{in} \\
V=64 \mathrm{in}^{3} & V=512 \mathrm{in}^{3}
\end{array}
\end{aligned}\)
Then, compare the volume of the larger cube to the smaller cube.
\(\begin{aligned} \dfrac{\text{Volume of larger cube}}{\text{Volume of smaller cube}}&=\dfrac{512}{64} \\ \dfrac{\text{Volume of larger cube}}{\text{Volume of smaller cube}}&=8\end{aligned}\)
The answer is 8.
The volume of the larger cube is 8 times the volume of the smaller cube.
Example \(\PageIndex{2}\)
Prove that the height of the following prism can be found by using a ratio of volume to area for a prism with a length of 6 inches, a width of 5 inches and a height of 9 inches.
Solution
First, calculate the volume of the prism.
\(\begin{aligned} V&=l\times w\times h \\ V&=6\times 5\times 9 \\ V&=270\end{aligned}\)
Next, calculate the area of the prism.
\(\begin{aligned} A&=l\times w \\ A&=6\times 5 \\ A&=30 \end{aligned}\)
Then, divide the volume by the area.
\(\begin{aligned}\dfrac{V}{A}&=\dfrac{270}{30} \\ \dfrac{V}{A}&=9\end{aligned}\)
The answer is 9.
Notice that this is the height of the prism.
Example \(\PageIndex{3}\)
A prism has a length of 16 feet, a width of 12 feet, and a height of 18 feet. Find the volume of the prism.
Solution
\(\begin{aligned} V&=l\times w\times h \\ V&=16\times 12\times 18 \\ V&=3456\end{aligned}\)
The answer is 3456.
The volume of the prism is \(3456 ft^{3}\) or 3456 cubic feet.
Example \(\PageIndex{4}\)
A prism has a length of 16 feet, a width of 12 feet, and a height of 18 feet. Find the area of the base.
Solution
\(\begin{aligned} A&=l\times w \\ A&=16\times 12 \\ A&=192\end{aligned}\)
The answer is 192.
The area of the prism is \(192 ft^{2}\) or 192 square feet.
Example \(\PageIndex{5}\)
Write a ratio comparing the volume to the area of the prism and simplify.
Solution
\(\beign{aligned}\dfrac{V}{A}&=\dfrac{3456}{192} \\ \dfrac{V}{A}&=18\end{aligned}\)
The answer is 18.
The height of the prism is 18 feet.
Review
Solve each problem.
1. A cube measures 8 feet on each side. A similar cube has dimensions that are twice as large. How does the volume of the larger cube compare to the volume of the smaller cube? Write a ratio to show the comparison.
2. A cube measures 3 inches on each side. A similar cube has dimensions that are half that of the other cube. How does the volume of the larger cube compare to the volume of the smaller cube? Write a ratio to show the comparison
3. A scale model of a sandbox has dimensions 0.5 inch by 3 inches by 4 inches. If the scale of the model is 1 inch=1 foot, what is the volume of the actual sandbox?
4. A cube measures 5 inches on each side. A similar cube has dimensions that are 3 times as large. How does the volume of the larger cube compare to the volume of the smaller cube? Write a ratio to show the comparison.
5. A shipping box measures 16 inches by 12 inches by 8 inches. A second box has a similar size but each dimension is 14 as long. How does the volume of the second box compare to the volume of the first box?
6. Rina’s fish tank has a volume of 8,000 cubic inches. The dimensions of AVA’s fish tank are all 12 the size of Rina’s. What is the volume of AVA’s fish tank?
7. A prism has a width of 6 feet, a length of 8 feet and a height of 12 feet. What is the volume of the prism?
8. What is the area of the base of this prism?
9. What would the volume be of a prism 14 the size of the one described above?
10. What would the volume be of a prism 12 the size of the one described above?
11. What would the volume be of a prism twice the size of the one described above?
12. What ratio can you use to discover the relationship between volume and area?
13. Which measurement will you find when you simplify this ratio?
14. True or false. You can use scale measurement to find the height of a prism.
15. True or false. You can use scale measurement to find the dimensions of a prism.
Resources
Review (Answers)
To see the Review answers, open this PDF file and look for section 4.11.
Vocabulary
| Term | Definition |
|---|---|
| Area | Area is the space within the perimeter of a two-dimensional figure. |
| Scale Model | A scale model is a model that represents a three-dimensional space. |
| Three – Dimensional | A figure drawn in three dimensions is drawn using length, width and height or depth. |
| Two – Dimensional | A figure drawn in two dimensions is only drawn using length and width. |
| Volume | Volume is the amount of space inside the bounds of a three-dimensional object. |
Video: Similar Solids Principles - Basic
Activities: Area and Volume of Similar Solids Discussion Questions
Study Aids: Surface Area and Volume Study Guide
Practice: Area and Volume of Similar Solids
Real world: Is There Life Out There?