Skip to main content
K12 LibreTexts

9.26: Surface Area of Spheres

  1. Last updated
  2. Save as PDF
  • Page ID
    6749

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

    \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

    \( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

    ( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

    \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

    \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

    \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

    \( \newcommand{\Span}{\mathrm{span}}\)

    \( \newcommand{\id}{\mathrm{id}}\)

    \( \newcommand{\Span}{\mathrm{span}}\)

    \( \newcommand{\kernel}{\mathrm{null}\,}\)

    \( \newcommand{\range}{\mathrm{range}\,}\)

    \( \newcommand{\RealPart}{\mathrm{Re}}\)

    \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

    \( \newcommand{\Argument}{\mathrm{Arg}}\)

    \( \newcommand{\norm}[1]{\| #1 \|}\)

    \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

    \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    \( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

    \( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

    \( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

    \( \newcommand{\vectorC}[1]{\textbf{#1}} \)

    \( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

    \( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

    \( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

    \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

    \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

    \(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)

    Surface area from radius

    Surface Area of Spheres

    f-d_2e299ae8db9c9825170a81cf9b9673fdb61b0a607d8ba8eb1fbcc6d0+IMAGE_THUMB_POSTCARD_TINY+IMAGE_THUMB_POSTCARD_TINY.png
    Figure \(\PageIndex{1}\)

    Erin is decorating a sphere shaped piñata with pink construction paper. Each sphere has a radius of 2.4 feet and Erin made four piñatas. How much construction paper will she need to cover each piñata completely? How much will she need in total?

    In this concept, you will learn to calculate the surface area of spheres.

    Surface Area

    A sphere is a solid figure that exists in three-dimensional space. Spheres consist of all the points that are equidistant from a center point. Every point on the sphere is the distance of the radius from the center. Spheres are perfectly round.

    f-d_5a1d598b7ee42f177604d03df7d19c30082b1d7518cbbdacf6f47434+IMAGE_THUMB_POSTCARD_TINY+IMAGE_THUMB_POSTCARD_TINY.png
    Figure \(\PageIndex{2}\)

    Spheres do not have any faces because they are round. Still, you can think of its surface as a flat plane that you can unroll. Imagine you could wrap a sphere in wrapping paper, like a present. The amount of wrapping paper needed to cover the figure exactly represents its surface area.

    If you could “unroll” the sphere and show it as a rectangle, the rectangle would have a width that is equivalent to the diameter of the sphere. Its length would be the same as the circumference of the sphere (circumference is the distance around a circle). Now this gives you something you can work with, because you can use the area formula for rectangles to find the area of the “unrolled” sphere.

    The formula for the area of rectangles is: \(A=lw\).

    The length is the circumference, so the formula becomes:

    \(\begin{aligned} l&=C \\ C&=2\pi r \\ \therefore A&=2\pi r\times w\end{aligned} \)

    Now, the width is equal to the diameter of the “roll”. The diameter is twice the radius. Now your formula becomes:

    \(\begin{aligned} w&=d \\ d&=2r \\ \therefore A&=2\pi r \times 2r \\ A&=4\pi r^{2}\end{aligned}\)

    So, the formula you use for finding the surface area of a sphere is \(SA=4\pi r^{2}\).

    All you need to do is substitute the measure of the radius for r in the formula and solve for \(SA\), the surface area.

    Let’s look at an example.

    What is the surface area of the sphere below?

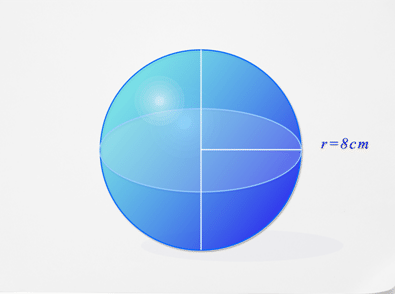
    f-d_eb8631fbbdc25bde200ab9c2de0f73da82a4fff5d2ab8e6c3bf9915d+IMAGE_THUMB_POSTCARD_TINY+IMAGE_THUMB_POSTCARD_TINY.png
    Figure \(\PageIndex{3}\)

    First, substitute what you know into the surface area formula.

    \(\begin{aligned} SA&=4\pi r^{2} \\ SA&=4\pi (8)^{2}\end{aligned}\)

    Next, use algebra to calculate the surface area.

    \(\begin{aligned} SA&=4\pi (8)^{2} \\ SA&=4\pi (64) \\ SA&=256\pi \\ SA&=804.25\end{aligned} \)

    The answer is 804.25.

    The surface area of the sphere is \(804.25 \text{ cm}^{2}\).

    If you wanted to make your measurement more accurate, you could say that the surface area is \(256\pi \text{ cm}^{2}\).

    Example \(\PageIndex{1}\)

    Earlier, you were given a problem about Erin and her piñatas.

    Solution

    Erin wants to know how much pink construction paper she needs to decorate a sphere shaped piñata with a radius of 2.4 feet. She also wants to know the total amount to decorate four piñatas.

    First, substitute what you know into the surface area formula.

    \(\begin{aligned}SA&=4\pi r^{2} \\ SA&=4\pi (2.4)^{2}\end{aligned}\)

    Next, use algebra to calculate the surface area.

    \(\begin{aligned}SA&=4\pi (2.4)^{2} \\ SA&=4\pi (5.76) \\ SA&=3.04\pi \\ SA&=72.38\end{aligned}\)

    Then multiply by 4 to find the total amount of construction paper.

    \(\begin{aligned}SA_{Total}&=4\times 72.38 \\ SA_{Total}&=289.52\end{aligned}\)

    The answer is 289.52.

    Erin would need \(72.38 \text{ ft}^{2}\) of pink construction paper for one piñata and \(289.52 \text{ ft}^{2}\) for all four.

    Example \(\PageIndex{2}\)

    The sphere below has a radius of 8.5 inches. What is the surface area of the sphere?

    f-d_b5b033b1cb5d9ed1927c2b867f179210dde04b518f7020fa87b0efee+IMAGE_THUMB_POSTCARD_TINY+IMAGE_THUMB_POSTCARD_TINY.png
    Figure \(\PageIndex{4}\)

    Solution

    First, substitute what you know into the surface area formula.

    \(\begin{aligned}SA&=4\pi r^{2} \\ SA&=4\pi (8.5)^{2}\end{aligned}\)

    Next, use algebra to calculate the surface area.

    \(\begin{aligned}SA&=4\pi (8.5)^{2} \\ SA&=4\pi (72.25) \\ SA&=89\pi \\ SA&=907.9\end{aligned} \)

    The answer is 907.9.

    The surface area of the sphere is \(907.9 \text{ in}^{2}\).

    If you wanted to make your measurement more accurate, you could say that the surface area is \(289 \pi \text{ in}^{2}\).

    Example \(\PageIndex{3}\)

    Find the surface area of a sphere with a radius of 5 inches.

    Solution

    First, substitute what you know into the surface area formula.

    \(\begin{aligned}SA&=4\pi r^{2} \\ SA&=4\pi (5)^{2}\end{aligned}\)

    Next, use algebra to calculate the surface area.

    \(\begin{aligned}SA&=4\pi (5)^{2} \\ SA&=4\pi (25) \\ SA&=100\pi \\SA&=314.16\end{aligned}\)

    The answer is 314.16.

    The surface area of the sphere is \(314.16 \text{ in}^{2}\) or \(100 \pi \text{ in}^{2}\).

    Example \(\PageIndex{4}\)

    Find the surface area of a sphere with a radius of 8 meters.

    Solution

    First, substitute what you know into the surface area formula.

    \(\begin{aligned}SA&=4\pi r^{2} \\ SA&=4\pi (8)^{2}\end{aligned}\)

    Next, use algebra to calculate the surface area.

    \(\begin{aligned}SA&=4\pi (8)^{2} \\ SA&=4\pi (64) \\ SA&=256\pi \\ SA&=804.25\end{aligned}\)

    The answer is 804.25.

    The surface area of the sphere is \(804.25 \text{ m}^{2}\) or \(256\pi \text{ m}^{2}\).

    Example \(\PageIndex{5}\)

    Find the surface area of a sphere with a radius of 12 feet.

    Solution

    First, substitute what you know into the surface area formula.

    \(\begin{aligned}SA&=4\pi r^{2} \\ SA&=4\pi (12)^{2}\end{aligned}\)

    Next, use algebra to calculate the surface area.

    \(\begin{aligned}SA&=4\pi (12)^{2} \\ SA&=4\pi (144) \\ SA&=576\pi \\ SA&=1809.6\end{aligned}\)

    The answer is 1809.6.

    The surface area of the sphere is \(1809.6 \text{ ft}^{2}\) or \(576\pi \text{ ft}^{2}\).

    Review

    Find the surface area of each sphere. Use 3.14 to approximate pi.

    1. A sphere with a radius of 4 in.
    2. A sphere with a radius of 2 in.
    3. A sphere with a radius of 3.5 ft.
    4. A sphere with a radius of 6.7 in.
    5. A sphere with a radius of 12 cm.
    6. A sphere with a radius of 1.6 ft.
    7. A sphere with a radius of 9 m.
    8. A sphere with a diameter of 9 m.
    9. A sphere with a diameter of 18 in.
    10. A sphere with a diameter of 10 cm.
    11. A sphere with a diameter of 12 m.
    12. A sphere with a diameter of 13 ft.
    13. A sphere with a diameter of 15 m.
    14. What is the surface area of a sphere whose diameter is 22 centimeters?
    15. Bruce is making a sculpture in his art class that is made of 3 spheres. Each sphere has a radius of 2.3 feet. He will paint them all with blue poster paint. If each bottle of paint covers 20 square feet, how many bottles will Bruce need to buy?

    Review (Answers)

    To see the Review answers, open this PDF file and look for section 8.14.

    Resources

    Vocabulary

    Term Definition
    Surface Area Surface area is the total area of all of the surfaces of a three-dimensional object.

    Additional Resources

    Interactive Element

    Practice: Surface Area of Spheres

    This page titled 9.26: Surface Area of Spheres is shared under a CK-12 license and was authored, remixed, and/or curated by CK-12 Foundation via source content that was edited to the style and standards of the LibreTexts platform.

    CK-12 Foundation
    LICENSED UNDER
    CK-12 Foundation is licensed under CK-12 Curriculum Materials License
    1. Back to top
    • Was this article helpful?

    Recommended articles

    1. Author
      CK12
      License
      CK-12
      OER program or Publisher
      CK-12
      Show TOC
      no
    2. Tags
      1. source@https://www.ck12.org/c/geometry