4.8: Unknown Dimensions of Triangles
- Page ID
- 4804
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)
Marie has a collection of photos of buildings shaped like pyramids. As she looked through the photos, she wondered how tall one of the buildings was. She knows that the area of the triangle shaped surface is 900 square feet. She also knows that the length of each side of the base of the pyramid (which is also the length of the base of each triangular side) is 60 feet. Given the base and area of the triangles forming the pyramid, how can Marie determine the height of the pyramid?
In this concept, you will learn how to find the unknown dimensions of triangles given the area and another dimension.
Finding Unknown Dimensions of Triangles
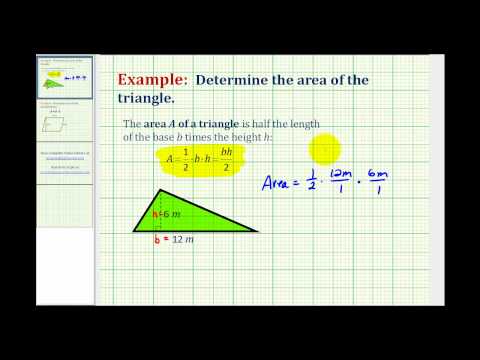
There may be a situation when you will be given the area of a triangle and one other dimension and asked to find the missing dimension. When this happens, you can figure out the missing dimension by using the formula for the area of a triangle.
\(A=\dfrac{1}{2}bh\)
If you have been given the area and the base of a triangle, then you can use the formula to figure out the height. If you have been given the area and the height then you can figure out the base.
Now let’s use the formula to find the base of a triangle when the area and height are given.
A triangle has an area of 36 square inches and a height of 6 inches. What is the measure of the base?
Start by looking at the formula for finding the area of a triangle.
\(A=\dfrac{1}{2}bh\)
First, substitute the given information into the formula.
\(36=\dfrac{1}{2}(6)b\)
Next, multiply one-half by six.
\(36=3b\)
Then, solve for the base by dividing both sides by 3.
\(\begin{align*} \dfrac{36}{3} &=\dfrac{3b}{3} \\ 12&=b \end{align*}\)
The answer is 12. The base of this triangle is 12 inches.
Now, let’s find the height of a triangle with a given area and base.
A triangle has an area of 64 square inches and a base of 8 inches. What is the measure of the base?
To figure this out, start by looking at the formula for finding the area of a triangle.
\(A=\dfrac{1}{2}bh\)
First, substitute the given information into the formula.
\(64=\dfrac{1}{2}(8)h\)
Next, multiply one-half by 8.
\(64=4h\)
Then, solve for the base by dividing both sides by 4.
\(\begin{align*} \dfrac{64}{4} &=\dfrac{4h}{4} \\ 16&=h \end{align*}\)
The answer is 16. The height of this triangle is 16 inches.
Example \(\PageIndex{1}\)
Earlier, you were given a problem about Marie and her photos of pyramid buildings.
Marie found out the area of each triangle shaped surface is 900 square feet. She also knows that the length of each side of the base of the pyramid (which is also the length of the base of each triangular side) is 60 feet. Marie found the formula for computing the area of a triangle, and decided to use it to figure out the triangle’s height.
\(A=\dfrac{1}{2}bh\)
Solution
First, she should substitute the given information into the formula.
\(900=\dfrac{1}{2}(60)h\)
Next, multiply one-half by 60.
\(900=30h\)
Then, solve for the base by dividing both sides by 30.
\(\begin{align*} \dfrac{900}{30}&=\dfrac{30h}{30} \\ 30 &=h\end{align*}\)
The answer is 30. The height of this pyramid is 30 feet.
Example \(\PageIndex{2}\)
Find the missing dimension of the following triangle.
The area of the triangle is 48 square feet. The base of the triangle is 12 feet. What is the height?
Solution
Start by looking at the formula for finding the area of a triangle.
\(A=\dfrac{1}{2}bh\)
First, fill in the given information.
\(48=\dfrac{1}{2}(12)h\)
Next, multiply one-half by 12.
\(48=6h\)
Then, solve for the base by dividing 48 by 6.
\(\begin{align*} \dfrac{48}{6}&=\dfrac{6h}{6} \\ 8 &=h\end{align*}\)
The answer is 8. The height of this triangle is 8 feet.
Example \(\PageIndex{3}\)
A triangle has an area of 42 sq. ft. If the base is 12 feet, what is the measure of the height?
Solution
To figure this out, start by looking at the formula for finding the area of a triangle.
\(A=\dfrac{1}{2}bh\)
First, substitute the given information into the formula.
\(42=\dfrac{1}{2} (12)h\)
Next, multiply one-half by 12.
\(42=6h\)
Then, solve for the base by dividing both sides by 6.
\(\begin{align*} \dfrac{42}{6} &=6h \\ 7&=6h\end{align*}\)
The answer is 7. The height of this triangle is 7 feet.
Example \(\PageIndex{4}\)
A triangle has an area of 16 sq. cm. If the height of the triangle is 4 cm, what is the measure of the base?
Solution
To figure this out, start by looking at the formula for finding the area of a triangle.
\(A=\dfrac{1}{2}bh\)
First, substitute the given information into the formula.
\(16=\dfrac{1}{2}b(4)\)
Next, multiply one-half by 4.
\(16=2b\)
Then, solve for the base by dividing both sides by 2.
\(\begin{align*} \dfrac{16}{2} &=\dfrac{2b}{2} \\ 8&=b \end{align*}\)
The answer is 8. The height of this triangle is 8 cm.
Example \(\PageIndex{5}\)
A triangle has an area of 40 sq. meters and a base of 10. What is the height?
Solution
Start by looking at the formula for finding the area of a triangle.
\(A=\dfrac{1}{2}bh\)
First, substitute the given information into the formula.
\(40=\dfrac{1}{2}(10)h\)
Next, multiply one-half by 10.
\(40=5h\)
Then, solve for the base by dividing both sides by 5.
\(\dfrac{40}{5}=\dfrac{5h}{5}\)
\(8=h\)
The answer is 8. The height of this triangle is 8 meters.
Review
Find the missing dimension given the area and either the base or height of a triangle.
- Area = 15 sq. in, Base = 10 in, what is the height?
- Area = 40 sq. in, Base = 20 in, what is the height?
- Area = 24 sq. ft, Base = 8 ft, what is the height?
- Area = 16 sq. m, Base = 8 m, what is the height?
- Area = 25 sq. in, height = 5 in, what is the base?
- Area = 36 sq. ft, Height = 6 ft, what is the base?
- Area = 54 sq. cm, Height = 9 cm, what is the base?
- Area = 80 sq. meters, Base = 16 meters, what is the height?
- Area = 75 sq. meters, Base = 10 meters, what is the height?
- Area = 90 sq. meters, Base = 30 meters, what is the height?
- Area = 180 sq. meters, Base = 10 meters, what is the height?
- Area = 90 sq. meters, Base = 15 meters, what is the height?
- Area = 120 sq. meters, Base = 60 meters, what is the height?
- Area = 150 sq. meters, Base = 50 meters, what is the height?
- Area = 280 sq. meters, Base = 140 meters, what is the height?
Review (Answers)
To see the Review answers, open this PDF file and look for section 10.4.
Resources
Vocabulary
| Term | Definition |
|---|---|
| Parallelogram | A parallelogram is a quadrilateral with two pairs of parallel sides. |
| Rectangle | A rectangle is a quadrilateral with four right angles. |
| Square | A square is a polygon with four congruent sides and four right angles. |
Additional Resources
Interactive Element
Video: Area of a Triangle (Whole Numbers)
Practice: Unknown Dimensions of Triangles