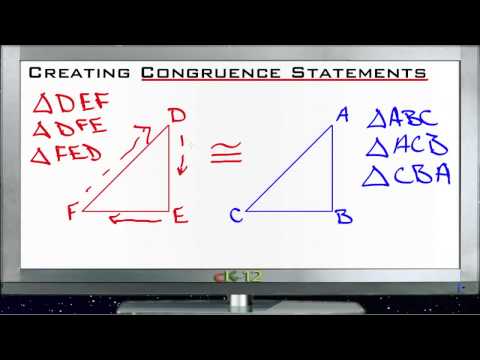
4.10: Congruence Statements
( \newcommand{\kernel}{\mathrm{null}\,}\)
Corresponding angles and sides of congruent triangles are congruent.
When stating that two triangles are congruent, the corresponding parts must be written in the same order. For example, if we know that ΔABC and ΔLMN are congruent then we know that:
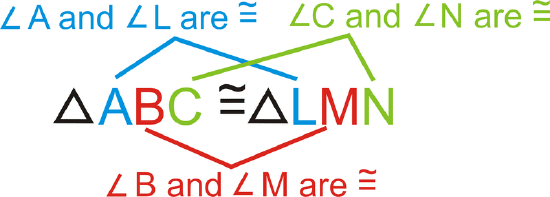
Notice that the congruent sides also line up within the congruence statement.
¯AB≅¯LM, ¯BC≅¯MN, ¯AC≅¯LN
We can also write this congruence statement five other ways, as long as the congruent angles match up. For example, we can also write ΔABC≅ΔLMN as:
ΔACB≅ΔLNMΔBCA≅ΔMNLΔBAC≅ΔMLNΔCBA≅ΔNMLΔCAB≅ΔNLM
What if you were told that ΔFGH≅ΔXYZ? How could you determine which side in ΔXYZ is congruent to ¯GH and which angle is congruent to ∠F?
Example 4.10.1
If ΔABC≅ΔDEF, what else do you know?
Solution
From this congruence statement, we know three pairs of angles and three pairs of sides are congruent.
∠A≅∠D,∠B≅∠E,\angle C\cong \angle F\),
¯AB≅¯DE,¯BC≅¯EF,¯AC≅¯DF.
Example 4.10.2
IfΔKBP≅ΔMRS, what else do you know?
Solution
From this congruence statement, we know three pairs of angles and three pairs of sides are congruent.
∠K≅∠M,∠B≅∠R,∠P≅∠S,
¯KB≅¯MR,¯BP≅¯RS,¯KP≅¯MS.
Example 4.10.3
Write a congruence statement for the two triangles below.
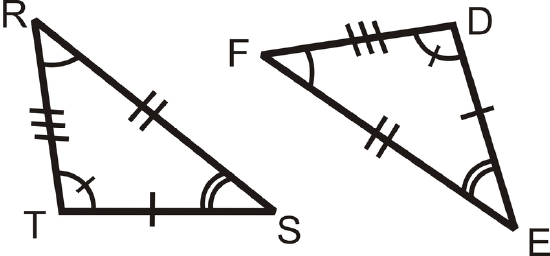
Solution
Line up the corresponding angles in the triangles:
∠R≅∠F,∠S≅∠Eand∠T≅∠D.
Therefore, one possible congruence statement is ΔRST≅∠FED
Example 4.10.4
If ΔCAT≅ΔDOG, what else do you know?
Solution
From this congruence statement, we know three pairs of angles and three pairs of sides are congruent.

Example 4.10.5
If ΔBUG≅ΔANT, what angle is congruent to ∠N?
Solution
Since the order of the letters in the congruence statement tells us which angles are congruent, ∠N≅∠U because they are each the second of the three letters.
Review
For questions 1-4, determine if the triangles are congruent using the definition of congruent triangles. If they are, write the congruence statement.
-
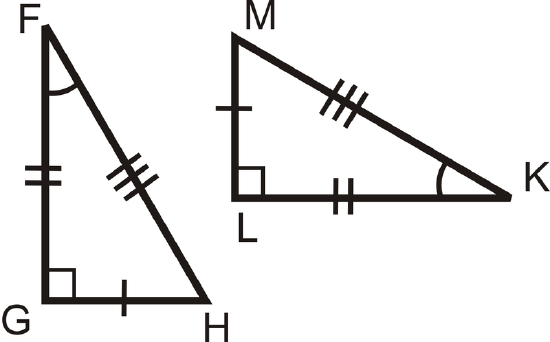
Figure 4.10.4 -
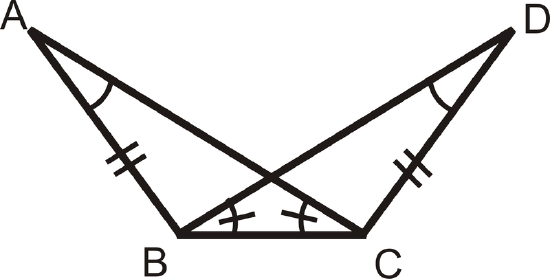
Figure 4.10.5 -
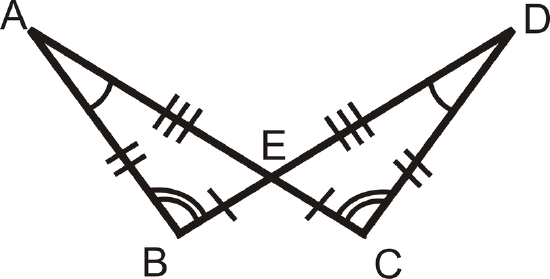
Figure 4.10.6 -
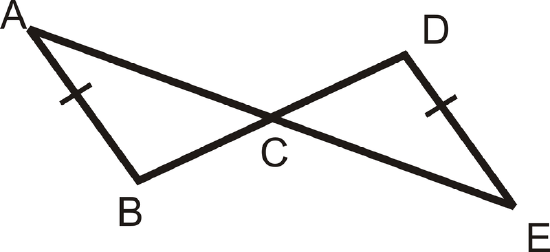
Figure 4.10.7 - Suppose the two triangles below are congruent. Write a congruence statement for these triangles.
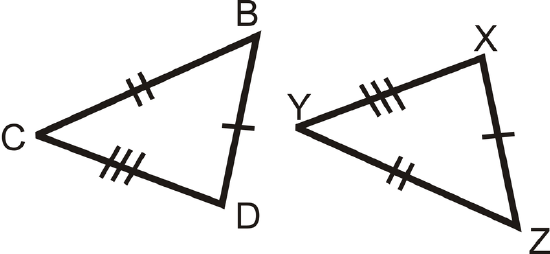
Figure 4.10.8 - Explain how we know that if the two triangles are congruent, then ∠B≅∠Z.
- If ΔTBS≅ΔFAM, what else do you know?
- If ΔPAM≅ΔSTE, what else do you know?
- If ΔINT≅ΔWEB, what else do you know?
- If ΔADG≅ΔBCE, what angle is congruent to ∠G?
Review (Answers)
To see the Review answers, open this PDF file and look for section 4.4.
Resources
Additional Resources
Video: Introduction to Congruent Triangles
Activities: Congruence Statements Discussion Questions
Study Aids: Triangle Congruence Study Guide
Practice: Congruence Statements
Real World: Congruent Statements