4.12: Congruent Triangles
( \newcommand{\kernel}{\mathrm{null}\,}\)
Verify congruency with SSS, SAS, RHS, and ASA
Applications of Congruent Triangles
Two triangles are congruent if and only if corresponding pairs of sides and corresponding pairs are congruent.
The following list summarizes the different criteria that can be used to show triangle congruence:
- AAS (Angle-Angle-Side): If two triangles have two pairs of congruent angles, and a non-common side of the angles in one triangle is congruent to the corresponding side in the other triangle, then the triangles are congruent.
- ASA (Angle-Side-Angle): If two triangles have two pairs of congruent angles and the common side of the angles (the side between the congruent angles) in one triangle is congruent to the corresponding side in the other triangle, then the triangles are congruent.
- SAS (Side-Angle-Side): If two triangles have two pairs of congruent sides and the included angle in one triangle is congruent to the included angle in the other triangle, then the triangles are congruent.
- SSS (Side-Side-Side): If two triangles have three pairs of congruent sides, then the triangles are congruent.
- Right triangles only: HL (Hypotenuse-Leg): If two right triangles have one pair of legs congruent and hypotenuses congruent, then the triangles are congruent.
If two triangles don't satisfy at least one of the criteria above, you cannot be confident that they are congruent.
Interactive Element
Recognizing Perpendicular Bisectors
In the triangle below, \overline{BC} is the perpendicular bisector of AD\overline{AB}. Therefore \overline{AC}\cong \overline{CD}. Also, m\angle ACB=90^{\circ}and m\angle DCB=90^{\circ}, so \angle ACB \cong \angle DCB. You also know that \overline{BC} is a side of both triangles, and is clearly congruent to itself (this is called the reflexive property).

The triangles are congruent by SAS. Note that even though these are right triangles, you would not use HL to show triangle congruence in this case since you are not given that the hypotenuses are congruent.
Measuring Angles
Using the information from the previous problem, if m∠A=50∘, what is m∠D?
m∠D=50∘
Since the triangles are congruent, all of their corresponding angles and sides must be congruent. \angle A\) and \angle D\) are corresponding angles, so ∠A≅∠D.
Congruent Triangles
Does one diagonal of a rectangle divide the rectangle into congruent triangles?
- Recall that a rectangle is a quadrilateral with four right angles.
- The opposite sides of a rectangle are congruent.

There is more than enough information to show that \(\Delta EFG\cong \Delta GHE)\.
- Method #1: The triangles have three pairs of congruent sides, so they are congruent by SSS.
- Method #2: The triangles have two pairs of congruent sides and congruent included angles, so they are congruent by SAS.
- Method #3: The triangles are right triangles with congruent hypotenuses and a pair of congruent legs, so they are congruent by HL.
Example 4.12.1
Max constructs a triangle using an online tool. He tells Alicia that his triangle has a 42^{\circ} angle, a side of length 12 and a side of length 8. With only this information, will Alicia be able to construct a triangle that must be congruent to Max's triangle?
Solution
If Max also told Alicia that the angle was in between the two sides, then she would be able to construct a triangle that must be congruent due to SAS. If the angle is not between the two sides, she cannot be confident that her triangle is congruent because SSA is not a criterion for triangle congruence. Because Max did not state where the angle was in relation to the sides, Alicia cannot create a triangle that must be congruent to Max's triangle.
Example 4.12.2
Are the following triangles congruent? Explain.

Solution
Notice that besides the one pair of congruent sides and the one pair of congruent angles, ¯AC≅¯CA.
ΔACB≅ΔCAD by SAS.
Example 4.12.3
Are the following triangles congruent? Explain.
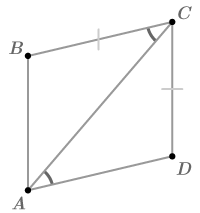
Solution
The congruent sides are not corresponding in the same way that the congruent angles are corresponding. The given information for ΔACB is SAS while the given information for ΔCAD is SSA. The triangles are not necessarily congruent.
Example 4.12.4
G is the midpoint of ¯EH. Are the following triangles congruent? Explain.

Solution
Because G\) is the midpoint of ¯EH, ¯EG≅¯GH. You also know that ∠EGF≅∠HGI because they are vertical angles. ΔEGF≅ΔHGI by ASA.
Review
1. List the five criteria for triangle congruence and draw a picture that demonstrates each.
2. Given two triangles, do you always need at least three pieces of information about each triangle in order to be able to state that the triangles are congruent?
For each pair of triangles, tell whether the given information is enough to show that the triangles are congruent. If the triangles are congruent, state the criterion that you used to determine the congruence and write a congruency statement.
Note that the images are not necessarily drawn to scale.
3.

4.

5.
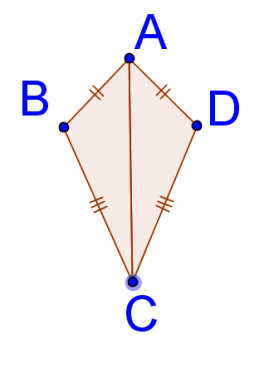
6.
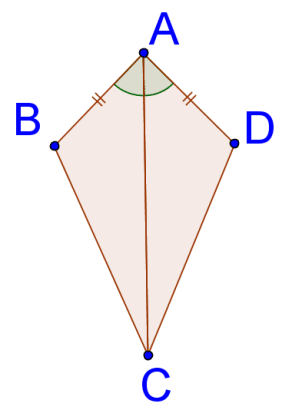
7.
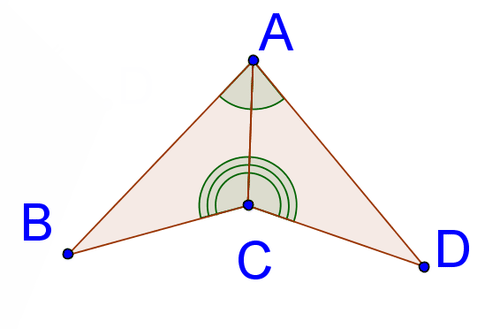
8.
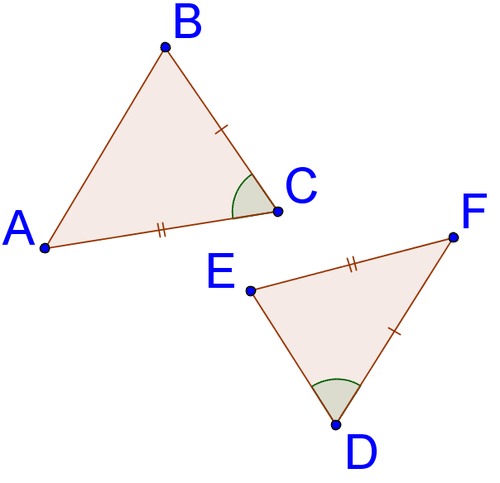
For 9-11, state whether the given information about a hidden triangle would be enough for you to construct a triangle that must be congruent to the hidden triangle. Explain your answer.
9. ΔABC with m∠A=72∘,AB=6cm,BC=8cm.
10. ΔABC with m∠A=90∘,AB=4cm,BC=5cm.
11. ΔABC with m∠A=72∘,AB=6cm,AC=8cm.
12. Recall that a square is a quadrilateral with four right angles and four congruent sides. Show and explain why a diagonal of a square divides the square into two congruent triangles.
13. Show and explain using a different criterion for triangle congruence why a diagonal of a square divides the square into two congruent triangles.
14. Recall that a kite is a quadrilateral with two pairs of adjacent, congruent sides. Will one of the diagonals of a kite divide the kite into two congruent triangles? Show and explain your answer.
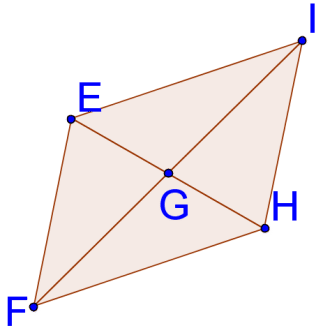
15. In the picture below, G is the midpoint of both ¯EH and ¯FI. Explain why ¯FH≅¯IE and ¯FE≅¯HI.
16. Explain why AAA is not a criterion for triangle congruence.
Review (Answers)
To see the Review answers, click here.
Additional Resources
Video: Congruent and Similar Triangles - KA
Practice: Congruent Triangles


